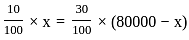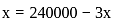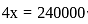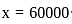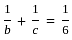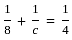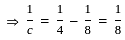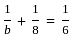RRB NTPC Mathematics Test - 3 - RRB NTPC/ASM/CA/TA MCQ
30 Questions MCQ Test RRB NTPC Mock Test Series 2025 - RRB NTPC Mathematics Test - 3
Two years ago, the population of a town was 66000. The population increased by 5% in the first year and decreased by 5% in the second year. Find the present population of the town.
A mixture contains milk and water in a ratio of 9 ∶ 5. When 6 litres of water is added to this mixture, the ratio of milk and water becomes 9 ∶ 7, Find the quantity of milk in the mixture
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
In a vessel, a mixture of milk and water is in ratio 8 : 7, while in another vessel mixture of milk and water is in ratio 7 : 9. In what ratio mixture of both the vessels should be mixed together so that in the resultant mixture ratio of water and milk becomes 9 : 8?
If the third proportion to 10 and x is 90 and the third proportion of 12 and y is 27. Find the value of (x + y).
The simple interest on a sum of money is 2/25 of the principal, and the number of years is equal to 2 times the rate percent per annum. Find the rate percent.
The following table presents data about the number of men, women and children and percentage (%) of overweight men, overweight women and overweight children in a city during the last six years from 2016 to 2021.
Year-wise Distribution of Population in a city
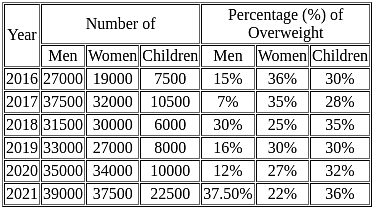
What was the total number of children who were not overweight in the year 2016 and 2017 together?
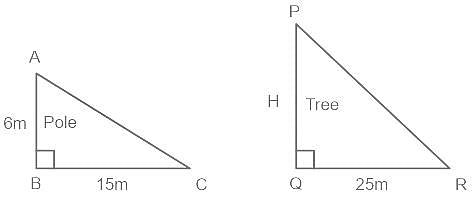
The shadow of a pole 6 mtr high is 15 mtr long and at the same time the shadow of a tree is 25 mtr long. What is the height of the tree?
The sum of radii of two spheres is 28 cm & their volumes are in ratio 27:64. Find the radii of the spheres.
In one hill station train there are three categories of seats. The seats are numbered from 1 to 50 in the first class, 51 to 150 in the second class and 151 to 300 in the third class. Every December, the seat occupancy was 30% in the first class, 50% in the second class and 100% in the third class. If the per seat charges are Rs. 2,000, Rs. 1,000 and Rs. 500 in first class, second class and third class respectively, then the income ratio per category in December month is:
The third proportional to a3 + b3 and a2 + ab + b2, when a = 2 and b = 3, is:
(correct to 2 decimal places)
Ram and Lal have a combined salary of Rs 80,000, Ram spends 90% of his salary and Lal spends 70% of his salary and at the end of the month both save equal amounts of money, find the salary of Lal.
In how much time would the simple interest on a principal amount be 0.125 times the principal amount at 10% per annum?
Which of the following options is the closest approximate value which will come in place of question mark(?) in the following equation?
104.96 + 120.96 + 103.16 - 12.89 × 2.04 + 124.93 ÷ 5.1 = ?
The average age of A and B is 30 years, the average age of B and C is 32 years and the average age of C and A is 34 years. The age of C is
Rate of evaporation of water from a mixture of milk and water in sunlight is 2.5L/hour. At 10:00 AM quantity of milk was 90 liters more than the water and at 4:00 PM same day, ratio of milk to water becomes 8 : 1, then milk will be what percent of total quantity of mixture at 8:00 PM on same day? (Consider sunlight till 8:00 PM)
A class has to be designed with seating groups containing either 4, 6 or 10 seats in each of the group. What should be the minimum number of seats that should the class contain?
State whether the statements given in questions are true (T) or false (F):
If N ÷ 5 leaves remainder 3 and N ÷ 2 leaves remainder 0, then N ÷ 10 leaves remainder 4.
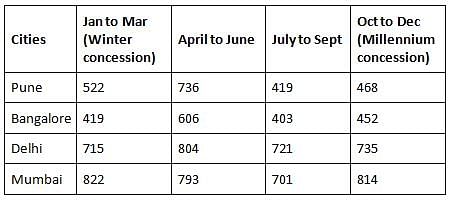
Given below is the data of the sale of Washing Machine of a company in four cities in a year. The record is based on the quarterly sale.
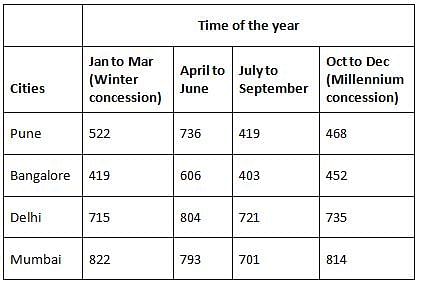
The number of Washing Machine sold during the quarterly period of winter concession in all cities were how many more or less than those of the millennium concession period?
Study the given pie chart and answer the question that follows.
The pie-chart shows the number of tourists visiting from different states. The total tourist traffic is 20 lakh.
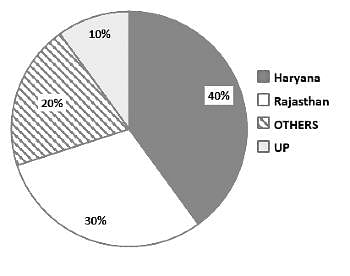
Find the difference between the number of tourists visiting from Haryana and Rajasthan.
Which of the following are divisible by 2, 3 and 11?
A. 8448
B. 9812
C. 9126
D. 9636
Choose the correct option: