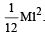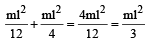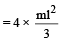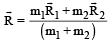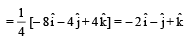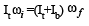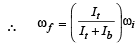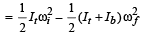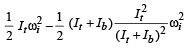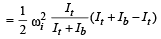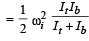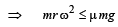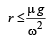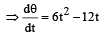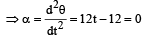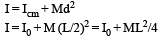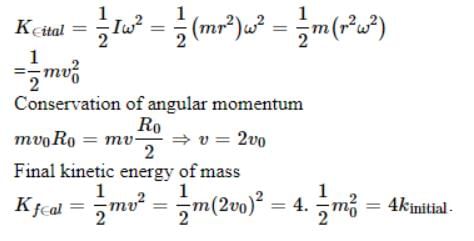31 Year NEET Previous Year Questions: System of Particles & Rotational Motion - 2 - NEET MCQ
30 Questions MCQ Test Physics 31 Years NEET Chapterwise Solved Papers - 31 Year NEET Previous Year Questions: System of Particles & Rotational Motion - 2
A solid sphere of radius R is placed on a smooth horizontal surface. A horizontal force F is applied at height h from the lowest point. For the maximum acceleration of the centre of mass, [2002]
A boy suddenly comes and sits on a circular rotating table. What will remain conserved? [2002]
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
A ball rolls without slipping. The radius of gyration of the ball about an axis passing through its centre of mass is K. If radius of the ball be R, then the fraction of total energy associated with its rotational energy will be [2003
A solid cylinder of mass M and radius R rolls without slipping down an inclined plane of length L and height h. What is the speed of its centre of mass when the cylinder reaches its bottom ? [2003]
A thin circular ring of mass M and radius r is rotating about its axis with a constant angular velocity ω. Four objects each of mass m, are kept gently to the opposite ends of two perpendicular diameters of the ring. The angular velocity of the ring will be [2003]
A wheel having moment of inertia 2 kg-m2 about its vertical axis, rotates at the rate of 60 rpm about this axis. The torque which can stop the wheel’s rotation in one minute would be [2004]
Consider a system of two particles having masses m1 and m2 . If the particle of mass m1 is pushed towards the centre of mass of particles through a distance d, by what distance would the particle of mass m2 move so as to keep the centre of mass of particles at the original position? [2004]
The ratio of the radii of gyration of a circular disc about a tangential axis in the plane of the disc and of a circular ring of the same radius about a tangential axis in the plane of the ring is [2004]
A r ound disc of moment of in ertia I2 about its axis perpendicular to its plane and passing through its centre is placed over another disc of moment of inertia I1 rotating with an angular velocity ω about the same axis. The final angular velocity of the combin ation of discs is [2004]
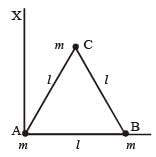
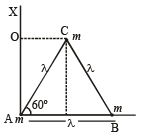
Three particles, each of mass m gram, are situated at the vertices of an equilateral triangle ABC of side l cm (as shown in the figure). The moment of inertia of the system about a line AX perpendicular to AB and in the plane of ABC, in gram-cm2 units will be [2004]
The moment of inertia of a uniform circular disc of radius R and mass M about an axis passing from the edge of the disc and normal to the disc is : [2 00 5]
Two bodies have th eir momen ts of in er tia I and 2I respectively about their axis of rotation. If their kinetic energies of rotation are equal, their angular momenta will be in the ratio[2005]
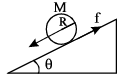
A drum of radius R and mass M, rolls down without slipping along an inclined plane of angle θ. The frictional force [2005]
The moment of inertia of a uniform circular disc of radius R and mass M about an axis touching the disc at its diameter and normal to the disc is
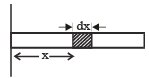
A tube of length L is filled completely with an incompressible liquid of mass M and closed at both the ends. The tube is then rotated in a horizontal plane about one of its ends with a uniform angular velocity ω. The force exerted by the liquid at the other end is [2006]
A wheel has angular acceleration of 3.0 rad/sec2 and an initial angular speed of 2.00 rad/sec. In a time of 2 sec it has rotated through an angle (in radian) of [2007]
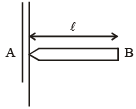
A uniform rod AB of length ℓ, and mass m is free to rotate about point A. The rod is released from rest in the horizontal position. Given that the moment of inertia of the rod about A is 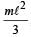 , the initial angular acceleration of the rod will be
, the initial angular acceleration of the rod will be
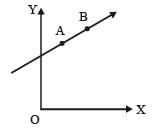
A particle of mass m moves in the XY plane with a velocity v along the straight line AB. If the angular momentum of the particle with respect to origin O is LA when it is at A and LB when it is at B, then
The ratio of the radii or gyration of a circular disc to that of a circular ring, each of same mass and radius, around their respective axes is:
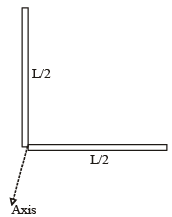
A thin rod of length L and mass M is bent at its midpoint into two halves so that the angle between them is 90°. The moment of inertia of the bent rod about an axis passing through the bending point and perpendicular to the plane defined by the two halves of the rod is: [2008]
A t hin circular ring of mass M an d radius R is rotating in a horizontal plane about an axis vertical to its plane with a constant angular velocity ω. If two objects each of mass m be attached gently to the opposite ends of a diameter of the ring, the ring will then rotate with an angular velocity: [2009]
If 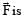 the force acting on a particle having position vector
the force acting on a particle having position vector 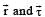 be the tor que of this force about the origin, then: [2009]
be the tor que of this force about the origin, then: [2009]
Four identical th in rods each of mass M and length l, form a square frame. Moment of inertia of this frame about an axis through the centre of the square and perpendicular to its plane is : [2009]
Two bodies of mass 1 kg and 3 kg have position vectors 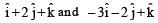 ˆ respectively. The centre of mass of this system has a position vector: [2009]
ˆ respectively. The centre of mass of this system has a position vector: [2009]
A circular disk of moment of inertia It is rotating in a horizontal plane, its symmetry axis, with a constant angular speed ωi . Another disk of moment of inertia Ib is dropped coaxially onto the rotating disk. Initially the second disk has zero angular speed. Eventually both the disks rotate with a constant angular speed ωf . The energy lost by the initially rotating disk to friction is:
[2010]
Two particles which are initially at rest, move towards each other under the action of their internal attraction. If their speeds are v and 2v at any instant, then the speed of centre of mass of the system will be: [2010]
A gramophone recor d is revolving with an angular velocity ω. A coin is placed at a distance r from the centre of the record. The static coefficient of friction is ω. The coin will revolve with the record if: [2010]
The instantaneous angular position of a point on a rotating wheel is given by the equation θ(t) = 2t3 – 6t2. The torque on the wheel becomes zero at[2011]
The moment of inertia of a thin uniform rod of mass M and length L about an axis passing through its midpoint and perpendicular to its length is I0. Its moment of inertia about an axis passing through one of its ends and perpendicular to its length is [2011]
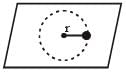
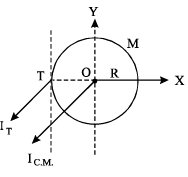
A small mass attached to a string rotates on frictionless table top as shown. If the tension in the string is increased by pulling the string causing the radius of the circular motion to decrease by a factor of 2, the kinetic energy of the mass will [2011M]
|
28 docs|47 tests
|
|
28 docs|47 tests
|


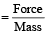
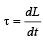
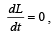 , L = constant.
, L = constant.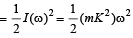
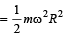
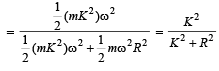
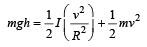
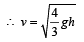
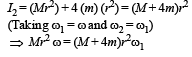
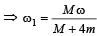
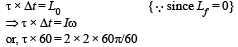
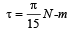
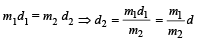
 Circular disc [1]
Circular disc [1]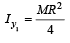
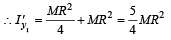
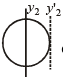 Circular ring
Circular ring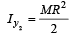
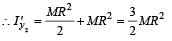
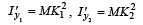
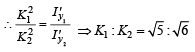
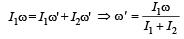
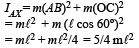
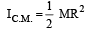
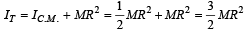

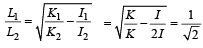
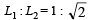
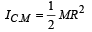
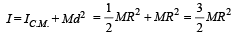
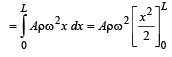
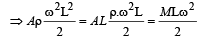
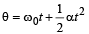
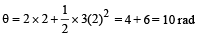
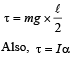
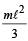 and α is the angular acceleration
and α is the angular acceleration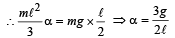
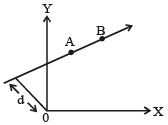
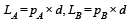
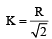
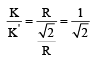
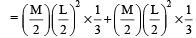
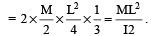
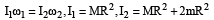
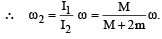
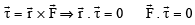
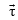 is perpendicular to the plane of
is perpendicular to the plane of 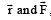 , h ence the dot product of
, h ence the dot product of