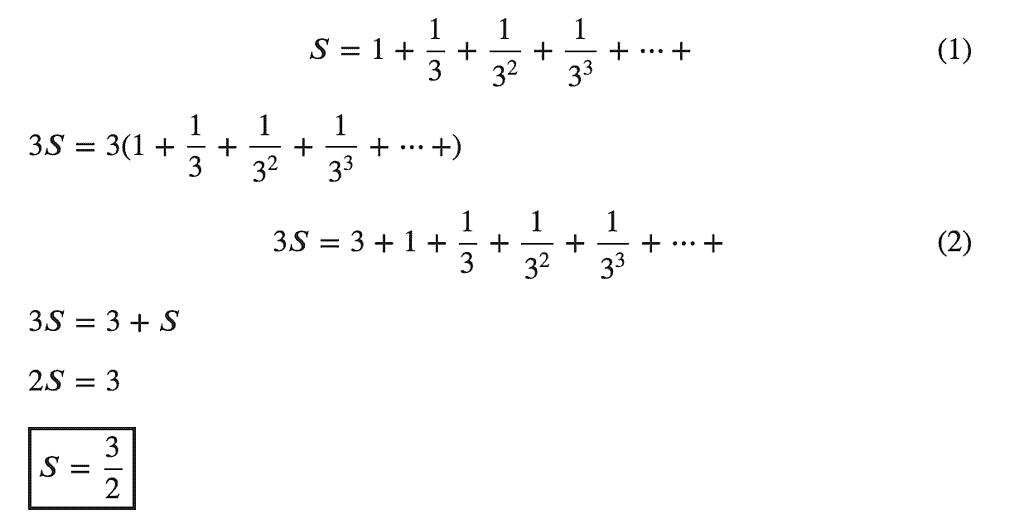All Courses
Get the App Signup / Login
Sign in Open App
CA Foundation Exam  >  CA Foundation Tests  >  Quantitative Aptitude for CA Foundation  >  Test: Arithmetic And Geometric Progressions - 2 - CA Foundation MCQ

Test: Arithmetic And Geometric Progressions - 2 - CA Foundation MCQ


Test Description

30 Questions MCQ Test Quantitative Aptitude for CA Foundation - Test: Arithmetic And Geometric Progressions - 2

Test: Arithmetic And Geometric Progressions - 2 for CA Foundation 2024 is part of Quantitative Aptitude for CA Foundation preparation. The Test: Arithmetic And Geometric Progressions - 2 questions and answers have been prepared according to the CA Foundation exam syllabus.The Test: Arithmetic And Geometric Progressions - 2 MCQs are made for CA Foundation 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Arithmetic And Geometric Progressions - 2 below.
Solutions of Test: Arithmetic And Geometric Progressions - 2 questions in English are available as part of our Quantitative Aptitude for CA Foundation for CA Foundation & Test: Arithmetic And Geometric Progressions - 2 solutions in Hindi for Quantitative Aptitude for CA Foundation course. Download more important topics, notes, lectures and mock test series for CA Foundation Exam by signing up for free. Attempt Test: Arithmetic And Geometric Progressions - 2 | 40 questions in 40 minutes | Mock test for CA Foundation preparation | Free important questions MCQ to study Quantitative Aptitude for CA Foundation for CA Foundation Exam | Download free PDF with solutions
Test: Arithmetic And Geometric Progressions - 2 - Question 1
Save

​Sum of n terms of the series 0.1 + 0.11 + 0.111 + … is

Test: Arithmetic And Geometric Progressions - 2 - Question 2
Save

The sum of the first 20 terms of a G. P is 244 times the sum of its first 10 terms. The common ratio is

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Arithmetic And Geometric Progressions - 2 - Question 3
Save

Sum of the series 1 + 3 + 9 + 27 +….is 364. The number of terms is

Test: Arithmetic And Geometric Progressions - 2 - Question 4
Save

The product of 3 numbers in G P is 729 and the sum of squares is 819. The numbers are
Test: Arithmetic And Geometric Progressions - 2 - Question 5
Save

The sum of the series 1 + 2 + 4 + 8 + .. to n term
Detailed Solution for Test: Arithmetic And Geometric Progressions - 2 - Question 5

Important Notes on GP Sum:The sum of GP (of n terms) is: Sn = a(rn - 1) / (r - 1) 
a = 1 , r = 2
Sn = a(rn - 1) / (r - 1) 
Sn = (1) x (2n - 1) / (2 - 1)
Sn = 2n - 1

Test: Arithmetic And Geometric Progressions - 2 - Question 6
Save

The sum of the infinite GP 14 – 2 + 2/7 – 2/49 + … is
Test: Arithmetic And Geometric Progressions - 2 - Question 7
Save

The sum of the infinite G. P. 1 - 1/3 + 1/9 - 1/27 +... is
Test: Arithmetic And Geometric Progressions - 2 - Question 8
Save

The number of terms to be taken so that 1 + 2 + 4 + 8 + will be 8191 is
Detailed Solution for Test: Arithmetic And Geometric Progressions - 2 - Question 8

If you're talking about geometric series:

a = 1

r = 2/1 = 4/2 = 8/4 = 2

S(n) = a(1 - r^n) / (1 - r)

a(1 - r^n) / (1 - r) = 8191

1(1 - 2^n) / (1 - 2) = 8191

(1 - 2^n) / (-1) = 8191

1 - 2^n = 8191 * (-1)

1 - 2^n = -8191

-2^n = -8191 - 1

-2^n = -8192

2^n = 8192

n = log (base 2) of 8192

n = 13

Test: Arithmetic And Geometric Progressions - 2 - Question 9
Save

Four geometric means between 4 and 972 are
Detailed Solution for Test: Arithmetic And Geometric Progressions - 2 - Question 9

Let t1 = 4, t2, t3, t4, t5, t6 = 972 be the sequence.
Then; t6 = 972 ⇒ ar5 = 972 ⇒ 4r5 = 972
⇒ r5 = 243 = 35 
⇒ r = 3
Hence the arithmetic means between 4 and 972 are 12, 36, 108, 324.

Test: Arithmetic And Geometric Progressions - 2 - Question 10
Save

Three numbers are in AP and their sum is 21. If 1, 5, 15 are added to them respectively, they form a G. P. The numbers are
Test: Arithmetic And Geometric Progressions - 2 - Question 11
Save

The sum of 1 + 1/3 + 1/32 + 1/33 + … + 1/3 n –1 is
Detailed Solution for Test: Arithmetic And Geometric Progressions - 2 - Question 11

Test: Arithmetic And Geometric Progressions - 2 - Question 12
Save

The sum of the infinite series 1 + 2/3 + 4/9 + .. is
*Multiple options can be correct
Test: Arithmetic And Geometric Progressions - 2 - Question 13
Save

The sum of the first two terms of a G.P. is 5/3 and the sum to infinity of the series is 3. The common ratio is
Test: Arithmetic And Geometric Progressions - 2 - Question 14
Save

If p, q and r are in A.P. and x, y, z are in G.P. then xq–r. y r–p. zp–q is equal to
*Multiple options can be correct
Test: Arithmetic And Geometric Progressions - 2 - Question 15
Save

The sum of three numbers in G.P. is 70. If the two extremes by multiplied each by 4 and the mean by 5, the products are in AP. The numbers are
*Multiple options can be correct
Test: Arithmetic And Geometric Progressions - 2 - Question 16
Save

The sum of 3 numbers in A.P. is 15. If 1, 4 and 19 be added to them respectively, the results are is G. P. The numbers are
Test: Arithmetic And Geometric Progressions - 2 - Question 17
Save

Given x, y, z are in G.P. and xp = yq = zσ, then 1/p , 1/q, 1/σ are in
Test: Arithmetic And Geometric Progressions - 2 - Question 18
Save

If the terms 2x, (x+10) and (3x+2) be in A.P., the value of x is
Detailed Solution for Test: Arithmetic And Geometric Progressions - 2 - Question 18

2b=a+c
2x+3x+2=2(x+10)
5x+2=2x+20
3x=18
x=6

Test: Arithmetic And Geometric Progressions - 2 - Question 19
Save

If A be the A.M. of two positive unequal quantities x and y and G be their G. M, then
Test: Arithmetic And Geometric Progressions - 2 - Question 20
Save

The A.M. of two positive numbers is 40 and their G. M. is 24. The numbers are
Test: Arithmetic And Geometric Progressions - 2 - Question 21
Save

Three numbers are in A.P. and their sum is 15. If 8, 6, 4 be added to them respectively, the numbers are in G.P. The numbers are
Test: Arithmetic And Geometric Progressions - 2 - Question 22
Save

The sum of four numbers in G. P. is 60 and the A.M. of the 1st and the last is 18. The numbers are
Test: Arithmetic And Geometric Progressions - 2 - Question 23
Save

A sum of Rs. 6240 is paid off in 30 instalments such that each instalment is Rs. 10 more than the proceeding installment. The value of the 1st instalment is
Test: Arithmetic And Geometric Progressions - 2 - Question 24
Save

The sum of 1.03 + (1.03)2 + (1.03)3 + …. to n terms is
Test: Arithmetic And Geometric Progressions - 2 - Question 25
Save

If x, y, z are in A.P. and x, y, (z + 1) are in G.P. then
Test: Arithmetic And Geometric Progressions - 2 - Question 26
Save

The numbers x, 8, y  are in G.P. and the numbers x, y, –8 are in A.P. The value of x and y are
Test: Arithmetic And Geometric Progressions - 2 - Question 27
Save

The nth term of the series 16, 8, 4,…. Is 1/217. The value of n is
Test: Arithmetic And Geometric Progressions - 2 - Question 28
Save

The sum of n terms of a G.P. whose first terms 1 and the common ratio is 1/2 , is equal to 
Test: Arithmetic And Geometric Progressions - 2 - Question 29
Save

t4 of a G.P. in x, t10 = y and t16 = z. Then
Test: Arithmetic And Geometric Progressions - 2 - Question 30
Save

If x, y, z are in G.P., then
View more questions
148 videos|174 docs|99 tests
Join Course
Information about Test: Arithmetic And Geometric Progressions - 2 Page
In this test you can find the Exam questions for Test: Arithmetic And Geometric Progressions - 2 solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Arithmetic And Geometric Progressions - 2, EduRev gives you an ample number of Online tests for practice

Top Courses for CA Foundation

Download as PDF

Top Courses for CA Foundation

Important Questions for Arithmetic And Geometric Progressions - 2

Find all the important questions for Arithmetic And Geometric Progressions - 2 at EduRev.Get fully prepared for Arithmetic And Geometric Progressions - 2 with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Arithmetic And Geometric Progressions - 2 syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Arithmetic And Geometric Progressions - 2 resources.

Arithmetic And Geometric Progressions - 2 MCQs with Answers

Prepare for the Arithmetic And Geometric Progressions - 2 within the CA Foundation exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Arithmetic And Geometric Progressions - 2 Quantitative Aptitude for CA Foundation

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Arithmetic And Geometric Progressions - 2 with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Follow Us
Signup to see your scores go up
within 7 days!
Takes less than 10 seconds to signup