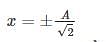Physics Exam > Physics Tests > Topic wise Tests for IIT JAM Physics > Simple Harmonic Motion MSQ - Physics MCQ
Simple Harmonic Motion MSQ - Physics MCQ
Test Description
10 Questions MCQ Test Topic wise Tests for IIT JAM Physics - Simple Harmonic Motion MSQ
Simple Harmonic Motion MSQ for Physics 2024 is part of Topic wise Tests for IIT JAM Physics preparation. The Simple Harmonic Motion MSQ questions and answers have been
prepared according to the Physics exam syllabus.The Simple Harmonic Motion MSQ MCQs are made for Physics 2024 Exam. Find important
definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Simple Harmonic Motion MSQ below.
Solutions of Simple Harmonic Motion MSQ questions in English are available as part of our Topic wise Tests for IIT JAM Physics for Physics & Simple Harmonic Motion MSQ solutions in
Hindi for Topic wise Tests for IIT JAM Physics course. Download more important topics, notes, lectures and mock
test series for Physics Exam by signing up for free. Attempt Simple Harmonic Motion MSQ | 10 questions in 45 minutes | Mock test for Physics preparation | Free important questions MCQ to study Topic wise Tests for IIT JAM Physics for Physics Exam | Download free PDF with solutions
*Multiple options can be correct
Simple Harmonic Motion MSQ - Question 1
The force which does not describe a simple harmonic motion is
Detailed Solution for Simple Harmonic Motion MSQ - Question 1
*Multiple options can be correct
Simple Harmonic Motion MSQ - Question 2
Choose the correct statements for the case of a one dimensional simple harmonic motion.
Detailed Solution for Simple Harmonic Motion MSQ - Question 2
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
*Multiple options can be correct
Detailed Solution for Simple Harmonic Motion MSQ - Question 3
*Multiple options can be correct
Simple Harmonic Motion MSQ - Question 4
The physical condition for simple harmonic motion is/are
Detailed Solution for Simple Harmonic Motion MSQ - Question 4
*Multiple options can be correct
Simple Harmonic Motion MSQ - Question 5
For a particular case of simple harmonic motion the total energy
Detailed Solution for Simple Harmonic Motion MSQ - Question 5
Simple Harmonic Motion MSQ - Question 6
A particle is executing simple harmonic motion (SHM) of amplitude A, along the x-axis, about x = 0. When its potential energy (PE) equals kinetic energy (KE), the position of the particle will be
Detailed Solution for Simple Harmonic Motion MSQ - Question 6
*Multiple options can be correct
Detailed Solution for Simple Harmonic Motion MSQ - Question 7
*Multiple options can be correct
Simple Harmonic Motion MSQ - Question 8
Velocity of a particle undergoing simple harmonic motion is
Detailed Solution for Simple Harmonic Motion MSQ - Question 8
Detailed Solution for Simple Harmonic Motion MSQ - Question 9
Simple Harmonic Motion MSQ - Question 10
When a rigid body is suspended vertically and it oscillates with a small amplitude under the action of the force of gravity, the body is known as
Detailed Solution for Simple Harmonic Motion MSQ - Question 10
Information about Simple Harmonic Motion MSQ Page
In this test you can find the Exam questions for Simple Harmonic Motion MSQ solved & explained in the simplest way possible.
Besides giving Questions and answers for Simple Harmonic Motion MSQ, EduRev gives you an ample number of Online tests for practice