Test: Relations and Functions- Case Based Type Questions- 2 - Commerce MCQ
15 Questions MCQ Test - Test: Relations and Functions- Case Based Type Questions- 2
Direction: Read the following text and answer the following questions on the basis of the same:
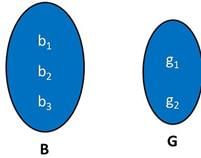
An organization conducted bike race under 2 different categories–boys and girls. Totally there were 250 participants. Among all of them finally three from Category 1 and two from Category 2 were selected for the final race. Ravi forms two sets B and G with these participants for his college project.
Let B = {b1, b2, b3} G = {g1, g2} where B represents the set of boys selected and G the set of girls who were selected for the final race.

Ravi decides to explore these sets for various types of relations and functions
Ravi wishes to form all the relations possible from B to G. How many such relations are possible?

Direction: Read the following text and answer the following questions on the basis of the same:
An organization conducted bike race under 2 different categories–boys and girls. Totally there were 250 participants. Among all of them finally three from Category 1 and two from Category 2 were selected for the final race. Ravi forms two sets B and G with these participants for his college project.
Let B = {b1, b2, b3} G = {g1, g2} where B represents the set of boys selected and G the set of girls who were selected for the final race.

Ravi decides to explore these sets for various types of relations and functions
Let R : B → G be defined by R = {(b1, g1), (b2, g2), (b3, g1)}, then R is__________

Direction: Read the following text and answer the following questions on the basis of the same:
Students of Grade 9, planned to plant saplings along straight lines, parallel to each other to one side of the playground ensuring that they had enough play area. Let us assume that they planted one of the rows of the saplings along the line y = x − 4. Let L be the set of all lines which are parallel on the ground and R be a relation on L.

Let relation R be defined by R = {(L1, L2) : L1 || L2 where L1, L2 ∈ L} then R is______ relation

Direction: Read the following text and answer the following questions on the basis of the same:
Students of Grade 9, planned to plant saplings along straight lines, parallel to each other to one side of the playground ensuring that they had enough play area. Let us assume that they planted one of the rows of the saplings along the line y = x − 4. Let L be the set of all lines which are parallel on the ground and R be a relation on L.

Let f : R → R be defined by f(x) = x − 4. Then the range of f(x) is _______
Direction: Read the following text and answer the following questions n the basis of the same:
Raji visited the Exhibition along with her family. The Exhibition had a huge swing, which attracted many children. Raji found that the swing traced the path of a Parabola as given by y = x2.

Let f : R → R be defined by f(x) = x2 is_________
Direction: Read the following text and answer the following questions n the basis of the same:
Raji visited the Exhibition along with her family. The Exhibition had a huge swing, which attracted many children. Raji found that the swing traced the path of a Parabola as given by y = x2.

Let f : {1, 2, 3, ….} → {1, 4, 9, ….} be defined by f(x) = x2 is _________
Direction: Read the following text and answer the following questions n the basis of the same:
Raji visited the Exhibition along with her family. The Exhibition had a huge swing, which attracted many children. Raji found that the swing traced the path of a Parabola as given by y = x2.

The function f : Z → Z defined by f(x) = x2 is ______
Direction: Read the following text and answer the following questions on the basis of the same:
An organization conducted bike race under 2 different categories–boys and girls. Totally there were 250 participants. Among all of them finally three from Category 1 and two from Category 2 were selected for the final race. Ravi forms two sets B and G with these participants for his college project.
Let B = {b1, b2, b3} G = {g1, g2} where B represents the set of boys selected and G the set of girls who were selected for the final race.

Ravi decides to explore these sets for various types of relations and functions
Ravi wants to know among those relations, how many functions can be formed from B to G?
Direction: Read the following text and answer the following questions on the basis of the same:
Students of Grade 9, planned to plant saplings along straight lines, parallel to each other to one side of the playground ensuring that they had enough play area. Let us assume that they planted one of the rows of the saplings along the line y = x − 4. Let L be the set of all lines which are parallel on the ground and R be a relation on L.

The function f : R → R defined by f(x) = x − 4 is______
Direction: Read the following text and answer the following questions on the basis of the same:
An organization conducted bike race under 2 different categories–boys and girls. Totally there were 250 participants. Among all of them finally three from Category 1 and two from Category 2 were selected for the final race. Ravi forms two sets B and G with these participants for his college project.
Let B = {b1, b2, b3} G = {g1, g2} where B represents the set of boys selected and G the set of girls who were selected for the final race.

Ravi decides to explore these sets for various types of relations and functions
Let R : B → B be defined by R = {(x, y) : x and y are students of same sex}, Then this relation R is_______
Direction: Read the following text and answer the following questions on the basis of the same:
Students of Grade 9, planned to plant saplings along straight lines, parallel to each other to one side of the playground ensuring that they had enough play area. Let us assume that they planted one of the rows of the saplings along the line y = x − 4. Let L be the set of all lines which are parallel on the ground and R be a relation on L.

Let R = {(L1, L2) : L1 ⊥ L2 where L1, L2 ∈ L} which of the following is true?
Direction: Read the following text and answer the following questions n the basis of the same:
Raji visited the Exhibition along with her family. The Exhibition had a huge swing, which attracted many children. Raji found that the swing traced the path of a Parabola as given by y = x2.

Let : N → R be defined by f(x) = x2. Range of the function among the following is _______
Direction: Read the following text and answer the following questions on the basis of the same:
An organization conducted bike race under 2 different categories–boys and girls. Totally there were 250 participants. Among all of them finally three from Category 1 and two from Category 2 were selected for the final race. Ravi forms two sets B and G with these participants for his college project.
Let B = {b1, b2, b3} G = {g1, g2} where B represents the set of boys selected and G the set of girls who were selected for the final race.

Ravi decides to explore these sets for various types of relations and functions
Ravi wants to find the number of injective functions from B to G. How many numbers of injective functions are possible?
Direction: Read the following text and answer the following questions on the basis of the same:
Students of Grade 9, planned to plant saplings along straight lines, parallel to each other to one side of the playground ensuring that they had enough play area. Let us assume that they planted one of the rows of the saplings along the line y = x − 4. Let L be the set of all lines which are parallel on the ground and R be a relation on L.

Let R = {(L1, L2) : L1 || L2 and L1 : y = x – 4} then which of the following can be taken as L2?
Direction: Read the following text and answer the following questions n the basis of the same:
Raji visited the Exhibition along with her family. The Exhibition had a huge swing, which attracted many children. Raji found that the swing traced the path of a Parabola as given by y = x2.

Let f : N → N be defined by f(x) = x2 is ________