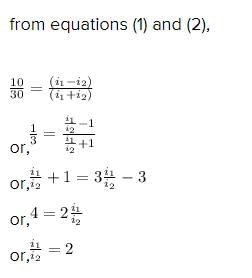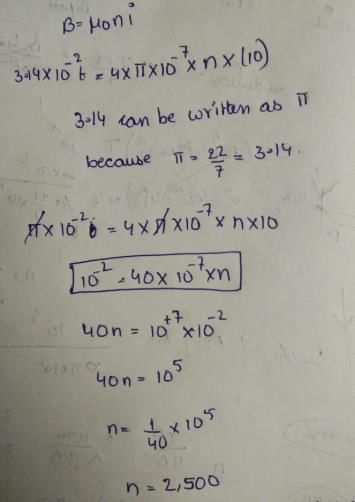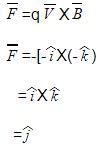Test: Magnetism & Matter - 2 - JEE MCQ
30 Questions MCQ Test Physics for JEE Main & Advanced - Test: Magnetism & Matter - 2
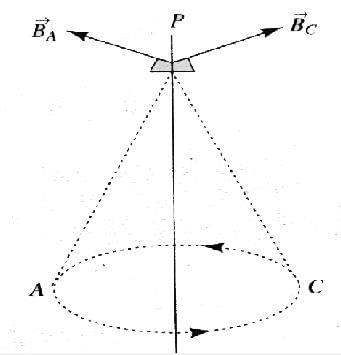
A point charge is moving in a circle with constant speed. Consider the magnetic field produced by the charge at a fixed point P (not centre of the circle) on the axis of the circle.
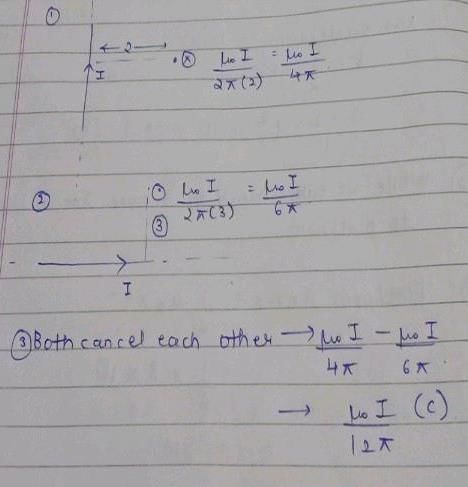
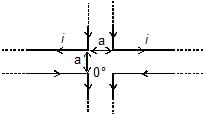
Four infinitely long `L' shaped wires, each carrying a current i have been arranged as shown in the figure. Obtain the magnetic field intensity at the point `O' equidistant from all the four corners.
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
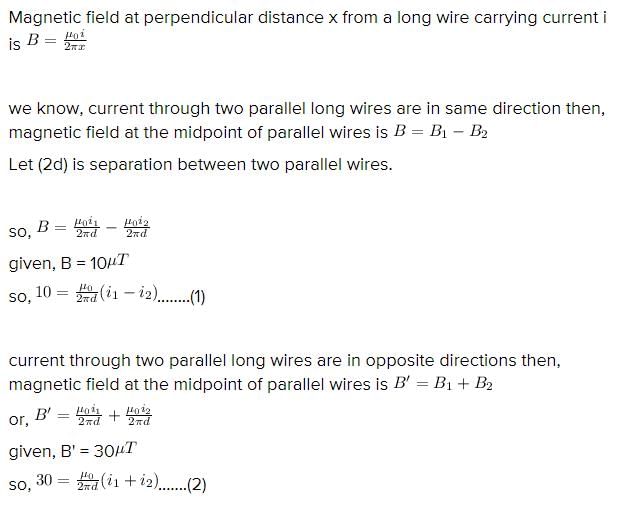
Two parallel, long wires carry currents i1 and i2 with i1 > i2. When the current are in the same direction, the magnetic field at a point midway between the wire is 10 mT. If the direction of i2 is reversed, the field becomes 30mT. The ratio i1/i2 is
Two mutually perpendicular insulated long conducting wires carrying equal currents I, intersect at origin. Then the resultant magnetic induction at point P (2m, 3m) will be -
Two parallel straight long conducting wires, which are placed at a distance r from each other, are carrying equal currents i in opposite directions. The value of magnetic induction at a point situated at a point situated x from one wire in between the wires will be
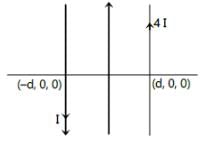
Two very long straight parallel wires, parallel to y-axis, carry currents 4I and I, along +y direction and _ y direction respectively. The wires are passes through the x-axis at the points (d, 0, 0) and (-d, 0, 0) respectively. The graph of magnetic field z-component as one moves along the x-axis from x = _ d to x = +d, is best given by
If the ratio of magnetic fields at two point in a definite direction due to a long current carrying wire is 3/4, then the ratio of the distances of these points from the wire will be :
The points A and B are situated perpendicular to the axis of 2 cm long bar magnet at large distances x and 3 x from the centre on opposite sides. The ratio of magnetic fields at A and B will be approximately equal to
A battery is connected between two points A and B the circumference of a uniform conducting ring of radius r and resistance R. One o the arcs AB of the ring subtends an angle q at the centre. The value of the magnetic induction at the centre due to the current in the ring is
Two circular coils of wire each having a radius of 4 cm and 10 turns have a common axis and are 6 cm apart. If a current of 1 A passess through each coil in the opposite direction find the magnetic induction. At a point on the axis, midway between them.
A direct current is passing through a wire. It is bent to form a coil of one turn. Now it is further bent to form a coil of two turns but at smaller radius. The ratio of the magnetic induction at the centre of this coil and at the centre of the coil of two turn is
If a copper rod carries a direct current, the magnetic field associated with the current will be [CPMT 1984]
Two wire loops PQRSP formed by joining two semicircular wires of radii R1 and R2 carries a current I as shown in (fig.) The magnitude of the magnetic induction at the centre C is ........
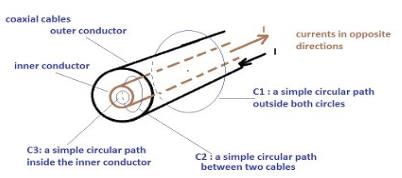
In a coaxial, straight cable, the central conductor and the outer conductor carry equal currents in opposite directions. The magnetic field is zero.
The magnetic field B inside a long solenoid, carrying a current of 10 A, is 3.14 × 10-2 T. Find the number of turns per unit length of the solenoid.
A rod AB moves with a uniform velocity v in a uniform magnetic field as shown in figure.
A charged particle is accelerated through a potential difference of 12 kV and acquires a speed of 1.0 × 106 m/s. It is then injected perpendicularly into a magnetic field of strength 0.2 T. Find the radius of the circle described by it.
A neutron, a proton, an electron and an a-particle enters a uniform magnetic field with equal velocities. The field is directed along the inward normal to the plane of the paper. Which of these tracks followed are by a - particle.
A proton of mass 1.67 × 10-27 kg and charge 1.6 × 10-19C is projected with a speed of 2 × 106 m/s at an angle of 60° to the x-axis. If a uniform magnetic field of 0.104 T is applied along the y-axis, the path of te proton is
Three ions H+, He+ and O+2 having same kinetic energy pass through a region in which there is a uniform magnetic field perpendicular to their velocity, then
Electrons moving with different speeds enter a uniform magnetic field in a direction perpendicular to the field. They will move along circular paths.
In the previous question, time periods of rotation will be
A particle having charge of 1 C, mass 1 kg and speed 1 m/s enters a uniform magnetic field, having magnetic induction of 1 T, at an angle q = 30° between velocity vector and magnetic induction. The pitch of its helical path is (in meters)
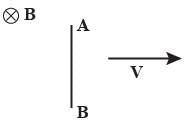
The direction of magnetic force on the electron as shown in the diagram is along
A particle moves in a region having a uniform magnetic field and a parallel, uniform electric field. at some instant, the velocity of the particle is perpendicular to the field direction. The path of the particle will be
An electron is moving along positive x-axis. A uniform electric field exists towards negative y-axis. What should be the direction of magnetic field of suitable magnitude so that net force of electron is zero.
A charged particle is released from rest in a region of uniform electric and magnetic fields, which are parallel to each other. The locus of the particle will be
|
289 videos|635 docs|179 tests
|
|
289 videos|635 docs|179 tests
|