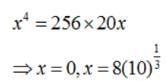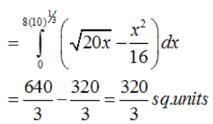Test: Application of Integrals- 5 - JEE MCQ
25 Questions MCQ Test Mathematics (Maths) Class 12 - Test: Application of Integrals- 5
The area bounded by the curve y = 4x - x2 and the x- axis is equal to
The area of the smaller segment cut off from the circle x2+y2 = 9by x = 1 is
For which of the following values of m , is the area of the region bounded by the curve y = x - x2 and the line y = mx equal to 9/2 ?
The area of the region bounded by the parabola (y−2)2 = x−1,the tangent to yhe parabola at the point (2 , 3) and the x – axis is equal to
The area of the loop between the curve y = a sin x and the x – axis and x = 0 , x = π. is
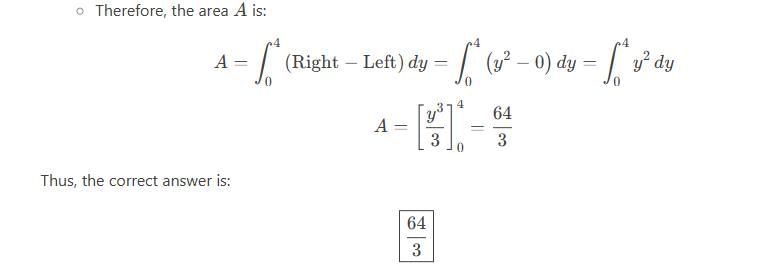
The area bounded by the curve y2 = x,line y = 4 and y – axis is equal to
The area enclosed by the curve xy2 = a2(a − x) and the y – axis is
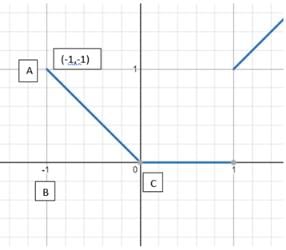
The area bounded by the curve y = x[x], the x – axis and the ordinates x = 1 and x = -1 is given by
The area bounded by the curves y = cos x and y = sin x between the ordinates x = 0 and x = π/2 is equal to
The area enclosed between the curves y = x3 ,x- axis and two ordinates x = 1 to x = 2 is [in square units]
The area bounded by y = log x , the x – axis and the ordinates x = 1 and x = 2 is
The area bounded by the curves y2 = x3 and |y| = 2x is given by
The area bounded by the curve y = 2x - x2 and the line x + y = 0 is
The area bounded by the parabola y = x2 and the line y = x is
Area of the region bounded by the curves y = ex, x = a , x = b and the x- axis is given by
The area bounded by the curves y2 = 4x and y = x is equal to
The area bounded by the curves y = √x, 2y+3 = x and the x- axis in the first quadrant is
Let y be the function which passes through (1 , 2) having slope (2x + 1) . The area bounded between the curve and the x – axis is
AOB is the positive quadrant of the ellipse in which OA = a and OB = b . The area between the arc AB and chord AB of the ellipse is
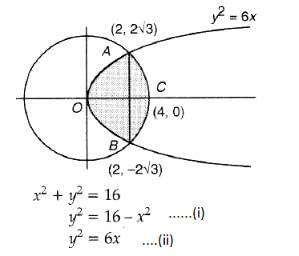
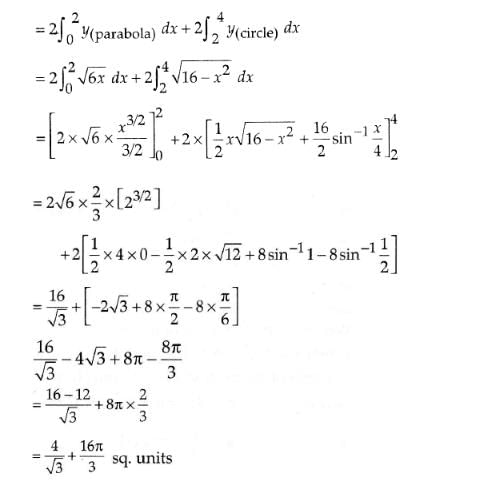
The area common to the circle x2+y2 = 16 and the parabola y2 = 6x is
The area included between the curves is equal to
The area bounded by the curves y2 = 20x and x2 = 16y is equal to
The area of the region bounded by the curves y = |x−2|, x = 1 , x = 3 and the x – axis is
|
203 videos|377 docs|167 tests
|