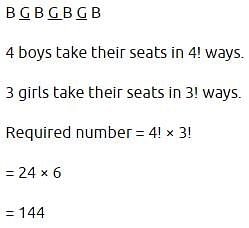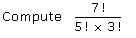Commerce Exam > Commerce Tests > Mathematics (Maths) Class 11 > Test: Permutation of Distinct Objects - Commerce MCQ
Test: Permutation of Distinct Objects - Commerce MCQ
Test Description
10 Questions MCQ Test Mathematics (Maths) Class 11 - Test: Permutation of Distinct Objects
Test: Permutation of Distinct Objects for Commerce 2025 is part of Mathematics (Maths) Class 11 preparation. The Test: Permutation of Distinct Objects questions and answers have been
prepared according to the Commerce exam syllabus.The Test: Permutation of Distinct Objects MCQs are made for Commerce 2025 Exam. Find important
definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Permutation of Distinct Objects below.
Solutions of Test: Permutation of Distinct Objects questions in English are available as part of our Mathematics (Maths) Class 11 for Commerce & Test: Permutation of Distinct Objects solutions in
Hindi for Mathematics (Maths) Class 11 course. Download more important topics, notes, lectures and mock
test series for Commerce Exam by signing up for free. Attempt Test: Permutation of Distinct Objects | 10 questions in 10 minutes | Mock test for Commerce preparation | Free important questions MCQ to study Mathematics (Maths) Class 11 for Commerce Exam | Download free PDF with solutions
Test: Permutation of Distinct Objects - Question 1
In how many ways 4 boys and 3 girls can be seated in a row so that they are alternate.
Detailed Solution for Test: Permutation of Distinct Objects - Question 1
Test: Permutation of Distinct Objects - Question 2
In how many ways 2 directors and 3 executives can be arranged for a meeting? If there are 6 chairs available two on one side and remaining four on the other side of the table and the two directors has to be together on one side and the executives on the other side.
Detailed Solution for Test: Permutation of Distinct Objects - Question 2
Test: Permutation of Distinct Objects - Question 3
A lady arranges a dinner party for 6 guests .The number of ways in which they may be selected from among 10 friends if 2 of the friends will not attend the party together is
Detailed Solution for Test: Permutation of Distinct Objects - Question 3
Detailed Solution for Test: Permutation of Distinct Objects - Question 4
Detailed Solution for Test: Permutation of Distinct Objects - Question 5
Test: Permutation of Distinct Objects - Question 6
Number of signals that can be made using 2 flags out of given 4 flags.
Detailed Solution for Test: Permutation of Distinct Objects - Question 6
Detailed Solution for Test: Permutation of Distinct Objects - Question 7
Detailed Solution for Test: Permutation of Distinct Objects - Question 8
Test: Permutation of Distinct Objects - Question 9
5 singers are to be given performance in a concert. How many ways can they be arranged in a order
Detailed Solution for Test: Permutation of Distinct Objects - Question 9
Detailed Solution for Test: Permutation of Distinct Objects - Question 10
|
75 videos|238 docs|91 tests
|
Information about Test: Permutation of Distinct Objects Page
In this test you can find the Exam questions for Test: Permutation of Distinct Objects solved & explained in the simplest way possible.
Besides giving Questions and answers for Test: Permutation of Distinct Objects, EduRev gives you an ample number of Online tests for practice