Test: Set Notations - Commerce MCQ
10 Questions MCQ Test Mathematics (Maths) Class 11 - Test: Set Notations
Let X = {x | x = 2 + 4k, where k = 0, 1, 2, 3,...24}. Let S be a subset of X such that the sum of no two elements of S is 100. What is the maximum possible number of elements in S ?
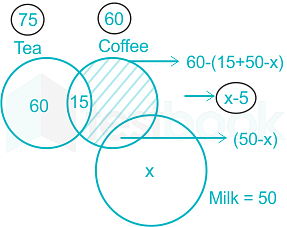
In a party of 150 persons, 75 persons take tea, 60 persons take coffee and 50 persons take milk. 15 of them take both tea and coffee, but no one taking milk takes tea. If each person in the party takes at least one drink, then what is the number of persons taking milk only ?
If A = {3, 6, 9, 12} and B = {6, 8, 9} then intersection of A and B is:
Consider the following statements:
I: If A = {x: x is an even natural number} and B = {y: y is a natural number}, A subset B.
II: Number of subsets for the given set A = {5, 6, 7, 8} is 12.
III: Number of proper subsets for the given set A = {5, 6, 7, 8} is 15.
Which of the following statement(s) is/are correct?
|
75 videos|238 docs|91 tests
|