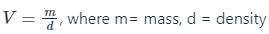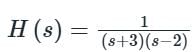Test: Properties of Systems - Electronics and Communication Engineering (ECE) MCQ
20 Questions MCQ Test - Test: Properties of Systems
A continuous-time system is memoryless if its output at any time ( t ) depends on:
For a system to have an inverse, which of the following must be true?
Within the super set of all input-output systems, the subset of linear systems is distinguished by which fundamental property?
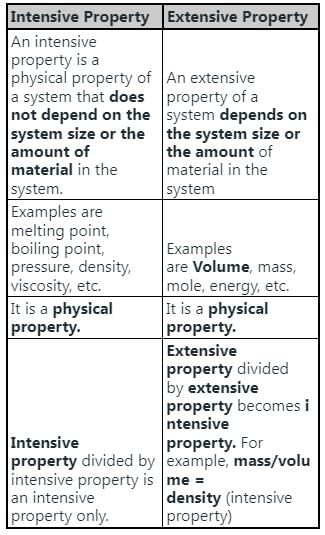
Specific enthalpy is an ________ of a system and its unit is ________.
What is the following type of system called? y[n] = x[n] + y[n-1].
Which property of delta function indicates the equality between the area under the product of function with shifted impulse and the value of function located at unit impulse instant ?
For what value of k, will the following system be time invariant?
y(t) = x(t) + x(kt) – x(2t) + x(t-1)
In the super set of signal processing operations for linear time-invariant (LTI) systems, the subset of convolution-based transformations includes the effect of time-scaling the input. Which property best describes how time scaling an input affects the convolution output?
A stable LTI system has a transfer function 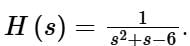 To make this system causal and stable it needs to be cascaded with another LTI system having transfer function H1(s). The correct choice for H1(s) is
To make this system causal and stable it needs to be cascaded with another LTI system having transfer function H1(s). The correct choice for H1(s) is
Consider a single input single output discrete-time system with x[n] as input and y[n] as output. Where the two are related as
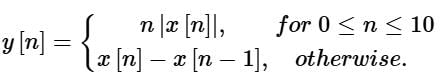
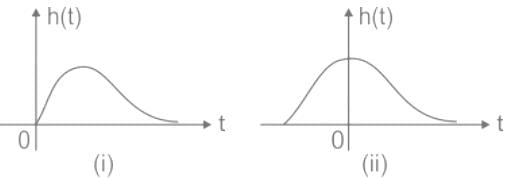
Which one of the following statements is true about the system?
Which of the following statements with respect to the stability of a control system is true?
a. The roots of the characteristic equation are on the left half of the s-plane.
b. The system is bounded input bounded output (BIBO) stable.
c. Stability is independent of the input.
d. The roots of the characteristic equation are on the left half of the s-plane and on the imaginary axis.