Electronics and Communication Engineering (ECE) Exam > Electronics and Communication Engineering (ECE) Tests > Test: BIBO Stability - Electronics and Communication Engineering (ECE) MCQ
Test: BIBO Stability - Electronics and Communication Engineering (ECE) MCQ
Test Description
10 Questions MCQ Test - Test: BIBO Stability
Test: BIBO Stability for Electronics and Communication Engineering (ECE) 2025 is part of Electronics and Communication Engineering (ECE) preparation. The Test: BIBO Stability questions and answers have been prepared
according to the Electronics and Communication Engineering (ECE) exam syllabus.The Test: BIBO Stability MCQs are made for Electronics and Communication Engineering (ECE) 2025 Exam.
Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: BIBO Stability below.
Solutions of Test: BIBO Stability questions in English are available as part of our course for Electronics and Communication Engineering (ECE) & Test: BIBO Stability solutions in
Hindi for Electronics and Communication Engineering (ECE) course.
Download more important topics, notes, lectures and mock test series for Electronics and Communication Engineering (ECE) Exam by signing up for free. Attempt Test: BIBO Stability | 10 questions in 10 minutes | Mock test for Electronics and Communication Engineering (ECE) preparation | Free important questions MCQ to study for Electronics and Communication Engineering (ECE) Exam | Download free PDF with solutions
Detailed Solution for Test: BIBO Stability - Question 1
Detailed Solution for Test: BIBO Stability - Question 2
Test: BIBO Stability - Question 3
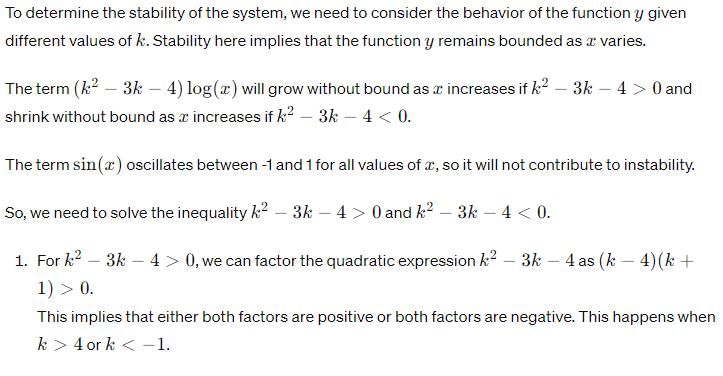
For what values of k is the following system stable, y = (k2 – 3k -4)log(x) + sin(x)?
Detailed Solution for Test: BIBO Stability - Question 3
Test: BIBO Stability - Question 4
For a bounded function, is the integral of the odd function from -infinity to +infinity defined and finite?
Detailed Solution for Test: BIBO Stability - Question 4
Test: BIBO Stability - Question 5
When a system is such that the square sum of its impulse response tends to infinity when summed over all real time space,
Detailed Solution for Test: BIBO Stability - Question 5
Detailed Solution for Test: BIBO Stability - Question 6
Detailed Solution for Test: BIBO Stability - Question 7
Test: BIBO Stability - Question 8
Comment on the stability of the following system, y[n] = n*x[n-1].
Detailed Solution for Test: BIBO Stability - Question 8
Detailed Solution for Test: BIBO Stability - Question 9
Test: BIBO Stability - Question 10
A control system represent by differential equation 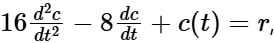 where r(t) is the input and c(t) is output. The system is excited with input of unit the step function. The response is similar to the waveform
where r(t) is the input and c(t) is output. The system is excited with input of unit the step function. The response is similar to the waveform
Detailed Solution for Test: BIBO Stability - Question 10
Information about Test: BIBO Stability Page
In this test you can find the Exam questions for Test: BIBO Stability solved & explained in the simplest way possible.
Besides giving Questions and answers for Test: BIBO Stability, EduRev gives you an ample number of Online tests for practice
Download as PDF