Test: Heron's Formula- 2 - Class 9 MCQ
25 Questions MCQ Test - Test: Heron's Formula- 2
Find the length of each side of an equilateral triangle having area of 9 root 3 cm square
If the height of a parallelogram having 500 cm2 as the area is 20 cm, then its base is of length
The area of an isosceles right angled triangle of equal side 30 cm, is given as
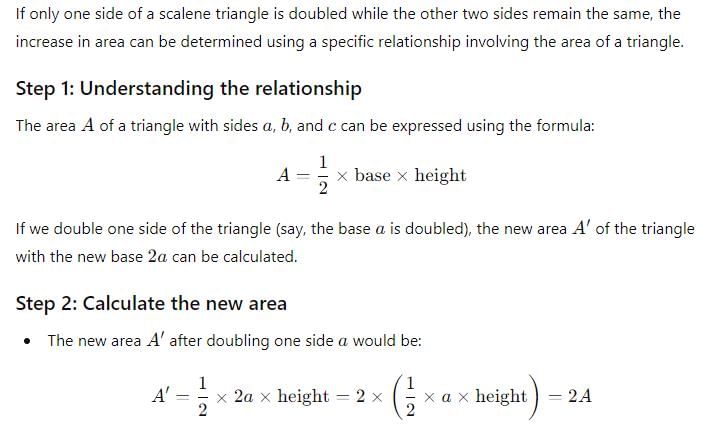
If one side of a scalene △ is doubled then area would be increased by
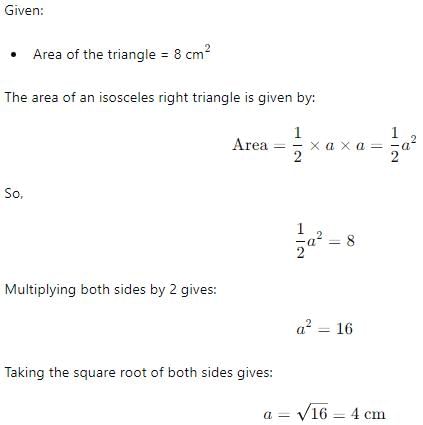
An isosceles right triangle has area 8 cm2. The length of its hypotenuse is :
If the area of an equilateral triangle is 36√3 cm2, then the perimeter of the triangle is
Given the product of diagonals of a rhombus ABCD is 2500 cm2, its area is
The area and length of one diagonal of a rhombus are given as 200 cm2 and 10 cm respectively. The length of other diagonal is
In each side of a △ is halved then its perimeter will be decreased by
In ∆ABC, angle A = 30°, side 'b' = 4 units, side 'c' = 6 units. Find the area of ∆ABC,
The edges of a triangular board are 6 cm, 8 cm and 10 cm. The cost of painting it at the rate of 70 paise per cm2 is
In triangle ABC, angle A = 120°, side b = 8 cm and side c = 10 cm. Find the area of triangle ABC.
The area of one triangular part of a rhombus ABCD is given as 125 cm2. The area of rhombus ABCD is
Length of perpendicular drawn on smallest side of scalene triangle is
The sides of a triangle are in ratio 3 : 4 : 5. If the perimeter of the triangle is 84 cm, then area of the triangle is :
The perimeter of a rhombus is 20 cm. If one of its diagonals is 6 cm, then its area is
Area of an isosceles triangle ABC with AB = a = AC and BC = b is
Each side of an equilateral triangle measures 10 cm. Then the area of the triangle is
Length of perpendicular drawn on longest side of a scale △ is
Each side of an equilateral triangle is 2x cm. If x√3 = √48, then area of the triangle is :
The area of a rhombus of 96 cm2. If one of its diagonals is 16 cm, then the length of its side is
The area of quadrilateral ABCD whose diagonals are perpendicular and of lengths 12 cm, 8 cm is
The area of a parallelogram whose base is 32 m and the corresponding altitude is 6 m is
Semiperimeter of scalene triangle of side k, 2k and 3k is
The sides of a triangle are x, y and z. If x + y = 7 m, y + z = 9 m, and z + x = 8 m, then area of the triangle is :