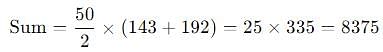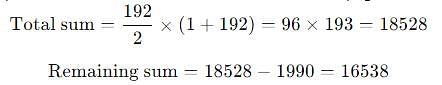Practice Test: Number System- 2 - Mechanical Engineering MCQ
20 Questions MCQ Test General Aptitude for GATE - Practice Test: Number System- 2
The HCF of two numbers is 11 and their LCM is 616. If one of the numbers is 77, find the other number.
The total number of 3 digit numbers which have two or more consecutive digits identical is:
Two players A and B are playing a game of putting ‘+’ and '-'signs in between any two integers written from 1 to 100. A starts the game by putting a plus sign anywhere between any two integers. Once all the signs have been put, the result is calculated. If it is even then A wins and if it is odd then B wins, provided they are putting signs by taking turns one by one and either of them can put any sign anywhere between any two integers. Who will win at the end?
How many divisors of 105 will have at least one zero at its end?
Tatto bought a notebook containing 96 pages leaves and numbered them which came to 192 pages. Tappo tore out the latter 25 leaves of the notebook and added the 50 numbers she found on those pages. Which of the following is not true?
There are 50 integers a1, a2,........, a50, not all of them necessarily different. Let the greatest integer of these 50 integers be referred to as G, and the smallest integer is referred to as L. The integers a1 through a24 form sequence S1, and the rest form sequence S2. Each member of S1 is less than or equal to each member of S2.
Every element of S1 is made greater than or equal to every element of S2 by adding to each element of S1 an integer x. Then, x cannot be less than:
Srini wrote his class 10th board examination this year. When the result came out he searched for his hall ticket to see his roll number but could not trace it. He could remember only the first three digits of the 6 digit number as 267. His father, however, remembered that the number was divisible by 11. His mother gave the information that the number was also divisible by 13. They tried to recollect the number when all of a sudden Srini told that the number was a multiple of 7. What was the unit digits of the number?
When 7179 and 9699 are divided by another natural number N , remainder obtained is same. How many values of N will be ending with one or more than one zeroes?
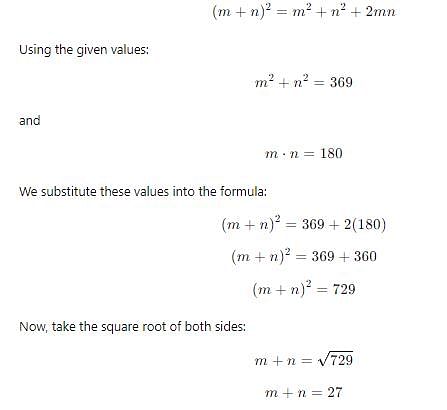
If the product of two numbers is 180 and the sum of their squares is 369, then what is the sum of the numbers?
Find the remainder when 73 * 75 * 78 * 57 * 197 * 37 is divided by 34.
Three distinct prime numbers, less than 10 are taken and all the numbers that can be formed by arranging all the digits are taken. Now, difference between the largest and the smallest number formed is equal to 495. It is also given that sum of the digits is more than 13. What is the product of the numbers?
What will be remainder when 1212121212... 300 times, is being divided by 99?
Which of the following would always divide a six-digit number of the form ababab?
When a number is successively divided by 7,5 and 4, it leaves remainders of 4,2 and 3 respectively. What will be the respective remainders when the smallest such number is successively divided by 8,5 and 6 ?
Four bells ringing together and ring at an interval of 12 sec, 15 sec, 20 sec, and 30 sec respectively. How many times will they ring together in 8 hours?
What would be the greatest number that divides 14, 20, and 32 leaving the same remainder?
|
194 videos|165 docs|152 tests
|