Test: CAT Quant 2019 ( Shift -1) - CAT MCQ
30 Questions MCQ Test - Test: CAT Quant 2019 ( Shift -1)
Two cars travel the same distance starting at 10:00 am and 11:00 am, respectively, on the same day. They reach their common destination at the same point of time. If the first car travelled for at least 6 hours, then the highest possible value of the percentage by which the speed of the second car could exceed that of the first car is
If a1, a2 ... are in A.P., then,
1/√a1 +√a2 + 1/√a2 + √a3 + …………… 1/√an +√an+1 is equal to
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
AB is a diameter of a circle of radius 5 cm. Let P and Q be two points on the circle so that the length of PB is 6 cm, and the length of AP is twice that of AQ. Then the length, in cm, of QB is nearest to
Option~
(a) 9.3
(b) 7.8
(c) 9.1
(d) 8.5
If (5.55) x = (0.555) y = 1000, then the value of 1/x – 1/y is
The income of Amala is 20% more than that of Bimala and 20% less than that of Kamala. If Kamala's income goes down by 4% and Bimala's goes up by 10%, then the percentage by which Kamala's income would exceed Bimala's is nearest to
The wheels of bicycles A and B have radii 30 cm and 40 cm, respectively. While traveling a certain distance, each wheel of A required 5000 more revolutions than each wheel of B. If bicycle B traveled this distance in 45 minutes, then its speed, in km per hour, was
The product of the distinct roots of | x2- x - 6 | = x + 2 is
In a race of three horses, the first beat the second by 11 metres and the third by 90 metres. If the second beat the third by 80 metres, what was the length, in metres, of the racecourse?
If the population of a town is p in the beginning of any year then it becomes 3 + 2p in the beginning of the next year. If the population in the beginning of 2019 is 1000, then the population in the beginning of 2034 will be
Consider a function f satisfying f (x + y) = f (x) f (y) where x,y are positive integers, and f(1) = 2. If f(a + 1) +f (a + 2) +... + f(a + n) = 16 (2n -1) then a is equal to
Amala, Bina, and Gouri invest money in the ratio 3: 4: 5 in fixed deposits having respective annual interest rates in the ratio 6:5:4. What is their total interest income (in Rs) after a year, if Bina's interest income exceeds Amala's by Rs 250?
For any positive integer n, let f (n) = n (n + 1) if n is even, and f (n) = n + 3 if n is odd. If m is a positive integer such that 8f (m + 1) - f (m) = 2, then m equals
The product of two positive numbers is 616. If the ratio of the difference of their cubes to the cube of their difference is 157:3, then the sum of the two numbers is
One can use three different transports which move at 10,20, and 30 kmph, respectively to reach from A to B. Amal took each mode of transport for 1/3 rd of his total journey time, while Bimal took each mode of transport for 1/3rd of the total distance. The percentage by which Bimal's travel time exceeds Amal's travel time is nearest to
Meena scores 40% in an examination and after review, even though her score is increased by 50%, she fails by 35 marks. If her postreview score is increased by 20%, she will have 7 marks more than thepassing score. The percentage score needed for passing the examination is
A person invested a total amount of Rs 15 lakh. A part of it was invested in a fixed deposit earning 6% annual interest, and the remaining amount was invested in two other deposits in the ratio 2:1, earning annual interest at the rates of 4% and 3%, respectively. If the total annual interest income is Rs 76000 then the amount (in Rs lakh) invested in the fixed deposit was
A club has 256 members of whom 144 can play football, 123 can play tennis, and 132 can play cricket. Moreover, 58 members can play both football and tennis, 25 can play both cricket and tennis, while 63 can play both football and cricket. If every member can play at least one game, then the number of members who can play only tennis is
If a1 + a2 + a3 +.... + an = 3(2n+1 - 2), for every n ≥ 1, then a11 equals
The number of the real roots of the equation 2 cos(x(x + 1)) = 2x + 2-x is
At their usual efficiency levels, A and B together finish a task in 12 days. If A had worked half as efficiently as she usually does, and B had worked thrice as efficiently as he usually does, the task would have been completed in 9 days. How many days would A take to finish the task if she works alone at her usual efficiency?
In a class, 60% of the students are girls and the rest are boys. There are 30 more girls than boys. If 68% of the students, including 30 boys, pass an examination, the percentage of the girls who do not pass is
In a circle of radius 11 cm, CD is a diameter and AB is a chord of length 20.5 cm. If AB and CD intersect at a point E inside the circle and CE has length 7 cm, then the difference of the lengths of BE and AE, in cm, is
On selling a pen at 5% loss and a book at 15% gain, Karim gains Rs. 7. If he Sells the pen at 5% gain and the book at 10% gain, he gains Rs. 13. What is the cost price of the book in Rupees?
A chemist mixes two liquids 1 and 2. One litre of liquid 1 weighs 1 kg and one litre of liquid 2 weighs 800 gm. If half litre of the mixture weighs 480 gm, then the percentage of liquid 1 in the mixture, in terms of volume, is
Ramesh and Gautam are among 22 students who write an examination. Ramesh scores 82.5. The average score of the 21 students other than Gautam is 62. The average score of all the 22 students is one more than the average score of the 21 students other than Ramesh. The score of Gautam is
If m and n are integers such that (√2 )1934429m8n = 3n16m(√64 ) then m is
Three men and eight machines can finish a job in half the time taken by three machines and eight men to finish the same job. If two machines can finish the job in 13 days, then how many men can finish the job in 13 days?
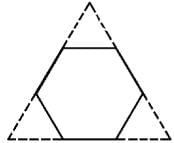
Corners are cut off from an equilateral triangle T to produce a regular hexagon H. Then, the ratio of the area of H to the area of T is
Let x and y be positive real numbers such that
log5 (x + y) + log5 (x - y) = 3, and log2 y - log2 x = 1 - log2 3. Then xy equals
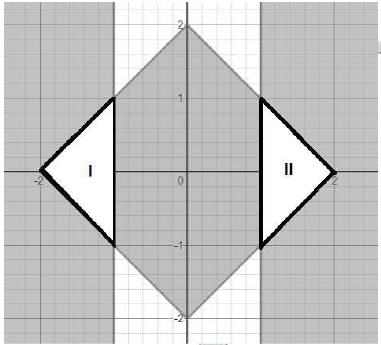
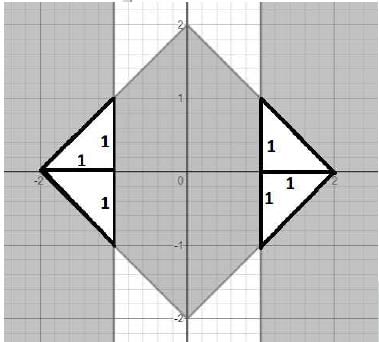
Let S be the set of all points (x, y) in the x-y plane such that |x|+|y|≤2 and |x| ≥ 1. Then, the area, in square units, of the regior represented by S equals