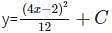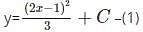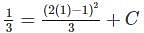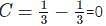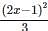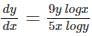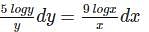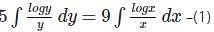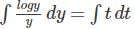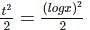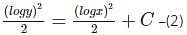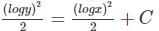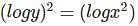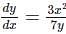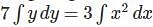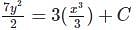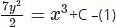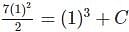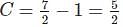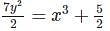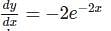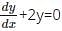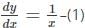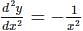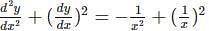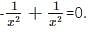Mathematics: CUET Mock Test - 4 - CUET MCQ
30 Questions MCQ Test CUET Mock Test Series - Mathematics: CUET Mock Test - 4
Which one of the following is correct if we differentiate the equation xy = aex + be-x two times?
What is the differential equation of all parabolas whose directrices are parallel to the x-axis?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
What will be the required solution of d2y/dx2 – 3dy/dx + 4y = 0?
What is the solution of the given equation (D + 1)2y = 0 given y = 2 loge 2 when x = loge 2 and y = (4/3) loge3 when x = loge3?
If, A and B are arbitrary constants then what will be the differential equation of y = Ax + B/x?
What will be the value of C if C the constant of the coefficient of the solution of the given equation (D + 1)2y = 0 given y = 2 loge 2 when x = loge 2 and y = (4/3) loge3 when x = loge3?
What is thedifferential equation whose solution represents the family y = ae3x + bex?
If y = t(x) be a differentiable function ᵾ x € R, then which of the following is always true?
What will be the general solution of the differential equation d2y/dx2 = e2x(12 cos3x – 5 sin3x)? (here, A and B are integration constant)
Which of the following is the valid differential equation x = a cos(αt + β)?
If, A normal is drawn at a point P(x, y) of a curve. It meets the x-axis at Q. If PQ is of constant length k. What kind of curve is passing through (0, k)?
What is the solution of (y(dy/dx) + 2x)2 = (y2 + 2x2)[1 + (dy/dx)2]?
A particle starts from the origin with a velocity 5cm/sec and moves in a straight line, its acceleration at time t seconds being (3t2 – 5t)cm/sec2. What will be the velocity of the particle?
What will be the differential equation form of √(a2 + x2)dy/dx + y = √(a2 + x2) – x?
A curve passes through (1, 1) such that the triangle formed by the coordinate axes and the tangent at any point of the curve is in the first quadrant and has its area equal to 2. What will be the equation of the curve?
A curve passes through (1, 1) such that the triangle formed by the coordinate axes and the tangent at any point of the curve is in the first quadrant and has its area equal to 2. What is the differential equation?
What will be the value of dy/dx – a/x * y = (x + 1)/x?
What is the solution of dy/dx = (6x + 9y – 7)/(2x + 3y – 6)?
A particle starts from the origin with a velocity 5cm/sec and moves in a straight line, its acceleration at time t seconds being (3t2 – 5t)cm/sec2. What will be the distance from the origin at the end of 4 seconds?
What is the equation of the curve passing through (1, 0) of (y(dy/dx) + 2x)2 = (y2 + 2x2)[1 + (dy/dx)2]?
Find the general solution of the differential equation 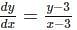 (x, y≠3).
(x, y≠3).
Find the general solution of the differential equation 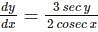 .
.
Find the general solution of the differential equation 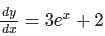
Find the particular solution of the differential equation 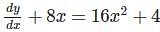 given that y = 1/3 when x = 1.
given that y = 1/3 when x = 1.
Find the particular solution of the differential equation 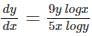 .
.
Find the particular solution for the differential equation 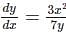 given that, y = 1 when x = 1.
given that, y = 1 when x = 1.
Which of the following functions is the solution of the differential equation dydx + 2y = 0?
Which of the following functions is a solution for the differential equation xy’ - y = 0?
Which of the following functions is a solution for the differential equation y” + 6y = 0?
Which of the following differential equations given below has the solution y = logx?
|
8 docs|148 tests
|


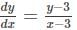
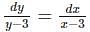
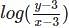 = logC1
= logC1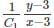 = 0
= 0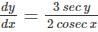
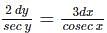
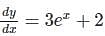
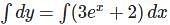 -----(1)
-----(1)