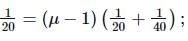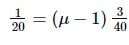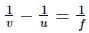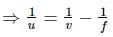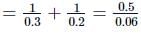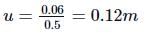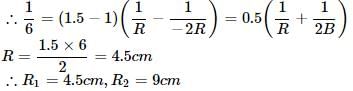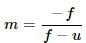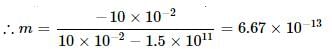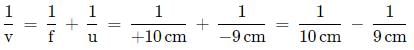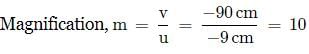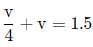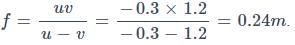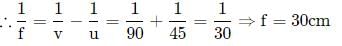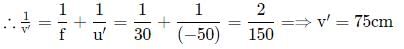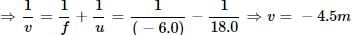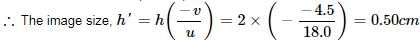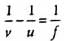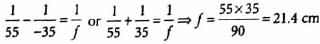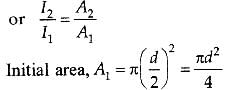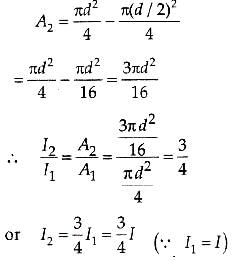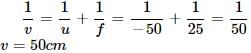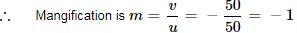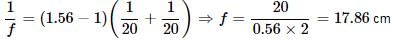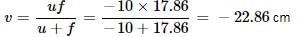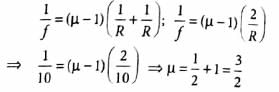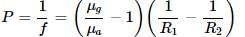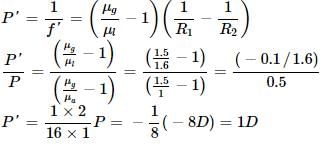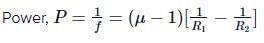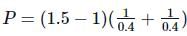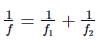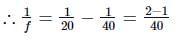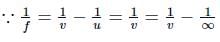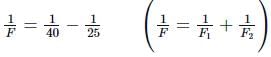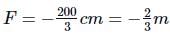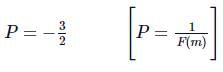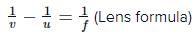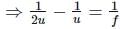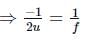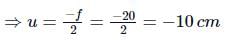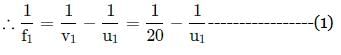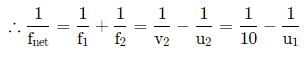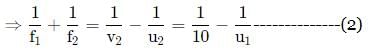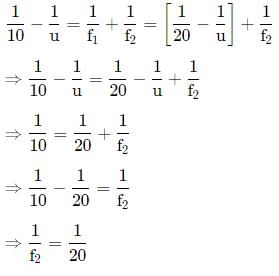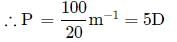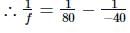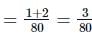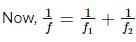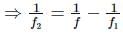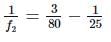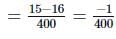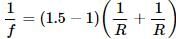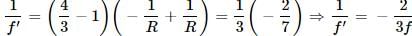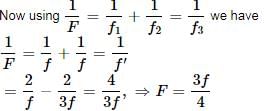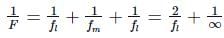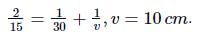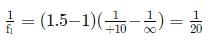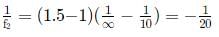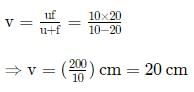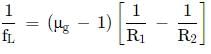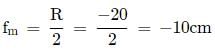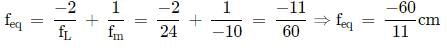All Courses
Get the App Signup / Login
Sign in Open App
NEET Exam  >  NEET Tests  >  NCERTs at Fingertips: Textbooks, Tests & Solutions  >  Test: Refraction at Spherical Surfaces & Lenses (NCERT) - NEET MCQ

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - NEET MCQ


Test Description

30 Questions MCQ Test NCERTs at Fingertips: Textbooks, Tests & Solutions - Test: Refraction at Spherical Surfaces & Lenses (NCERT)

Test: Refraction at Spherical Surfaces & Lenses (NCERT) for NEET 2024 is part of NCERTs at Fingertips: Textbooks, Tests & Solutions preparation. The Test: Refraction at Spherical Surfaces & Lenses (NCERT) questions and answers have been prepared according to the NEET exam syllabus.The Test: Refraction at Spherical Surfaces & Lenses (NCERT) MCQs are made for NEET 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Refraction at Spherical Surfaces & Lenses (NCERT) below.
Solutions of Test: Refraction at Spherical Surfaces & Lenses (NCERT) questions in English are available as part of our NCERTs at Fingertips: Textbooks, Tests & Solutions for NEET & Test: Refraction at Spherical Surfaces & Lenses (NCERT) solutions in Hindi for NCERTs at Fingertips: Textbooks, Tests & Solutions course. Download more important topics, notes, lectures and mock test series for NEET Exam by signing up for free. Attempt Test: Refraction at Spherical Surfaces & Lenses (NCERT) | 30 questions in 30 minutes | Mock test for NEET preparation | Free important questions MCQ to study NCERTs at Fingertips: Textbooks, Tests & Solutions for NEET Exam | Download free PDF with solutions
Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 1
Save

The radii of curvature of the surfaces of a double convex lens are 20cm and 40cm respectively, and its focal length is 20cm. What is the refractive index of the material of the lens?

Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 1

Here, R1 = 20cm,R2 = −40cm, f = 20cm
Using lens makers formula we get,


⇒ μ = 5/3

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 2
Save

A convex lens is dipped in a liquid whose refractive index is equal to the refractive index of the lens. Then its focal length will

Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 2

When refractive index of lens is equal to the refractive index of liquid, the lens be have lik e a plane surface with focal length infinity.

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 3
Save

A convergent beam of light passes through a diverging lens of focal length 0.2m and comes to focus 0.3m behind the lens. The position of the point at which the beam would converge in the absence of the lens is

Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 3

Here, f = −0.2m,v = +0.3m
The lens formula



Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 4
Save

Radii of curvature of a converging lens are in the ratio 1:2. Its focal length is 6cm and refractive index is 1.5. Then its radii of curvature are
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 4

Here, f = 6cm, μ = 1.5, R1 = R, R2 = −2R
According to lens maker's formula

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 5
Save

A man is trying to start a fire by focusing sunlight on a piece of paper using an equiconvex lens of focal length 10 cm. The diameter of the sun is 1.39 × 109 m and its mean distance from the earth is 1.5 × 1011m. What is the diameter of the sun's image on the paper?
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 5

Magnification for convex lens,

Here, f = 10cm = 10 × 10−2 m, u = 1.5 × 1011 m

∴  Diameter of the image,
d = m × 1.39 × 109
= 6.67 × 10−13 × 1.39 × 109
= 9.27 × 10−4m

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 6
Save

A square card of side length 1mm is being seen through a magnifying lens of focal length 10cm. The card is placed at a distance of 9cm from the lens. The apparent area of the card through the lens is
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 6

Area of square card = 1 mm × 1 mm = 1 mm2
Focal length of magnifying lens, f = +10 cm
Object distance, u = -9 cm
According to thin lens formula

or v = -90 cm

∴ Apparent area of the card through the  lens 
= 10 × 10 × 1 mm2
 = 100 mm2
 = 1 cm2

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 7
Save

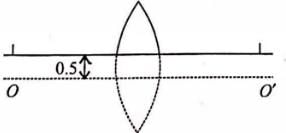
A converging lens is used to form an image on a screen. When the upper half of the lens is covered by an opaque screen
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 7

Image formed is complete but has decreased intensity.

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 8
Save

Which of the following forms a virtual and erect image for all positions of the object?
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 8

Concave lens and convex mirror are diverging in nature. They form virtual and erect images.

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 9
Save

A real image of a distance object is formed by a plano-convex lens on its principal axis. Spherical aberration is
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 9

When the curved surface of the lens faces the object, the spherical aberration is smaller. The total deviation is shared between the curved and the plane surfaces.

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 10
Save

An object is placed at a distance of 1.5 m from a screen and a convex lens is interposed between them. The magnification produced is 4. The focal length of the lens is then
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 10

Here, m = u/v = − 4 or u = -v/4
Also, ∣u∣ + ∣v∣ = 1.5

or v = 1.2 m and u = -1.2/4 = -0.3 m

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 11
Save

The image of the needle placed 45 cm from a lens is formed on a screen placed 90 cm on the other side of the lens. The displacement of the image, if the needle is moved by 5.0 cm away from the lens is 
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 11

Here, u = -45 cm,  v = 90 cm 

when the needle is move 5 cm away from the lens,
u = -(45 + 5) = -50 cm

∴ Displacement of image = v - v' = 90 - 75 = 15 cm, towards the lens

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 12
Save

A tree is 18.0 m away and 2.0 m high from a concave lens. How high is the image formed by the given lens of focal length 6m
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 12

Focal length of the lens is f = −6.0 m
u = −18 m and h = 2 m

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 13
Save

A luminous object is separated from a screen by distance d. A convex lens is placed between the object and the screen such that it forms a distinct image on the screen. The maximum possible focal length of this convex lens is
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 13

From lens displacement method, fmax = d/4

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 14
Save

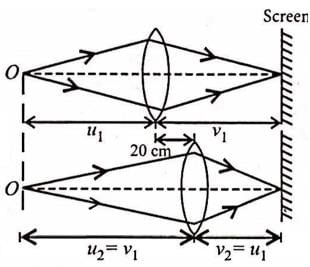
A screen is placed 90cm away from an object. The image of the object on the screen is formed by a convex lens at two different locations separated by 20cm. Find the focal length of lens.
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 14

The image of the object can be located on the screen for two positions of convex lens such that u and v are exchanged.
The separation between two positions of the lens is d = 20cm

 

From the figure.
u1 + v1 = 90 cm
v1 − u1 = 20 cm
Solving
v1 = 55cm, u1 = 35cm
From lens formula,

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 15
Save

A lens having focal length f and aperture of diameter d forms an image of intensity I. Aperture of diameter d/2 in central region of lens is covered by a black paper. Focal length of lens and intensity of image now will be respectively
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 15

Focal length of the lens remains same.
Intensity of image formed by lens is proportional to area exposed to incident light from object.
i.e.  Intensity ∝ area


After blocking, exposed area,

Hence, focal length of a lens = f, intensity of the image = 3I/4

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 16
Save

A thin convex lens of focal length 25cm is cut into two pieces 0.5cm above the principal axis. The top part is placed at (0, 0) and an object placed at (−50cm, 0). Find the coordinates of the image.
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 16


If there was no cut then the object would have been at a height of 0.5cm from the principle axis OO′.
Consider the image for this case


Thus the image would have been formed at 50 cm from the pole and 0.5 cm below the principal axis.
Hence with respect to x-axis passing through the edge of the cut lens, the co-ordinates of the image are (50 cm, −1 cm)

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 17
Save

A double convex lens made of glass of refractive index 1.56 has both radii of curvature of magnitude 20cm. If an object is placed at a distance of 10cm from this lens, the position of the image formed is
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 17

Here, R1 = 20 cm, R2 = −20 cm, u = −10 cm and μ = 1.56
Using lens make'rs formula,

Now, from lens equation,

Since v is negative, the image will be formed on the same side as that of object.

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 18
Save

The power of biconvex lens is 10 dioptre and the radius of curvature of each surface is 10 cm. Then the refractive index of the material of the lens is
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 18

Power of lens, P (in dioptre) = 100/f(in cm)
∴  f  = 100/10 = 10 cm
For biconvex lens, R1 = + R, R2 = -R =  According to lens makers formula

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 19
Save

A thin glass (refractive index 1.5) lens has optical power of -8 D in air. Its optical power in a liquid medium with refractive index 1.6 will be :
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 19

In air,

In medium

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 20
Save

The radius of curvature of each surface of a convex lens of refractive index 1.5 is 40cm. Its power is
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 20


Here, μ = 1.5, R1 = 40 cm = 0.4 cm, R2 = −0.4m

= 2.5D

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 21
Save

Two lenses of focal lengths 20cm and −40cm are held in contact. The image of an object at infinity will be formed by the combination at
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 21

Equivalent focal length is given by,

Here, f1 = 20cm and f2 = −40cm

⇒ f = 40cm

⇒ v = f = 40 cm [∵ u = ∞]

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 22
Save

An eye specialist prescribes spectacles having combination of convex lens of focal length 40 cm in contact with a concave lens of focal length 25 cm. The power of this lens combination in diopters is

(a) +1.5
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 22

The focal length of combination is given by

or 

∴ Power of the combination in dioptres, 

= -1.5

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 23
Save

Two lenses of power +10D and −5D are placed in contact. Where should an object be held from the lens, so as to obtain a virtual image of magnification 2?
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 23

P1 = +10D, P2 = −5D
P = P1 + P2 = 10−5 = 5D
f = 100/5 = 20cm
Magnification = v/u = 2
v = 2u




 

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 24
Save

A real image of an object is formed at a distance of 20 cm from a lens. On putting another lens in contact with it, the image is shifted 10 cm towards the combination. The power of the lens is
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 24

As image formed is real, therefore lens must be convex, v = 20cm.
Let f1, be the focal length for this lens and f2 be the focal length of the other lens put in contact later.
for the first lens, 

After placing it in contact with another lens, the image shifted to 10cm towards the combination
i.e., v2 = (20 − 10) cm = 10 cm
Let fnet be the effective focal length of the combination, which can be given as:


From equation (1) and (2):

Hence, f2 = 20cm

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 25
Save

A concave lens is placed in contact with a convex lens of focal length 25 cm. The combination produces a real image at a distance of 80 cm, If an object is at a distance of 40 cm, the focal length of concave lens is
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 25

For the combination of lens,
Here, u = −40 cm, v = +80 cm,f = ?






⇒ f2 = −400cm

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 26
Save

Two identical glass (μg = 3/2)  equiconvex lenses of focal length f each are kept in contact. The space between the two lenses is filled with water (μw = 4/3). The focal length of the combination is
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 26

Let R be the radius of curvature of each surface. Then

For the water lens

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 27
Save

A convex lens of focal length 15 cm is placed on a plane mirror. An object is placed at 30 cm from the lens. The image is
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 27


⇒ F = 15/2 cm
∴ image is real, 10 cm in front of mirror.

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 28
Save

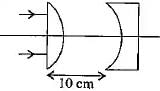
In the given figure, the radius of curvature of curved surface for both the plano-convex and plano-concave lens is 10 cm and refractive index for both is 1.5. The location of the final image after all the refractions through lenses is
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 28

The focal length of the plano - convex lens is:

Focal length of plano - concave lens is:

since parallel beams are incident on the lens, its image from plano - concave lens will be formed at +20 cm from it (at the focus) and will act as an object for the plano - concave lens. since the two lens are at a distance of 10 cm from each other, therefore, for the next lens
u = +10 cm

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 29
Save

A convex lens of radii of curvature 20 cm and 30 cm respectively. It is silvered at the surface which has smaller radius of curvature. Then it will behave as (μg =1.5)
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 29

Focal length for lens,

Here, μg = 1.5, R1 = 20 cm, R2 = −30cm

Focal length for mirror, 

∴ Equivalent focal length

Hence, this system behaves like a concave mirror of local length -60/11 cm

Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 30
Save

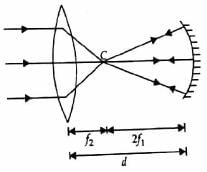
A concave mirror of focal length f1 is placed at a distance of d from a convex lens of focal length f2. A beam of light coming from infinity and falling on this convex lens - concave mirror combination returns to infinity. The distance d must equal
Detailed Solution for Test: Refraction at Spherical Surfaces & Lenses (NCERT) - Question 30


∴ d = 2f1 + f2 

257 docs|234 tests
Join Course
Information about Test: Refraction at Spherical Surfaces & Lenses (NCERT) Page
In this test you can find the Exam questions for Test: Refraction at Spherical Surfaces & Lenses (NCERT) solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Refraction at Spherical Surfaces & Lenses (NCERT), EduRev gives you an ample number of Online tests for practice

Top Courses for NEET

Download as PDF

Top Courses for NEET

Important Questions for Refraction at Spherical Surfaces & Lenses (NCERT)

Find all the important questions for Refraction at Spherical Surfaces & Lenses (NCERT) at EduRev.Get fully prepared for Refraction at Spherical Surfaces & Lenses (NCERT) with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Refraction at Spherical Surfaces & Lenses (NCERT) syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Refraction at Spherical Surfaces & Lenses (NCERT) resources.

Refraction at Spherical Surfaces & Lenses (NCERT) MCQs with Answers

Prepare for the Refraction at Spherical Surfaces & Lenses (NCERT) within the NEET exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Refraction at Spherical Surfaces & Lenses (NCERT) NCERTs at Fingertips: Textbooks, Tests & Solutions

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Refraction at Spherical Surfaces & Lenses (NCERT) with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Follow Us
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup