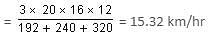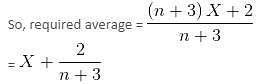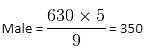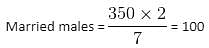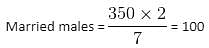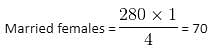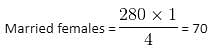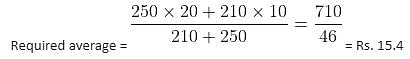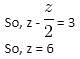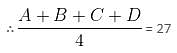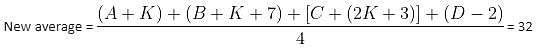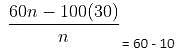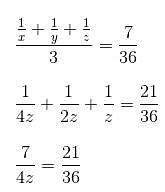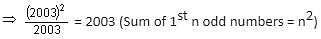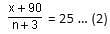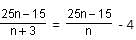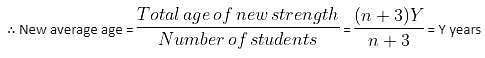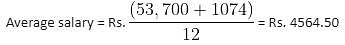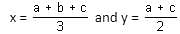Test Level 2: Averages - 2 - CAT MCQ
20 Questions MCQ Test Level-wise Tests for CAT - Test Level 2: Averages - 2
The average of x and y is 4 and both x and y are positive integers. How many pairs of (x, y) satisfy this condition?
The average speed of a cyclist who covers first, second and third km at speeds of 20 km/hr, 16 km/hr and 12 km/hr, respectively, is
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
The average age of a class of n boys is X years. Three new boys join the class whose ages are X - 1, X + 1 and X + 2 years, respectively. What is the new average age of the class?
The average age of a family of 5 members is 22 years. If the age of the youngest member is 10 years, then what was the average age of the family at the birth of the youngest member?
The average weight of a class of 35 students is 47.5 kg. If the weight of the teacher is included, the average rises by 500 g. The weight of the teacher is
The average marks of students A, B and C are 48. Another student (D) joins the group and the new average becomes 44 marks. If again, another student (E), who has three marks more than D, joins the group, the average of students B, C, D and E becomes 43 marks. How many marks did A get in the exam?
In a party the ratio of male and female is 5 : 4, the ratio of married and bachelor males is 2 : 5 and the ratio of married and unmarried females is 1 : 3. Total persons in the party are 630. If a man contributes Rs. 20 and a female Rs. 10, then what is the average contribution (in Rs.) per head by unmarried persons?
Average of three numbers is x. Instead of the first number if we take only half of that number, the average will be x - 1. If twice the first number is taken in place of the first number, what will be the average?
Total expenses of a boarding house are partly fixed and partly varying linearly with the number of boarders. The average expense per boarder is Rs. 700 when there are 25 boarders, and Rs. 600 when there are 50 boarders. What will be the average expense per boarder when there are 100 boarders?
The average of four numbers is 27. If the first number is increased by K, the second by (K + 7), the third by (2K + 3) and the fourth is decreased by 2, then the new average of the four numbers will become 32. Find the value of K.
Amol was asked to calculate the arithmetic mean of ten positive integers, each of which had two digits. By mistake, he interchanged the two digits, say a and b, in one of these ten integers i.e. ab. As a result, his answer for the arithmetic mean was 1.8 more than what it should have been. Then, b - a is equal to
In an exam, the average score of the candidates was found to be 60. After deducting computational errors, the scores of 100 candidates had to be changed from 80 to 50 each, and the overall average score of students had to be reduced by 10. What was the total number of students in the class?
Mr. Rajan takes three numbers. Out of these three numbers, the second is twice the third and the first is twice the second. The average of the reciprocal of the numbers is 7/36. Find the sum of all the three numbers.
A publisher incurs a fixed expense of Rs. 2,000 towards composing and other activities in printing books. A variable cost of Rs. 2.25 per book is incurred towards paper, binding charges etc. What is the average cost per book if 40,000 copies are printed?
There are some consecutive numbers and 'x' is the average of all the numbers, except the first number. If 'y' is the average of all the numbers, except the last number, then what is the value of x - y?
P = {1, 2, 3, 4… 2n + 1} is a set, where n is a positive integer and n > 2000. If A is the average of all the even numbers of the set P and B is the average of all the odd numbers of the set P, then what is the difference between A and B?
An enterprise got a bonus and decided to share it in equal parts between the workers. Three more workers joined the enterprise. Hence, each of them got 4 rupees less. The administration had found the possibility to increase the total sum of the bonus by 90 rupees and as a result, each worker including the three new workers got 25 rupees. How many total workers are there now?
The present average age of n boys in Class VIII is Y years. Three new boys join the class, whose ages are Y - 1, Y - 2 and Y + 3 years. What will be the new average age (in years) of the class, when all the students are promoted to Class X(tenth)?
A salesman gets a bonus of Rs. (3x + 7), if he sells articles worth Rs. x2. Apart from this, he receives a basic salary, which is 50 times his total bonus. In the month of January, the value of his sale was Rs. 100, in February, it was Rs. 400, and from March to December, it was Rs. 900 each month. Find his average salary per month for the year.
x is the average of three different integers, y is the average of minimum and maximum integers among the three. If x is two more than y, what is the difference between x and the third integer, which is neither minimum nor maximum?
|
5 docs|272 tests
|