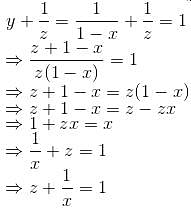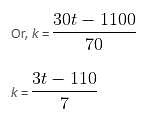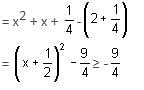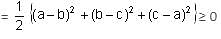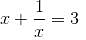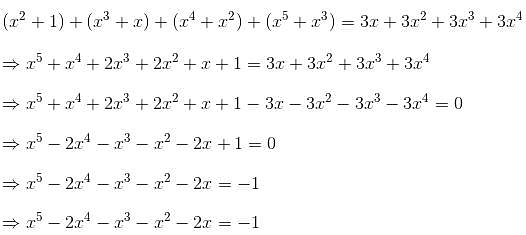Test Level 2: Quadratic Equations & Linear Equations - 2 - CAT MCQ
20 Questions MCQ Test Level-wise Tests for CAT - Test Level 2: Quadratic Equations & Linear Equations - 2
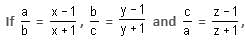
then which of the following is true?
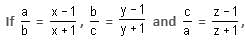
then which of the following is true?
The sum of ages of a group of teachers, doctors and lawyers is 2160 years and their average age is 36 years. If each teacher had been older by 1 year, each doctor by 6 years and each lawyer by 7 years, their average age would have increased by 5 years. Find the minimum possible number of doctors in the group.
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
What is the value of the expression 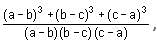 when a = 333, b = 444 and c = 555?
when a = 333, b = 444 and c = 555?
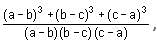 when a = 333, b = 444 and c = 555?
when a = 333, b = 444 and c = 555?The number of real solutions of |x|2 + 3 |x| + 2 = 0 is
The condition that the roots of the equation lx2 + mx + n = 0 are in the ratio 3 : 4, is
How many possible integral values are there for m if it is known that m2 + 12m + 20 ≤ 0?
A and B had certain number of stamps. A said to B, ''If you give me one of your stamps, we shall have equal number of stamps''. B replies, ''If you give me one of your stamps, I shall have twice as many as you will be left with''. Find the total number of stamps with A and B.
If p = x2 - yz, q = y2 - zx and r = z2 - xy, then the value of
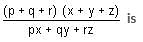
If α, β and γ are the roots of the equation 2x3 - 3x2 - 5x + 6 = 0, then α2 + β2 + γ2 is equal to
If a + b = 2c, then what is the value of
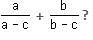
The set of value of λ for which both the roots of the equation x2 - (λ + 1)x + λ + 4 = 0 are negative, is
If x2 = y + z, y2 = z + x and z2 = x + y, then the value of
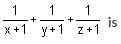
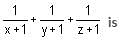
A famous cricketer Mr. Michael Swrfieai went to purchase a certain number of balls. There were two types of balls in the outlet: tennis balls and leather balls. A leather ball was 70 cents costlier than a tennis ball. If it is known that he purchased a total of 30 balls and spent $32(1$ = 100 cents) on purchasing the balls, what could be the possible cost of a tennis ball?
If p, q are roots (non-zero) of the equation x2 + px + q = 0, then the least value of x2 + px + q is
If x, y and z are real numbers, then x2 + 4y2 + 9z2 - 6yz - 3zx - 2xy is always
If y = x - 1/x, the expression 5x4 + 3x3 - 7x2 - 3x + 5 can be expressed as
If 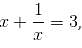 then find the value of (x5 - 2x4 - x3 - x2 - 2x)
then find the value of (x5 - 2x4 - x3 - x2 - 2x)
There is a father, a mother and 2 sons in a family and their total age is 60 years. The difference between the sons' age is 3 years, mother's age exceeds the sum of the sons' age by 17 years and the difference of age of father and mother is equal to the age of the elder son. How old is the father?
The values of a and b for which 3x3 - ax2 - 74x + b is a multiple of x2 + 2x - 24 are
|
5 docs|272 tests
|


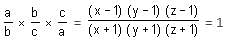
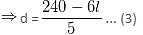
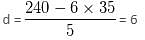
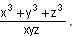
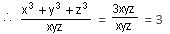
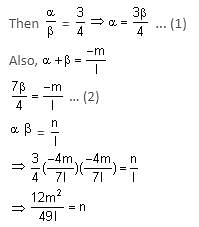
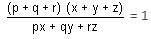
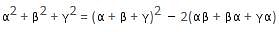
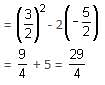
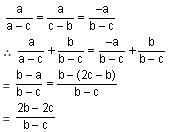
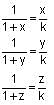
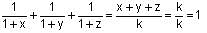
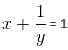 and
and 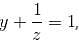 then
then 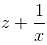 is equal to
is equal to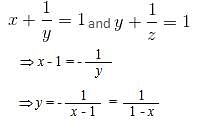
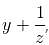 we get
we get