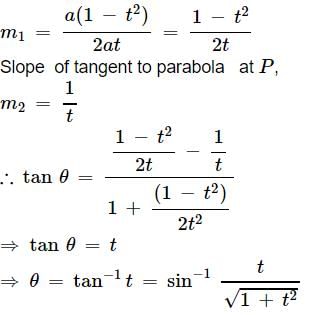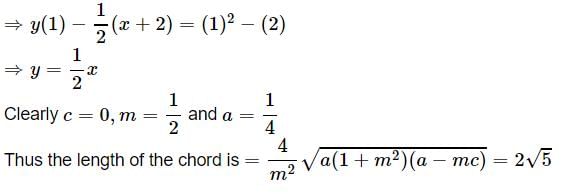Test: Parabola- 4 - Commerce MCQ
15 Questions MCQ Test Online MCQ Tests for Commerce - Test: Parabola- 4
The straight line joining any point P on the parabola y2 = 4ax to the vertex and perpendicular from the focus to the tangent at P, intersect at R, then the equation of the locus of R is
PQ is a normal chord of the parabola y2 = 4ax at P, A being the vertex of the parabola. Through P a line is drawn parallel to AQ meeting the x-axis in R. Then the length of AR is
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
If the tangents and normals at the extremities of a focal chord of a parabola intersect at (x1, y1) and (x2, y2) respectively, then
From point P two tangents are drawn from it to the parabola y2 = 4x such that the slope of one tangent is three times the slope of the other. The locus of P is
The equation of a straight line passing through the point (3, 6) and cutting the curve y = √x orthogonally is
The tangent and normal at P (t), for all real positive t, to the parabola y2 = 4ax meet the axis of the parabola in T and G respectively, then the angle at which the tangent at P to the parabola is inclined to the tangent at P to the circle through the points P, T and G is
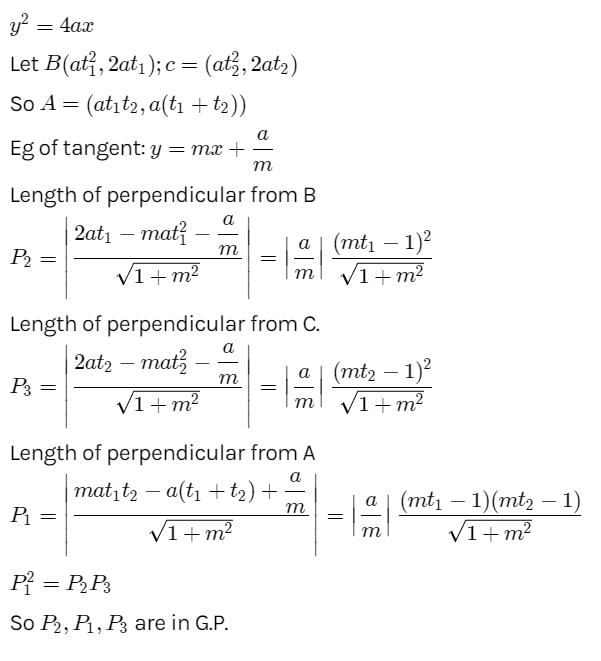
AB, AC are tangents to a parabola y2 = 4ax. p1 p2 and p3 are the lengths of the perpendiculars from A, B and C respectively on any tangent to the curve, then p2, p1, p3 are in
Through the vertex O of the parabola, y2 = 4ax two chords OP and OQ are drawn and the circles on OP and OQ as diameter intersect in R. If q1, q2 and f are the angles made with the axis by the tangent at P and Q on the parabola and by OR then the value of cotq1 + cotq2 equals
Let A be the vertex and L the length of the latus rectum of parabola, y2 – 2y – 4x – 7 = 0. The equation of the parabola with point A as vertex, 2L as the length of the latus rectum and the axis at right angles to that of the given curve is
Tangent to the parabola y2 = 4ax at point P meets the tangents at vertex A at point B and the axis of parabola at T, Q is any point on this tangent and N as the foot of perpendicular from Q on SP, where S is focus, M is the foot of perpendicular from Q on the directrix then
The parametric coordinates of any point on the parabola y2 = 4ax can be
The length of the chord of the parabola y2 = x which is bisected at the point (2, 1) is less than
The locus of the mid point of the focal radii of a variable point moving on the parabola, y2 = 4ax is a parabola whose
A variable circle is described to passes through the point (1, 0) and tangent to the curve y = tan(tan-1x). The locus of the centre of the circle is a parabola whose
Two parabolas have the same focus. If their directrices are the x-axis & the y-axis respectively, then the slope of their common chord is
|
705 tests
|
Important Questions for Parabola- 4
Parabola- 4 MCQs with Answers
Online Tests for Parabola- 4 Online MCQ Tests for Commerce
|
© EduRev
|
Education Revolution
|
|
