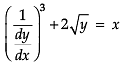All Courses
Get the App Signup / Login
Sign in Open App
JEE Exam  >  JEE Tests  >  Mathematics (Maths) Class 12  >  Test: Differential Equations- Assertion & Reason Type Questions - JEE MCQ

Test: Differential Equations- Assertion & Reason Type Questions - JEE MCQ


Test Description

6 Questions MCQ Test Mathematics (Maths) Class 12 - Test: Differential Equations- Assertion & Reason Type Questions

Test: Differential Equations- Assertion & Reason Type Questions for JEE 2024 is part of Mathematics (Maths) Class 12 preparation. The Test: Differential Equations- Assertion & Reason Type Questions questions and answers have been prepared according to the JEE exam syllabus.The Test: Differential Equations- Assertion & Reason Type Questions MCQs are made for JEE 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Differential Equations- Assertion & Reason Type Questions below.
Solutions of Test: Differential Equations- Assertion & Reason Type Questions questions in English are available as part of our Mathematics (Maths) Class 12 for JEE & Test: Differential Equations- Assertion & Reason Type Questions solutions in Hindi for Mathematics (Maths) Class 12 course. Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free. Attempt Test: Differential Equations- Assertion & Reason Type Questions | 6 questions in 12 minutes | Mock test for JEE preparation | Free important questions MCQ to study Mathematics (Maths) Class 12 for JEE Exam | Download free PDF with solutions
Test: Differential Equations- Assertion & Reason Type Questions - Question 1
Save

Direction: In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as

Assertion (A): The differential equation formed by eliminating a and b from

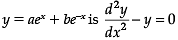
Reason (R):y = aex + be-x ….(i)Differentiating w. r. t. 'x'

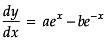
Differentiating again w .r .t .'x'

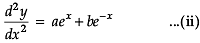
Subtracting eqn. (i) from eqn. (ii)

= 0

Test: Differential Equations- Assertion & Reason Type Questions - Question 2
Save

Direction: In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as

Assertion (A): The solution of differential equation

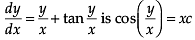
Reason (R): 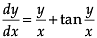we can clearly see that it is an homogeneous equation substituting

y = vx

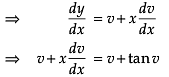
separating the variables and integrating we get

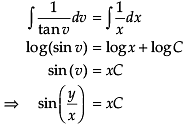
is the solution where, C is constant.

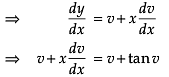
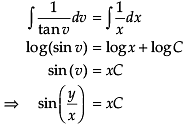
1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Differential Equations- Assertion & Reason Type Questions - Question 3
Save

Direction: In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as

Assertion (A): The degree of the differential equation given by

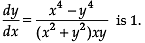
Reason (R): The degree of a differential equation is the degree of the highest order derivative when differential coefficients are free from radicals and fraction.

The given differential equation has first order derivative which is free from radical and fraction with power = 1, thus it has a degree of 1.

Test: Differential Equations- Assertion & Reason Type Questions - Question 4
Save

Direction: In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as

Assertion (A): The order and degree of the differential equation are 2 and 1

respectively

Reason (R): The differential equation

is of order 1 and degree 3.
Detailed Solution for Test: Differential Equations- Assertion & Reason Type Questions - Question 4
Squaring both sides of the given differential equation,

⇒

The highest order is 2 and its power is 1

∴ Order is 2, degree is 1

Hence, Assertion (A) is true.

The equation given in reason (R) is,

Highest order is 1 and its power is 3

∴ Order is 1 and degree is 3.

Hence, reason (R) is also true.

Test: Differential Equations- Assertion & Reason Type Questions - Question 5
Save

Direction: In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as

Assertion (A): Solution of the differential equation

Reason (R):

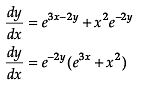
separating the variables
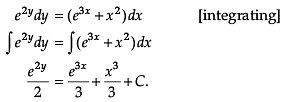
Test: Differential Equations- Assertion & Reason Type Questions - Question 6
Save

Direction: In the following questions, A statement of Assertion (A) is followed by a statement of Reason (R). Mark the correct choice as

Assertion (A): The order of the differential equation given by

Reason (R): Since the order of a differential equation is defined as the order of the highest derivative occurring in the differential equation, i.e., for nth derivative dny/dxn if n = 1. then it’s order = 1.

Given differential equation contains only dy/dx derivative with variables and constants.
204 videos|290 docs|139 tests
Join Course
Information about Test: Differential Equations- Assertion & Reason Type Questions Page
In this test you can find the Exam questions for Test: Differential Equations- Assertion & Reason Type Questions solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Differential Equations- Assertion & Reason Type Questions, EduRev gives you an ample number of Online tests for practice

Top Courses for JEE

204 videos|290 docs|139 tests
Join Course
Download as PDF

Top Courses for JEE

Important Questions for Differential Equations- Assertion & Reason Type Questions

Find all the important questions for Differential Equations- Assertion & Reason Type Questions at EduRev.Get fully prepared for Differential Equations- Assertion & Reason Type Questions with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Differential Equations- Assertion & Reason Type Questions syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Differential Equations- Assertion & Reason Type Questions resources.

Differential Equations- Assertion & Reason Type Questions MCQs with Answers

Prepare for the Differential Equations- Assertion & Reason Type Questions within the JEE exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Differential Equations- Assertion & Reason Type Questions Mathematics (Maths) Class 12

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Differential Equations- Assertion & Reason Type Questions with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Follow Us
Signup to see your scores go up
within 7 days!
Takes less than 10 seconds to signup