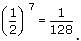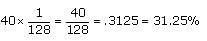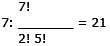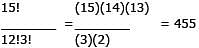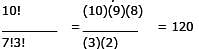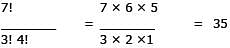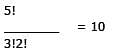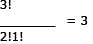Test: Probability for GMAT - 2 - GMAT MCQ
15 Questions MCQ Test Quantitative for GMAT - Test: Probability for GMAT - 2
A small, experimental plane has t hree engines, one of which is redundant. That is, as long as two of the engines are working, the plane will stay in the air. Over the course of a typical flight, there is a 1/3 chance that engine one will fail. There is a 75% probability that engine two will work. The third engine works only half the time. What is the probability that the plane will crash in any given flight?
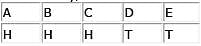
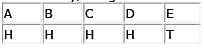
Ms. Barton has four children. You are told correctly that she has at least two girls but you are not told which two of her four children are those girls. What is the probability that she also has two boys? (Assume that the probability of having a boy is the same as the probability of having a girl.)
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Laura has a deck of standard playing cards with 13 of the 52 cards designated as a "heart." If Laura shuffles the deck thoroughly and then deals 10 cards off the top of the deck, what is the probability that the 10th card dealt is a heart?
A license plate in the country Kerrania consists of four digits followed by two letters. The letters A, B, and C are used only by government vehicles while the letters D through Z are used by non-government vehicles. Kerrania's intelligence agency has recently captured a message from the country Gonzalia indicating that an electronic transmitter has been installed in a Kerrania government vehicle with a license plate starting with 79. If it takes the police 10 minutes to inspect each vehicle, what is the probability that the police will find the transmitter within three hours?
Baseball's World Series matches 2 teams against each other in a best-of-seven series. The first team to win four games wins the series and no subsequent games are played. If you have no special information about either of the teams, what is the probability that the World Series will consist of fewer than 7 games?
Harriet and Tran each have $10. Together, they flip a fair coin 5 times. Every time the coin lands on heads, Tran gives Harriet $1. Every time the coin lands on tails, Harriet gives Tran $1. After the five coin flips, what is the probability that Harriet has more than $10 but less than $15?
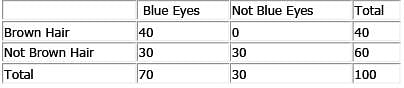
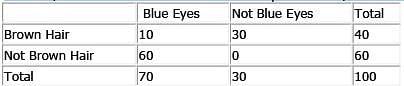
If 40 percent of all students at College X have brown hair and 70 percent of all students at College X have blue eyes, what is the difference between the minimum and the maximum probability of picking a student from College X who has neither brown hair nor blue eyes?
In a room filled with 7 people, 4 people have exactly 1 friend in the room and 3 people have exactly 2 friends in the room (Assuming that friendship is a mutual relationship, i.e. if John is Peter's friend, Peter is John's friend). If two individuals are selected from the room at random, what is the probability that those two individuals are NOT friends?
Bill has a small deck of 12 pl aying cards made up of only 2 suits of 6 cards each. Each of the 6 cards within a suit has a different value from 1 to 6; thus, there are 2 cards in the deck that have the same value. Bill likes to play a game in which he shuffles the deck, turns over 4 cards, and looks for pairs of cards that have the same value. What is the chance that Bill finds at least one pair of cards that have the same value?
If a jury of 12 people is to be selected randomly from a pool of 15 potential jurors, and the jury pool consists of 2/3 men and 1/3 women, what is the probability that the jury will comprise at least 2/3 men?
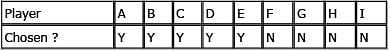
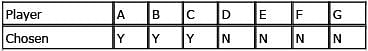
John and Peter are among the nine pl ayers a basketball coach can choose from to field a five-player team. If all five players are chosen at random, what is the probability of choosing a team that includes John and Peter?
A small company employs 3 men and 5 women. If a team of 4 employees is to be randomly selected to organize the company retreat, what is the probability that the team will have exactly 2 women?
A box contains one dozen donuts. Four of the donuts are chocolate, four are glazed, and four are jelly. If two donuts are randomly selected from the box, one after the other, what is the probability that both will be jelly donuts?
A hand purse contains 6 nickels, 5 pennies and 4 dimes. What is the probability of picking a coin other than a nickel twice in a row if the first coin picked is not put back?
Jim and Renee will play one game of Rock, Paper, Scissors. In this game, each will select and show a hand sign for one of the three items. Rock beats Scissors, Scissors beat Paper, and Paper beats Rock. Assuming that both Jim and Renee have an equal chance of choosing any one of the hand signs, what is the probability that Jim will win?
|
108 videos|103 docs|114 tests
|
|
108 videos|103 docs|114 tests
|


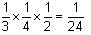

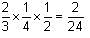
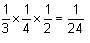
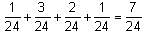
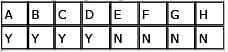
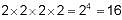 possible ways that the children might be born, as listed below: BBBB (all boys) BBBG, BBGB, BGBB, GBBB, (3 boys, 1 girl) BBGG, BGGB, BGBG, GGBB, GBBG, GBGB (2 boys, 2 girls) GGGB, GGBG, GBGG, BGGG (3 girls, 1 boy) GGGG (all girls) Since we are told that there are at least 2 girls, we can eliminate 5 possibilities--the one possibility in which all of the children are boys (the first row) and the four possibilities in which only one of the children is a girl (the second row).
possible ways that the children might be born, as listed below: BBBB (all boys) BBBG, BBGB, BGBB, GBBB, (3 boys, 1 girl) BBGG, BGGB, BGBG, GGBB, GBBG, GBGB (2 boys, 2 girls) GGGB, GGBG, GBGG, BGGG (3 girls, 1 boy) GGGG (all girls) Since we are told that there are at least 2 girls, we can eliminate 5 possibilities--the one possibility in which all of the children are boys (the first row) and the four possibilities in which only one of the children is a girl (the second row).