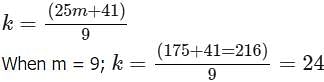Test: Divisibility/Multiples/Factors - GMAT MCQ
10 Questions MCQ Test Practice Questions for GMAT - Test: Divisibility/Multiples/Factors
If a and b are both factors of 50, which of the following must be true?
Set A consists of three consecutive positive multiples of 3, and set B consists of five consecutive positive multiples of 5. If the sum of the integers in set A is equal to the sum of the integers in set B, what is the least number that could be a member of set A?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
If c and d are positive integers and m is the greatest common factor of c and d, then m must be the greatest common factor of c and which of the following integers?
A department of motor vehicles asks visitors to draw numbered tickets from a dispenser so that they can be served in order by number. Six friends have graduated from truck-driving school and go to the department to get commercial driving licenses. They draw tickets and find that their numbers are a set of evenly spaced integers with a range of 10. Which of the following could NOT be the sum of their numbers?
How many even integers between 100 and 200, inclusive, are divisible by 7?
What is the largest 3 digit number to have an odd number of factors?
x and y are integers less than 60 such that x is equal to the sum of the squares of two distinct prime numbers, and y is a multiple of 17. Which of the following could be the value of x – y?
What is the least positive value that can be subtracted from 22050 so that the result is a multiple of 17?
Both a and b are perfect squares, and the product a×b is divisible by 10 as well as 15. By which of the following the product a×b may NOT be divisible?
If x and y are positive integers, each of the following could be the greatest common divisor of 30x and 15y EXCEPT
|
18 docs|139 tests
|