Mathematics Test - 3 - SAT MCQ
30 Questions MCQ Test Digital SAT Mock Test Series 2024 - Mathematics Test - 3
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
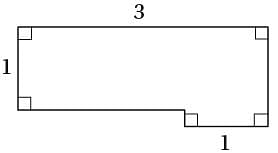
If the area of the figure above is 16/5 square units, what is its perimeter?

If the area of the figure above is 16/5 square units, what is its perimeter?
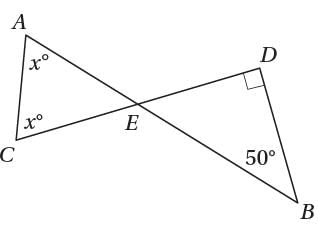
In the figure above, line segments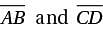 intersect at point E. What is the value of x ?
intersect at point E. What is the value of x ?
Everyone in Niko’s class has a different birth date. If Niko is both the 8th oldest person and the 12th youngest person in his class, how many students are in Niko’s class?
The functions f, g, and h are defined by the equations f (x) = x2, g (x) = x, and h(x) = √x. Which of the following must be true?
If the function m(x) satisfies the equation 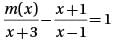 for all values of x greater than 1, then m(x) =
for all values of x greater than 1, then m(x) =
If k > 2, which of the following could be the graph of y + x = k(x - 1) in the x y-plane?
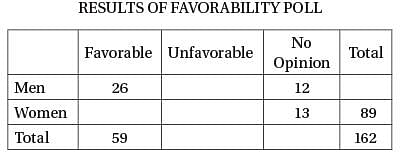
The table above shows the partial results of a favorability poll for a local politician. If the data shown are correct, how many of the women who were polled viewed the politician unfavorably?
Question based on the graph below.
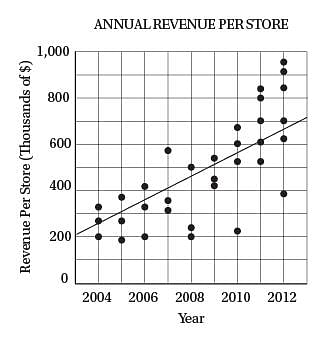
The scatterplot above shows the annual revenue for each of the individual retail stores operated by a clothing company for each year from 2004 through 2012. Based on the line of best fit to the data shown, which of the following is closest to the average annual increase in revenue per store?
A culture of bacteria initially contained p cells, where p > 100. After one hour, this population decreased by 1/3. In the second and third hours, however, the population increased by 40% and 50%, respectively. At the end of those first three hours, what was the population of the culture?
An online trading company charges a 3% commission for all stock purchases. If a trader purchases 200 shares of a stock through this company and is charged $3,399 including commission, what is the cost per share for this stock?
In the xy-plane, the parabola with equation y = (x − 11)2 intersects the line with equation y = 25 at two points, A and B. What is the length of 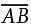 ?
?
C = 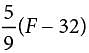
The equation above shows how a temperature F, measured in degrees Fahrenheit, relates to a temperature C, measured in degrees Celsius. Based on the equation, which of the following must be true?
I. A temperature increase of 1 degree Fahrenheit is equivalent to a temperature increase of 5/9 degree Celsius.
II. A temperature increase of 1 degree Celsius is equivalent to a temperature increase of 1.8 degrees Fahrenheit.
III. A temperature increase of 5/9 degree Fahrenheit is equivalent to a temperature increase of 1 degree Celsius.
In triangle ABC, the measure of ∠B is 90° , BC = 16 , and AC = 20. Triangle DEF is similar to triangle ABC, where vertices D, E, and F correspond to vertices A, B, and C, respectively, and each side of triangle DEF is 1/3
the length of the corresponding side of triangle ABC. What is the value of sin F?
Question refer to the following information.
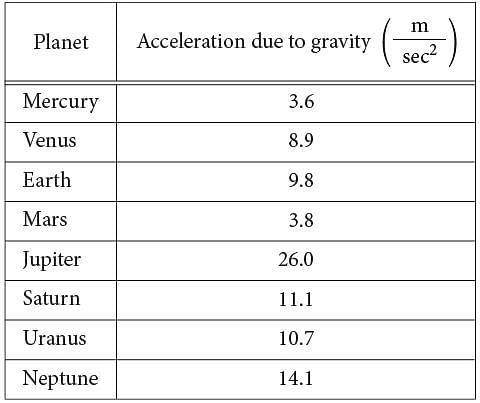
The chart above shows approximations of the acceleration due to gravity in meters per second squared 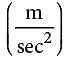 for the eight planets in our solar system. The weight of an object on a given planet can be found by using the formula W =mg , where W is the weight of the object measured in newtons, m is the mass of the object measured in kilograms, and g is the acceleration due to gravity on the planet measured in m/sec2.
for the eight planets in our solar system. The weight of an object on a given planet can be found by using the formula W =mg , where W is the weight of the object measured in newtons, m is the mass of the object measured in kilograms, and g is the acceleration due to gravity on the planet measured in m/sec2.
Q. What is the weight, in newtons, of an object on Mercury with a mass of 90 kilograms?
In order to determine if treatment X is successful in improving eyesight, a research study was conducted.From a large population of people with poor eyesight, 300 participants were selected at random.Half of the participants were randomly assigned to receive treatment X, and the other half did not receive treatment X. The resulting data showed that participants who received treatment X had significantly improved eyesight as compared to those who did not receive treatment X. Based on the design and results of the study, which of the following is an appropriate conclusion?
Which of the following is equivalent to the sum of the expressions a2 −1 and a + 1?
Maria plans to rent a boat. The boat rental costs $60 per hour, and she will also have to pay for a water safety course that costs $10. Maria wants to spend no more than $280 for the rental and the course. If the boat rental is available only for a whole number of hours, what is the maximum number of hours for which Maria can rent the boat?
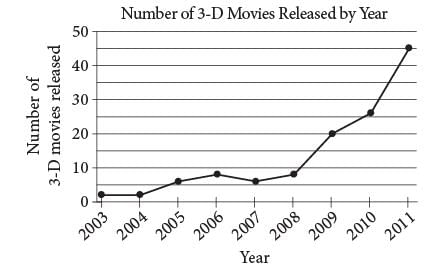
According to the line graph above, between which two consecutive years was there the greatest change in the number of 3‑D movies released?
An online bookstore sells novels and magazines.
Each novel sells for $4, and each magazine sells for $1. If Sadie purchased a total of 11 novels and magazines that have a combined selling price of $20, how many novels did she purchase?
−2x + 3y = 6
In the xy-plane, the graph of which of the following equations is perpendicular to the graph of the equation above?
x = y - 3
x/2 + 2y = 6
Which ordered pair (x, y) satisfies the system of equations shown above?
x2 + 6x + 4
Which of the following is equivalent to the expression above?
The function f is defined by f(x) = (x+ 3)(x + 1).The graph of f in the xy-plane is a parabola. Which of the following intervals contains the x-coordinate of the vertex of the graph of f ?
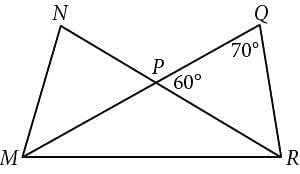
In the figure above, 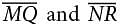 intersect at point P, NP = QP , and MP = PR. What is the measure, in degrees, of ∠ QMR ? (Disregard the degree symbol when gridding your answer.)
intersect at point P, NP = QP , and MP = PR. What is the measure, in degrees, of ∠ QMR ? (Disregard the degree symbol when gridding your answer.)
Question refer to the following information.
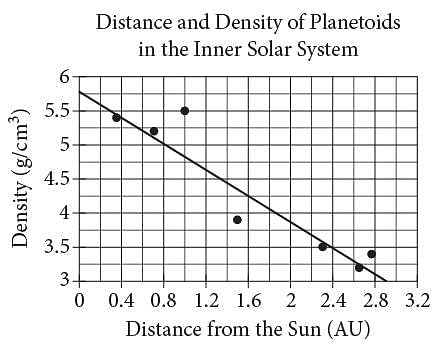
The scatterplot above shows the densities of 7 planetoids, in grams per cubic centimeter, with respect to their average distances from the Sun in astronomical units (AU). The line of best fit is also shown.
Q. According to the scatterplot, which of the following statements is true about the relationship between a planetoid’s average distance from the Sun and its density?
A customer paid $53.00 for a jacket after a 6 percent sales tax was added. What was the price of the jacket before the sales tax was added?
y = 19.99 + 1.50x
The equation above models the total cost y,in dollars, that a company charges a customer to rent a truck for one day and drive the truck x miles. The total cost consists of a flat fee plus a charge per mile driven. When the equation is graphed in the xy-plane, what does the y-intercept of the graph represent in terms of the model?
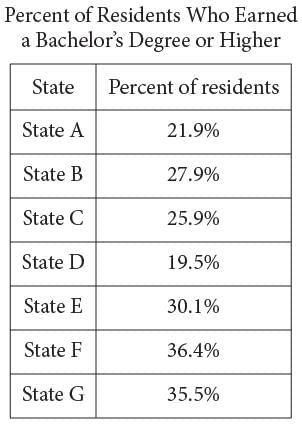
A survey was given to residents of all 50 states asking if they had earned a bachelor’s degree or higher.The results from 7 of the states are given in the table above. The median percent of residents who earned a bachelor’s degree or higher for all 50 states was 26.95%. What is the difference between the median percent of residents who earned a bachelor’s degree or higher for these 7 states and the median for all 50 states?
|
8 docs|15 tests
|


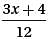 ?
?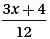
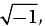 which of t he follow ing is NOT equal to i3 + i ?
which of t he follow ing is NOT equal to i3 + i ?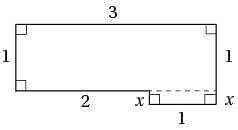
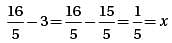
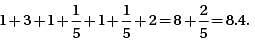
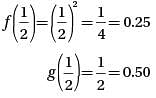
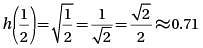
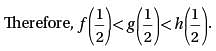
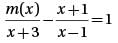
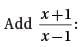
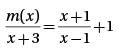
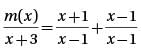
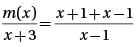
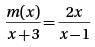
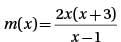
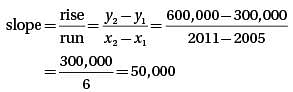
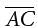 is the hypotenuse of right triangle ABC, and
is the hypotenuse of right triangle ABC, and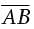 and
and 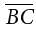 are the legs of right triangle ABC. By the Pythagorean theorem,
are the legs of right triangle ABC. By the Pythagorean theorem, 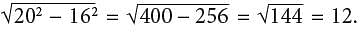 Since triangle DEF is similar to triangle ABC, with vertex F corresponding to vertex C, the measure of angle F equals the measure of angle C. Thus, sinF = sinC. From the side lengths of triangle ABC, sinC = opposite side/hypotenuse = AB/AC = 12/20 = 3/5 . Therefore, sinF = 3/5. Either 3/5 or its decimal equivalent, .6, may be gridded as the correct answer.
Since triangle DEF is similar to triangle ABC, with vertex F corresponding to vertex C, the measure of angle F equals the measure of angle C. Thus, sinF = sinC. From the side lengths of triangle ABC, sinC = opposite side/hypotenuse = AB/AC = 12/20 = 3/5 . Therefore, sinF = 3/5. Either 3/5 or its decimal equivalent, .6, may be gridded as the correct answer.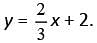 So the slope of the graph of the given equation is 2/3. In the xy-plane, when two nonvertical lines are perpendicular, the product of their slopes is −1. So, if m is the slope of a line perpendicular to the line with equation
So the slope of the graph of the given equation is 2/3. In the xy-plane, when two nonvertical lines are perpendicular, the product of their slopes is −1. So, if m is the slope of a line perpendicular to the line with equation  which yields m =
which yields m = 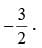 Of the given choices, only the equation in choice A can be rewritten in the form
Of the given choices, only the equation in choice A can be rewritten in the form 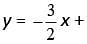 b , for some constant b. Therefore, the graph of the equation in choice A is perpendicular to the graph of the given equation.
b , for some constant b. Therefore, the graph of the equation in choice A is perpendicular to the graph of the given equation.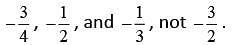
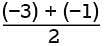 = –2. Of the shown intervals, only the interval in choice B contains –2.
= –2. Of the shown intervals, only the interval in choice B contains –2.














