Practice Test: Quadratic Equations - Class 10 MCQ
25 Questions MCQ Test Mathematics (Maths) Class 10 - Practice Test: Quadratic Equations
Which of the following quadratic expression can be expressed as a product of real linear factors?
Two candidates attempt to solve a quadratic equation of the form x2 + px + q = 0. One starts with a wrong value of p and finds the roots to be 2 and 6. The other starts with a wrong value of q and finds the roots to be 2 and – 9. Find the correct roots of the equation :
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Determine k such that the quadratic equation x2 + 7(3 + 2k) – 2x (1 + 3k) = 0 has equal roots :
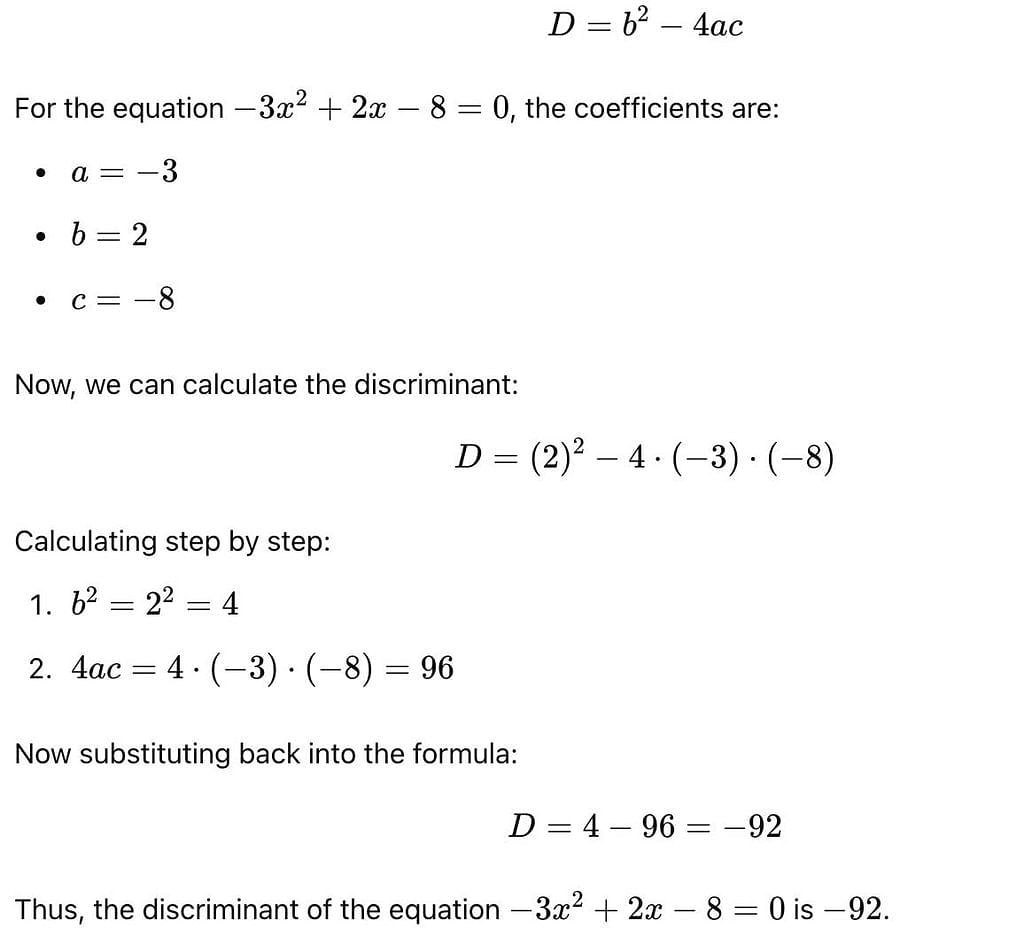
Discriminant of the equation: ( – 3x2 + 2x – 8 = 0 ) is
The nature of the roots of the equation x2 – 5x + 7 = 0 is –
The equation x2 – px + q = 0 p, q ε R has no real roots if :
Determine the value of k for which the quadratic equation 4x2 – 3kx + 1 = 0 has equal roots :
Find the value of k such that the sum of the squares of the roots of the quadratic equation x2 – 8x + k = 0 is 40:
Find the value of p for which the quadratic equation x2 + p(4x + p – 1) + 2 = 0 has equal roots :
The length of a hypotenuse of a right triangle exceeds the length of its base by 2 cm and exceeds twice the length of the altitude by 1 cm. Find the length of each side of the triangle (in cm) :
A two digit number is such that the product of it's digits is 12. When 9 is added to the number, the digits interchange their places, find the number :
A plane left 40 minutes late due to bad weather and in order to reach it's destination, 1600 km away in time,it had to increase it's speed by 400 km/h from it's usual speed. Find the usual speed of the plane :
The sum of the squares of two consecutive positive odd numbers is 290. Find the sum of the numbers :
A shopkeeper buys a number of books for Rs. 80. If he had bought 4 more for the same amount, each book would have cost Re. 1 less. How many books did he buy?
Two squares have sides x cm and (x + 4) cm. The sum of their areas is 656 cm2. Find the sides of the square.
The real values of a for which the quadratic equation 2x2 – (a3 + 8a – 1) x + a2 – 4a = 0 possesses roots of opposite signs are given by :
The number of real solutions of the equation is :
If the equation (3x)2 + (27 × 31/k – 15) x + 4 = 0 has equal roots, then k =
Equation ax2 + 2x + 1 has one double root if :
Solve for x : (x + 2) (x – 5) (x – 6) (x + 1) = 144 :
|
123 videos|457 docs|77 tests
|
|
123 videos|457 docs|77 tests
|


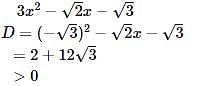
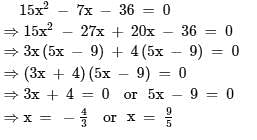
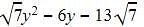
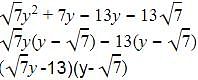
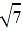
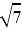
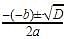 , Since we do not have any real number which is a root of a negative number, the roots are not real.
, Since we do not have any real number which is a root of a negative number, the roots are not real.