Test: Circles- Case Based Type Questions - EmSAT Achieve MCQ
12 Questions MCQ Test Mathematics for EmSAT Achieve - Test: Circles- Case Based Type Questions
Rohan draws a circle of radius 10 cm with the help of compass and scale. He also draws two chords, AB and CD in such a way that AB and CD are 6 cm and 8 cm from the centre O. Now, he has some doubts that are given below. Help him out by answering these questions:
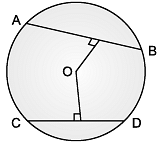
Q. A quadrilateral is called cyclic if all the four vertices of it lie on a _________

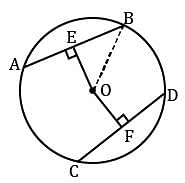
In a circle of radius 10 cm given below, chord AB and CD are equal. If OE bisects AB and OF bisects CD and OF = 6 cm, then length EB is ________ .

| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Rohan draws a circle of radius 10 cm with the help of compass and scale. He also draws two chords, AB and CD in such a way that AB and CD are 6 cm and 8 cm from the centre O. Now, he has some doubts that are given below. Help him out by answering these questions:
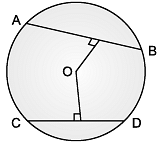
Q. What is the length of AB?

Rohan draws a circle of radius 10 cm with the help of compass and scale. He also draws two chords, AB and CD in such a way that AB and CD are 6 cm and 8 cm from the centre O. Now, he has some doubts that are given below. Help him out by answering these questions:
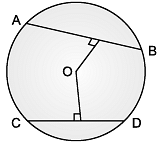
Q. A circle divides the plane, on which it lies, in _________ parts.
Rohan draws a circle of radius 10 cm with the help of compass and scale. He also draws two chords, AB and CD in such a way that AB and CD are 6 cm and 8 cm from the centre O. Now, he has some doubts that are given below. Help him out by answering these questions:
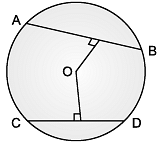
Q. Which statement is not true?
A Ferris wheel (or a big wheel in the United Kingdom) is an amusement ride consisting of a rotating upright wheel with multiple passenger-carrying components (commonly referred to as passenger cars, cabins, tubs, capsules, gondolas, or pods) attached to the rim in such a way that as the wheel turns, they are kept upright, usually by gravity.
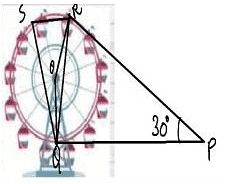
After taking a ride in Ferris wheel, Aarti came out from the crowd and was observing her friends who were enjoying the ride . She was curious about the different angles and measures that the wheel will form. She forms the figure as given below.
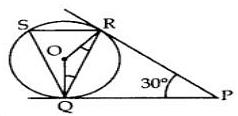
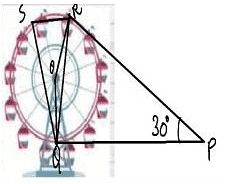
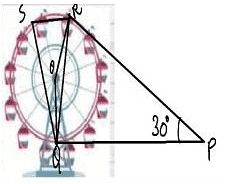
Q. Find ∠ORP
A Ferris wheel (or a big wheel in the United Kingdom) is an amusement ride consisting of a rotating upright wheel with multiple passenger-carrying components (commonly referred to as passenger cars, cabins, tubs, capsules, gondolas, or pods) attached to the rim in such a way that as the wheel turns, they are kept upright, usually by gravity.
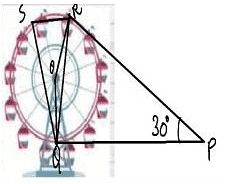
After taking a ride in Ferris wheel, Aarti came out from the crowd and was observing her friends who were enjoying the ride . She was curious about the different angles and measures that the wheel will form. She forms the figure as given below.
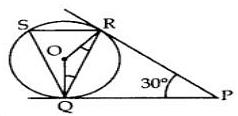
Q. Find ∠RQP
A Ferris wheel (or a big wheel in the United Kingdom) is an amusement ride consisting of a rotating upright wheel with multiple passenger-carrying components (commonly referred to as passenger cars, cabins, tubs, capsules, gondolas, or pods) attached to the rim in such a way that as the wheel turns, they are kept upright, usually by gravity.
After taking a ride in Ferris wheel, Aarti came out from the crowd and was observing her friends who were enjoying the ride . She was curious about the different angles and measures that the wheel will form. She forms the figure as given below.
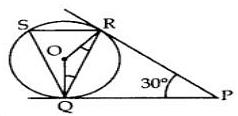
Q. In the given figure find ∠ROQ
A Ferris wheel (or a big wheel in the United Kingdom) is an amusement ride consisting of a rotating upright wheel with multiple passenger-carrying components (commonly referred to as passenger cars, cabins, tubs, capsules, gondolas, or pods) attached to the rim in such a way that as the wheel turns, they are kept upright, usually by gravity.
After taking a ride in Ferris wheel, Aarti came out from the crowd and was observing her friends who were enjoying the ride . She was curious about the different angles and measures that the wheel will form. She forms the figure as given below.
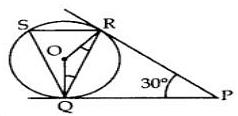
Q. Find ∠RSQ

Varun has been selected by his School to design logo for Sports Day T-shirts for students and staff . The logo design is as given in the figure and he is working on the fonts and different colours according to the theme. In given figure, a circle with centre O is inscribed in a ΔABC, such that it touches the sides AB, BC and CA at points D, E and F respectively. The lengths of sides AB, BC and CA are 12 cm, 8 cm and 10 cm respectively.
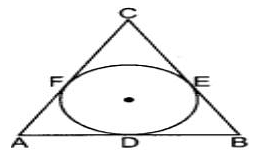
Q. If radius of the circle is 4cm, Find the area of ∆OAB

Varun has been selected by his School to design logo for Sports Day T-shirts for students and staff . The logo design is as given in the figure and he is working on the fonts and different colours according to the theme. In given figure, a circle with centre O is inscribed in a ΔABC, such that it touches the sides AB, BC and CA at points D, E and F respectively. The lengths of sides AB, BC and CA are 12 cm, 8 cm and 10 cm respectively.
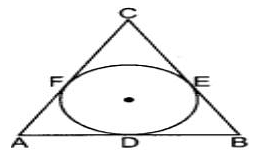
Q. Find the Length of BE

Varun has been selected by his School to design logo for Sports Day T-shirts for students and staff . The logo design is as given in the figure and he is working on the fonts and different colours according to the theme. In given figure, a circle with centre O is inscribed in a ΔABC, such that it touches the sides AB, BC and CA at points D, E and F respectively. The lengths of sides AB, BC and CA are 12 cm, 8 cm and 10 cm respectively.
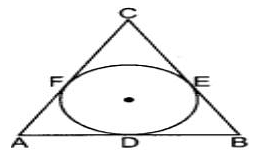
Q. Find area of ∆ABC
|
141 videos|213 docs|254 tests
|
|
141 videos|213 docs|254 tests
|

















