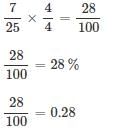Irrational Numbers - Number System - Class 9 MCQ
25 Questions MCQ Test Mathematics (Maths) Class 9 - Irrational Numbers - Number System
A number is irrational if and only if its decimal representation is:
The product or quotient of a non-zero rational number with an irrational number is:
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Which of the following statement about real numbers is false ?
The decimal expansion 0.080080008000080000080000008……. is a
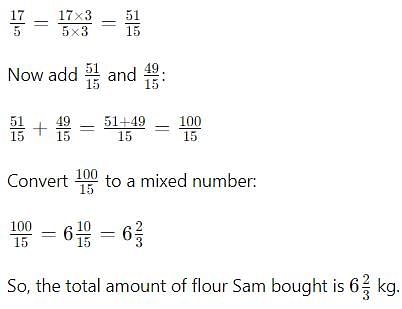
Sam bought 2+7⁄5 kg of flour in one week and 3+4⁄15 kg of flour in second week. The flour Sam bough altogether is
The product of two numbers is -20/9. If one of the numbers is 4, find the other.
Which of the following is an irrational number ?
The ratio of the circumference of a circle to the diameter of the circle is.
Of the given numbers
(i) √23
(ii) √256
(iii) 0.3796
(iv) 7.478478…
(v) 1.101001000100001…
Which among these is the approximate value of π?
The decimal expansion 0.080080008000080000080000008….. is a
How many rational numbers can you find between 5 and 6?
From the choices given below mark the co-prime numbers
|
44 videos|412 docs|54 tests
|
|
44 videos|412 docs|54 tests
|


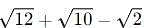 involves the square roots of non-perfect squares (12, 10, and 2), which are irrational numbers.
involves the square roots of non-perfect squares (12, 10, and 2), which are irrational numbers.
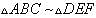 ∠A = 50° ∠C = 35° ∠E = ?
∠A = 50° ∠C = 35° ∠E = ?