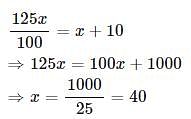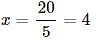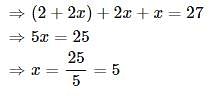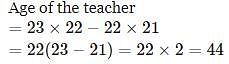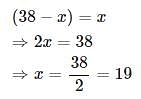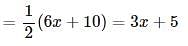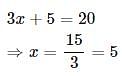Test: Problems On Ages - 1 - CUET Commerce MCQ
10 Questions MCQ Test General Test Preparation for CUET - Test: Problems On Ages - 1
Ten years ago, P was half of Q's age. If the ratio of their present ages is 3:4, what will be the total of their present ages?
Father is aged three times more than his son Sunil. After 8 years, he would be two and a half times of Sunil's age. After further 8 years, how many times would he be of Sunil's age?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
A man's age is 125%of what it was 10 years ago, but 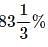 of what it will be after 10years. What is his present age?
of what it will be after 10years. What is his present age?
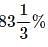 of what it will be after 10years. What is his present age?
of what it will be after 10years. What is his present age?A man is 24 years older than his son. In two years, his age will be twice the age of his son. What is the present age of his son?
Present ages of Kiran and Syam are in the ratio of 5 : 4 respectively. Three years hence, the ratio of their ages will become 11 : 9 respectively. What is Syam's present age in years?
The sum of ages of 5 children born at the intervals of 3 years each is 50 years. Find out the age of the youngest child?
A is two years older than B who is twice as old as C. The total of the ages of A, B and C is 27 . How old is B?
The average age of a class of 22 students is 21 years. The average increased by 1 when the teacher's age also included. What is the age of the teacher?
A father said to his son, "I was as old as you are at the present at the time of your birth". If the father's age is 38 years now, what was the son's age five years back?
Ayisha's age is 1 / 6 th of her father's age. Ayisha's father's age will be twice Shankar's age after 10 years. If Shankar's eight birthdays was celebrated two years before, then what is Ayisha's present age.
|
154 videos|396 docs|723 tests
|
|
154 videos|396 docs|723 tests
|


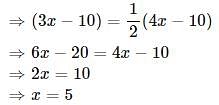
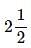 times of Sunils' age
times of Sunils' age