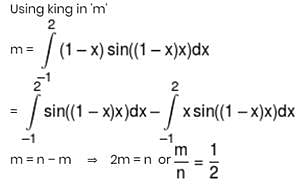JEE Main Maths Mock Test- 8 - JEE MCQ
25 Questions MCQ Test Mock Tests for JEE Main and Advanced 2025 - JEE Main Maths Mock Test- 8
In the following question, a Statement of Assertion (A) is given followed by a corresponding Reason (R) just below it. Read the Statements carefully and mark the correct answer-
Assertion(A) :If a 2 x 2 matrix commutes with every 2 x 2 matrix, then it is a scalar matrix.
Reason(R) :A 2 x 2 scalar matrix commutes with every 2 x 2 matrix.
Assertion(A) :If a 2 x 2 matrix commutes with every 2 x 2 matrix, then it is a scalar matrix.
Reason(R) :A 2 x 2 scalar matrix commutes with every 2 x 2 matrix.
In the following question, a Statement of Assertion (A) is given followed by a corresponding Reason (R) just below it. Read the Statements carefully and mark the correct answer-

| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
The area, in square unit, of the region bounded by the curve x2 = 4y , the line x = 2 and the x-axis is
The radical axis of the circles, belongs to the coaxial system of circles whose limiting points are (1,3) and (2,6) is
If the line 2x - y + k = 0 is a diameter of the circle x2 + y2 + 6x -6y + 5 =0, then k is equal to
If z₁ and z₂ are any two complex numbers then |z₁ + z₂|2 + |z₁ - z₂|2 =
The complex equation |z + 1 − i| = |z + i − 1| represents a
In the following question, a Statement of Assertion (A) is given followed by a corresponding Reason (R) just below it. Read the Statements carefully and mark the correct answer-
Assertion (A): Three vectors
Reason (R): The coplanar vectors are linearly independent
In the following question, a Statement-1 is given followed by a corresponding Statement-2 just below it. Read the statements carefully and mark the correct answer-
Consider the system of equations
x - 2y + 3z = -1
- x + y - 2z = k
x - 3y + 4z = 1
Statement-1:
The system of equations has no solution for k ≠3.
Statement-2:
The determinant
Twelve students compete for a race. The number of ways in which first three prizes can be taken is
The tangents at the points a on the parabola
are at right angles if
Points (0,0), (2,-1) and (9,2) are vertices of a triangle, then cosB =
The angle between the lines (x - 1)/1 = (y - 1)/1 = (z - 1)/2 and (x - 1)/(- √3 - 1) = (y - 1)/(√3 - 1) = (z - 1)/4 is
If function ƒ(x) = x3 + ax2 + bx + c is monotonically increasing ∀ x ∈ R, where a & b are prime numbers less than 10, then number of possible ordered pairs (a,b) is
Suppose that ƒ is differentiable for all x such that ƒ'(x) ≤ 2 for all x. If ƒ(1) = 2 and ƒ(4) = 8 then ƒ(2) has the value equal to -
Let y = g(x) be the inverse of a bijective mapping f : R → R, f(x) = 3x3 + 2x. The area bounded by graph of g(x), the x-axis and the ordinate at x = 5 is -
|
357 docs|148 tests
|
|
357 docs|148 tests
|


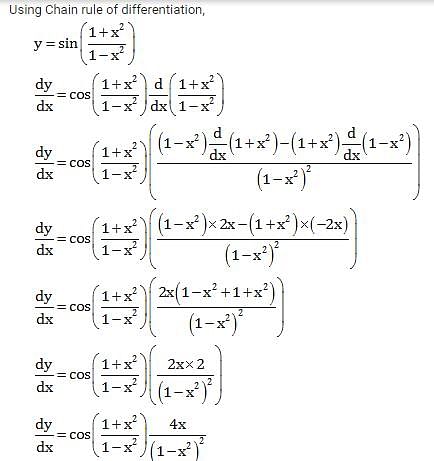

 then I1 + I2 is equal to
then I1 + I2 is equal to 
 is equal to:-
is equal to:-