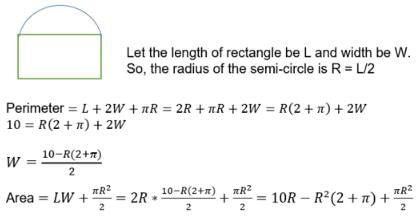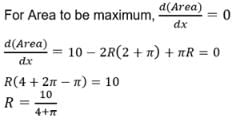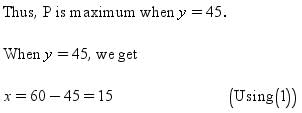Test: Maxima Minima (Derivative)- 2 - JEE MCQ
10 Questions MCQ Test Mathematics (Maths) Class 12 - Test: Maxima Minima (Derivative)- 2
A window is in the form of a rectangle surmounted by a semicircular opening. The total perimeter of the window is 10 m. Find the radius of the semicircular opening of the window to admit maximum light.
The point of local maxima for the function f(x) = sinx. cos x is
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
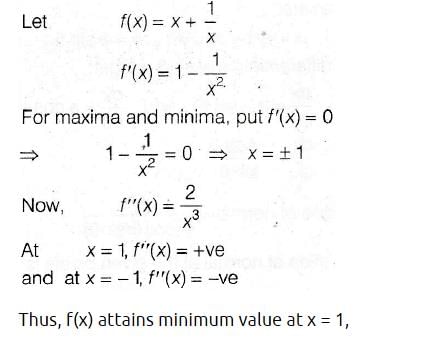
A real number x when added to its reciprocal give minimum value to the sum when x is
The sum of two positive numbers is 20. Find the numbers if their product is maximum
f(x) = x5 – 5x4 + 5x3 – 1. The local maxima of the function f(x) is at x =
How many units should be sold so that a company can make maximum profit if the profit function for x units is given by p(x) = 25 + 64x - x2
Find two numbers whose sum is 24 and product is a large as possible.
A point c in the domain of a function f is called a critical point of f if
Find two positive numbers x and y such that x + y = 60 and xy3 is maximum
|
204 videos|290 docs|139 tests
|
|
204 videos|290 docs|139 tests
|