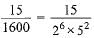RS Aggarwal Test: Real Numbers - 2 - Class 10 MCQ
15 Questions MCQ Test Mathematics (Maths) Class 10 - RS Aggarwal Test: Real Numbers - 2
If n is a Natural number, then 52n − 22n is divisible by
Two alarm clocks ring their alarms at regular intervals of 50 seconds and 48 seconds. If they first beep together at 12 noon, at what time will they beep again for the first time?
The product of three consecutive integers is divisible by
If A = 2n + 13, B = n + 7, where n is a natural number, then HCF of A and B is:
The largest number which divides 615 and 963 leaving remainder 6 in each case is
There are 576 boys and 448 girls in a school that are to be divided into equal sections of either boys or girls alone. The total number of sections thus formed are:
The largest number which divides 70 and 125 leaving remainders 5 and 8 respectively is
The HCF of 2472, 1284 and a third number N is 12. If their LCM is 23 x 32 x 5 x 103 x 107, then the number N is :
The product of a non zero rational and an irrational number is
Two natural numbers whose difference is 66 and the least common multiple is 360, are:
The decimal expansion of the rational number 14587/1250 will terminate after
Which of the following rational numbers have a terminating decimal expansion?
If two positive integers a and b are written as a = x3y2 and b = xy3 ; x, y are prime numbers, then HCF (a, b) is
The LCM of two numbers is 1200. Which of the following cannot be their HCF?
|
126 videos|457 docs|75 tests
|