Test: Functions- 1 - GMAT MCQ
15 Questions MCQ Test Quantitative for GMAT - Test: Functions- 1
If g(x) = -2x2 + 8 and g (-q) = -24, which of the following could be the value of q?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
$x = 6x +4 and £x = 8x – 2
Find the value of x for which $x = £x?
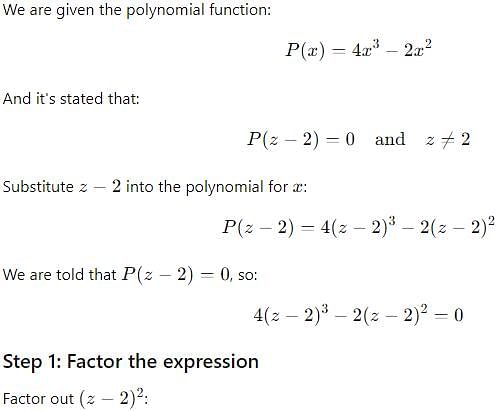
A polynomial function P(x) is defined as,
P(x) = 4x3 – 2x2
If P (z -2) =0 & z ≠ 2, find the value of z?
For which of the following functions is f(ab) = f(a) * f(b)?
- f(x) = x2
- f (x) = √x
- f (x) = 2x
If f(x) = 3x2 – 5x + 9 and g(x) = 4x – 5, then find the value of g( f(x)) at x = 3.
If v* = (1/v), find the value of
v* + (v*)* + ((v*)*)* if v = 1/2.
If # and $ are mathematical operations such that p # q = (p+ q)2 and p $ q = (p – q)2, what is the value of (2 # 2) + (3 $ 3) – (5 # 5) + (6 $ 6)?
A function is defined as f (n) = the number of factors of n. If f (p*q*r) = 8, where p, q and r are prime numbers, what is the value of p?
(1) p + q + r is an even number
(2) q < p < r
If x ≠ 0, for which of following functions is , for all values of x?
If f(x) = x + x2, is f(a+1) – f(a) divisible by 4, where a is an integer > 0
(1) f(a) is divisible by 4
(2) (-1)a < (-1)a+1
The function {x} is defined as the lowest odd integer greater than x. What is the value of {x}?
(1) -3.1 < x < -2.5
(2) x2 < 9
If and
then for positive integers (a, b) the maximum value of
is
The function SCI is defined as SCI(x, y) = z, where z is the sum of y consecutive positive integers starting from positive integer x. If a and n are positive integers, is SCI(a,n) divisible by n?
(1) 3n +2a is not divisible by 2
(2) 3a +2n is divisible by 2
|
115 videos|106 docs|113 tests
|
|
115 videos|106 docs|113 tests
|