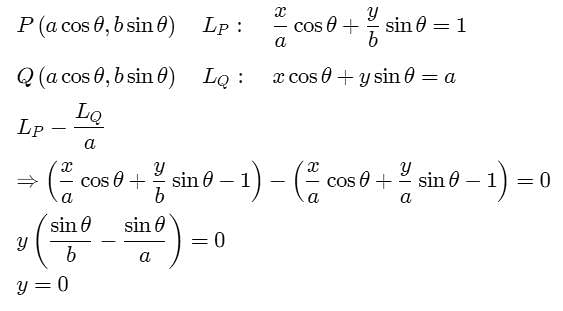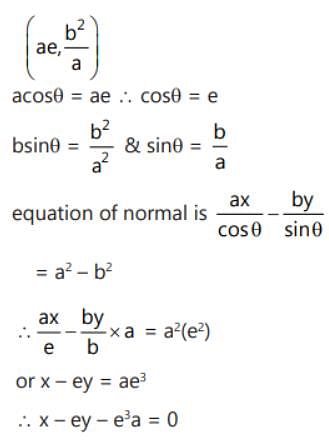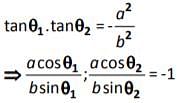All Courses
Get the App Signup / Login
Sign in Open App
JEE Exam  >  JEE Tests  >  Mathematics (Maths) for JEE Main & Advanced  >  Test: Ellipse- 2 - JEE MCQ

Test: Ellipse- 2 - JEE MCQ


Test Description

20 Questions MCQ Test Mathematics (Maths) for JEE Main & Advanced - Test: Ellipse- 2

Test: Ellipse- 2 for JEE 2024 is part of Mathematics (Maths) for JEE Main & Advanced preparation. The Test: Ellipse- 2 questions and answers have been prepared according to the JEE exam syllabus.The Test: Ellipse- 2 MCQs are made for JEE 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Ellipse- 2 below.
Solutions of Test: Ellipse- 2 questions in English are available as part of our Mathematics (Maths) for JEE Main & Advanced for JEE & Test: Ellipse- 2 solutions in Hindi for Mathematics (Maths) for JEE Main & Advanced course. Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free. Attempt Test: Ellipse- 2 | 20 questions in 40 minutes | Mock test for JEE preparation | Free important questions MCQ to study Mathematics (Maths) for JEE Main & Advanced for JEE Exam | Download free PDF with solutions
Test: Ellipse- 2 - Question 1
Save

If distance between the directrices be thrice the distance between the foci, then eccentricity of ellipse is

Detailed Solution for Test: Ellipse- 2 - Question 1

Distance between the directrices is 2a/e
Distance between the foci is 2ae
Given: 2a/e = 3∗2ae
Or,e2 = 1/3
∴ e=1/√3

Test: Ellipse- 2 - Question 2
Save

If the eccentricity of an ellipse be 5/8 and the distance between its foci be 10, then its latus rectum is

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Ellipse- 2 - Question 3
Save

The curve represented by x = 3(cost + sint), y = 4(cost – sint), is

Test: Ellipse- 2 - Question 4
Save

If the distance of a point on the ellipse +  = 1 from the centre is 2, then the eccentric angle is
Test: Ellipse- 2 - Question 5
Save

An ellipse having foci at (3, 3) and (–4, 4) and passing through the origin has eccentricity equal to
Test: Ellipse- 2 - Question 6
Save

A tangent having slope of - 4/3 to the ellipse +  = 1 intersects the major & minor axes in points A & B respectively. If C is the centre of the ellipse then the area of the triangle ABC is
Detailed Solution for Test: Ellipse- 2 - Question 6

Since the major axis is along the y-axis.
∴ Equation of tangent is x = my + [b2m2 + a]1/2
Slope of tangent = 1/m = −4/3    
⇒ m = −3/4
Hence, equation of tangent is 4x+3y=24 or  
x/6 + y/8 = 1
Its intercepts on the axes are 6 and 8.
Area (ΔAOB) = 1/2×6×8
= 24 sq. unit

Test: Ellipse- 2 - Question 7
Save

The equation to the locus of the middle point of the portion of the tangent to the ellipse  +  = 1 included between the co-ordinate axes is the curve
Test: Ellipse- 2 - Question 8
Save

An ellipse is drawn with major and minor axes of lengths 10 and 8 respectively. Using one focus as centre, a circle is drawn that is tangent to the ellipse, with no part of the circle being outside the ellipse. The radius of the circle is
Detailed Solution for Test: Ellipse- 2 - Question 8

2a=10,a=5
2b=8,b=4
b2=a2(1−e2)
e2=1−16/25
e=3/5
Focus of ellipse (±3,0)
Taking (3,0) as centre, circle is drawn,
Radius of circle will be =5−3 = 2 units.

Test: Ellipse- 2 - Question 9
Save

Which of the following is the common tangent to the ellipses  +  = 1 & + = 1 ?
Detailed Solution for Test: Ellipse- 2 - Question 9

For x2/(a2 + b2) + y2/b2 = 1
Equation for tangent y = mx +- [(a2 + b2)m2 + b2]1/2
For x2/a2 + y2/(a2 + b2) = 1
Equation for tangent y = mx +- [a2m2 + (a2 + b2)}1/2
For common tangent: [(a2 + b2)m2 + b2]1/2 = [a2m2 + (a2 + b2)}1/2
(a2 + b2)m2 + b2 = a2m2 + (a2 + b2)
a2m2 + b2m2 + b2 = a2m2 + (a2 + b2)
b2m2 = a2
m = +- a/b
Equation for tangent : y = +- ax/b +-[(a2 + b2)a2/b2 + b2]1/2
y = +- ax/b +-{[a4 + a2b2 + b4]1/2}/b
by = +- ax +-{[a4 + a2b2 + b4]1/2}

Test: Ellipse- 2 - Question 10
Save

Angle between the tangents drawn from point (4, 5) to the ellipse  +  = 1 is
Test: Ellipse- 2 - Question 11
Save

The eccentricity of the ellipse  + = 1 is decreasing at the rate of 0.1/second due to change in semi minor axis only. The time at which ellipse become auxiliary circle is
Test: Ellipse- 2 - Question 12
Save

The point of intersection of the tangents at the point P on the ellipse  +  = 1, and its corresponding point Q on the auxiliary circle meet on the line
Detailed Solution for Test: Ellipse- 2 - Question 12

Test: Ellipse- 2 - Question 13
Save

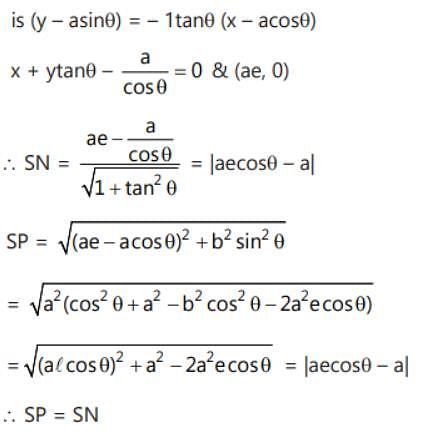
Q is a point on the auxiliary circle of an ellipse. P is the corresponding point on ellipse. N is the foot of perpendicular from focus S, to the tangent of auxiliary circle at Q. Then
Detailed Solution for Test: Ellipse- 2 - Question 13

P = (acosθ, bsinθ)
Q = (acosθ, asinθ)
equation of tangent at Q 

Test: Ellipse- 2 - Question 14
Save

Q is a point on the auxiliary circle corresponding to the point P of the ellipse  +  = 1. If T is the foot of the perpendicular dropped from the focus S onto the tangent to the auxiliary circle at Q then the D SPT is
Detailed Solution for Test: Ellipse- 2 - Question 14

Q = (acosθ, asinθ) 
 P = (acosθ, bsinθ) 
 ∆ SPT is an isosceles triangle.

Test: Ellipse- 2 - Question 15
Save

The equation of the normal to the ellipse  +  = 1 at the positive end of latus rectum is
Detailed Solution for Test: Ellipse- 2 - Question 15

 The positive end of latus rectum is

Test: Ellipse- 2 - Question 16
Save

The eccentric angle of the point where the line, 5x - 3y = 8√2 is a normal to the ellipse  +  = 1 is
Test: Ellipse- 2 - Question 17
Save

PQ is a double ordinate of the ellipse x2+ 9y2 = 9, the normal at P meets the diameter through Q at R, then the locus of the mid point of PR is
Detailed Solution for Test: Ellipse- 2 - Question 17

Equation of ellipse : x2/9 + y2/1= 1
Co−ordinates of point P is : (acosθ , bsinθ)
Equation of normal at point (x1, y1) is :
a2x/x1 − b2y/y1 = (ae)2
Equation of normal at point P is:
ax/cosθ− by/sinθ= (ae)2−−−−−(1)
Equation of diameter at Q is : y = (−b/a)tanθ−−−−−(2)
Point of intersection of equation 1 and 2 is :
ax/cosθ + (b2/acosθ) = (ae)2
Or , x = ae2cosθ − b2/a2
And y = − be2sinθ + b3/a3 (from 2)
∴ Point R(ae2cosθ − b2/a2 , − be2sinθ + b3/a3)
Let mid point of PR is (h,k)
h =[acosθ + ae2cosθ − (b2/a2)]/2
Or , 2h + b2/a2= acosθ + ae2cosθ
Or , cosθ= [2h + (b2/a2)/(a + ae2)]
k = [bsinθ − be2sinθ + (b3/a3/2)]/2
Or , sinθ = [2k − (b3/a3)/(b − be2)]
On squaring adding we get :
[2h + (b2/a2)]/(a + ae2)2 + [2k − (b3/a3)] / (b − be2)2= 1
Which is an equation of ellipse.

Test: Ellipse- 2 - Question 18
Save

Which of the following is the eccentricity for ellipse?
Detailed Solution for Test: Ellipse- 2 - Question 18

The eccentricity for ellipse is always less than 1. The eccentricity is always 1 for any parabola. The eccentricity is always 0 for a circle. The eccentricity for a hyperbola is always greater than 1.

Test: Ellipse- 2 - Question 19
Save

If F1 & F2 are the feet of the perpendiculars from the foci S1 & S2 of an ellipse  +  = 1 on the tangent at any point P on the ellipse, then (S1F1) . (S2F2) is equal to
Detailed Solution for Test: Ellipse- 2 - Question 19

Given,  x2/5 + y2/3= 1
We know S1F1 × S2F2 = b2
∴ S1F1 × S2F2 = 3

Test: Ellipse- 2 - Question 20
Save

If tan q1. tan q2 = – then the chord joining two points q1 & q2 on the ellipse +  = 1 will subtend a right angle at
Detailed Solution for Test: Ellipse- 2 - Question 20


Clearly, they subtend right angle at centre.

209 videos|443 docs|143 tests
Join Course
Information about Test: Ellipse- 2 Page
In this test you can find the Exam questions for Test: Ellipse- 2 solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Ellipse- 2, EduRev gives you an ample number of Online tests for practice

Top Courses for JEE

Download as PDF

Top Courses for JEE

Important Questions for Ellipse- 2

Find all the important questions for Ellipse- 2 at EduRev.Get fully prepared for Ellipse- 2 with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Ellipse- 2 syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Ellipse- 2 resources.

Ellipse- 2 MCQs with Answers

Prepare for the Ellipse- 2 within the JEE exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Ellipse- 2 Mathematics (Maths) for JEE Main & Advanced

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Ellipse- 2 with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Follow Us
Signup to see your scores go up
within 7 days!
Takes less than 10 seconds to signup