Test: Cartesian Equation Of A Line - JEE MCQ
10 Questions MCQ Test Mathematics (Maths) Class 12 - Test: Cartesian Equation Of A Line
Let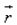 
be the position vector of an arbitrary point P(x, y, z). Cartesian form of the equation of line passes through two points (x1, y1, z1) and (x2, y2, z2) is:
be the position vector of an arbitrary point P(x, y, z). Cartesian form of the equation of line passes through two points (x1, y1, z1) and (x2, y2, z2) is:Find the vector equation of the line that passes through the origin and (-6,2,1).
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
The Cartesian equation of the line which passes through the point (2, -2, -1) and parallel to the line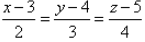 
, is given by
, is given byIf the vector equation of a line is 
find its caryesian equation.
The vector form of the equation is
. The Cartesian equation of the line is:
Let the coordinates of the given point A be (x1, y1, z1) and the direction ratios of the line be a, b, c. If the co-ordinates of any point P is (x, y, z), then the equation of the line in Cartesian form is:
Let 
be a position vector of A with respect to the origin O and 
be a position vector of an arbitrary point. The equation of line which passes through A and parallel to a vector 
is:
The Cartesian equation of the line passing through the points (-3, 1, 0) and (1, 2, 3) is:
The Cartesian equation of the line which passes through the origin and parallel to the line 
, is given by
Find the Cartesian equation of the line that passes through the point (-3,-4,-2) and (3,4,2).
|
204 videos|290 docs|139 tests
|
|
204 videos|290 docs|139 tests
|
Top Courses for JEE
Important Questions for Cartesian Equation Of A Line
Cartesian Equation Of A Line MCQs with Answers
Online Tests for Cartesian Equation Of A Line Mathematics (Maths) Class 12
|
© EduRev
|
Education Revolution
|
Follow Us
|
