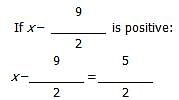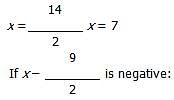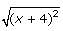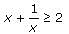Test: Inequalities + Absolute Value (Modulus) - GMAT MCQ
15 Questions MCQ Test Quantitative for GMAT - Test: Inequalities + Absolute Value (Modulus)
If -1 < x < 0, which of the following must be true?
I. x3 < x2
II. x5 < 1 – x
III. x4 < x2
I. x3 < x2
II. x5 < 1 – x
III. x4 < x2
If a – b > a + b, where a and b are integers, which of the following must be true?
I. a < 0
II. b < 0
III. ab < 0
I. a < 0
II. b < 0
III. ab < 0
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
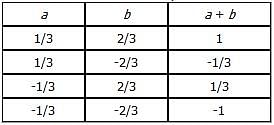
If |a| = 1/3 and |b| = 2/3, which of the following CANNOT be the result of a + b?
If |a| = |b|, which of the following must be true?
I. a = b
II. |a| = -b
III. -a = -b
Which of the following inequalities has a solution set that when graphed on the number line, is a single
segment of finite length?
If |x – (9/2)| = 5/2, and if y is the median of a set of p consecutive integers, where p is odd, which of the
following must be true?
I. xyp is odd
II. xy(p2 + p) is even
III. x2y2p2 is even
If a, b, c, and d are integers and ab2c3d4 > 0, which of the following must be positive?
I. a2cd
II. bc4d
III. a3c3d2
If 3|3 – x| = 7, what is the product of all the possible values of x?
If √[(x + 4)2] = 3,which of the following could be the value of x – 4?
If |x | + |y | = -x – y and xy does not equal 0, which of the following must be true?
If x > 0, what is the least possible value for x + (1/x)?
If (a – b)c < 0, which of the following cannot be true?
If |ab| > ab, which of the following must be true?
I. a < 0
II. b < 0
III. ab < 0
If b < c < d and c > 0, which of the following cannot be true if b, c and d are integers?
If ab > cd and a, b, c and d are all greater than zero, which of the following CANNOT be true?
|
110 videos|110 docs|120 tests
|
|
110 videos|110 docs|120 tests
|