CAT Previous Year Questions: Probability & Combinatorics (July 23) - CAT MCQ
10 Questions MCQ Test Daily Test for CAT Preparation - CAT Previous Year Questions: Probability & Combinatorics (July 23)
The number of ways of distributing 20 identical balloons among 4 children such that each child gets some balloons but no child gets an odd number of balloons, is (2022)
The arithmetic mean of all the distinct numbers that can be obtained by rearranging the digits in 1421, including itself, is (2022)
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
The number of integers greater than 2000 that can be formed with the digits 0, 1, 2, 3, 4, 5, using each digit at most once, is (2022)
The number of ways of distributing 15 identical balloons, 6 identical pencils and 3 identical erasers among 3 children, such that each child gets at least four balloons and one pencil, is (2021)
The number of groups of three or more distinct numbers that can be chosen from 1, 2, 3, 4, 5, 6, 7 and 8 so that the groups always include 3 and 5, while 7 and 8 are never included together is (2021)
How many three-digit numbers are greater than 100 and increase by 198 when the three digits are arranged in the reverse order? (2021)
A four-digit number is formed by using only the digits 1, 2 and 3 such that both 2 and 3 appear at least once. The number of all such four-digit numbers is (2021)
With rectangular axes of coordinates, the number of paths from (1, 1) to (8, 10) via (4, 6), where each step from any point (x, y) is either to (x, y+1) or to (x+1, y), is (2019)
How many two-digit numbers, with a non-zero digit in the units place, are there which are more than thrice the number formed by interchanging the positions of its digits? (2018)
In a tournament, there are 43 junior level and 51 senior level participants. Each pair of juniors play one match. Each pair of seniors play one match. There is no junior versus senior match. The number of girl versus girl matches in junior level is 153, while the number of boy versus boy matches in senior level is 276. The number of matches a boy plays against a girl is (2018)
|
152 docs|327 tests
|


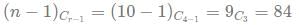
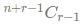 ways, where n = 3 and r = 3.
ways, where n = 3 and r = 3. 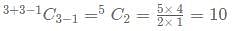
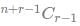 ways, where n = 3 and r = 3.
ways, where n = 3 and r = 3.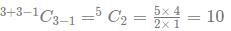
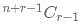 ways, where n = 3 and r = 3.
ways, where n = 3 and r = 3.