Test: Simple Harmonic Motion(9 Oct) - JEE MCQ
15 Questions MCQ Test Daily Test for JEE Preparation - Test: Simple Harmonic Motion(9 Oct)
Two identical spring, each of stiffness k are welded to each other at point P. The other two ends are fixed to the edge of a smooth horizontal tube as shown. A particle of mass m is welded at P. The entire system is horizontal. The period of oscillation of the particle in the direction of x is 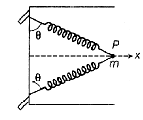
Two pendulums of length 100 cm and 121 cm starts oscillating. At some instant, the two are at the mean position in the same phase. After how many oscillations of the longer pendulum will the two be in the same phase at the mean position again
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
A pendulum has time period T for small oscillations. Now, an obstacle is situated below the
point of suspension O at a distance 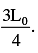 The pendulum is released from rest. Throughout the motion, the moving string makes small angle with vertical. Time after which the pendulum returns back to its initial position is
The pendulum is released from rest. Throughout the motion, the moving string makes small angle with vertical. Time after which the pendulum returns back to its initial position is
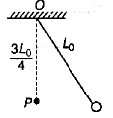
point of suspension O at a distance
In the figure is shown a small block B of mass m resting on a smooth horizontal floor and the block is attached to an ideal spring (of force constant k). The spring is attached to vertical wall W1. At a distance d from the block, right side of it, is present the vertical wall W2. Now, the block is compressed by a distance 5d/3 and released. It starts oscillating. If the collision of the block with W2 are perfectly elastic, the time period of oscillation of the block is
Two simple pendulums of length 1m and 25 m, respectively, are both given small displacements in the same direction at the same instant.If they are in phase after the shorter pendulum has completed n oscillation, n is equal to
Three masses of 500 g, 300 g and 100 g are suspended at the end of an ideal spring as shown and are in equilibrium. When the 500 g mass is suddenly removed, the system oscillated with a period of 2 s. When 300 g mass is also removed, it will oscillate with the period

A particle of mass m is executing oscillations about the origin on the X-axis with amplitude A. Its potential energy is given as U(x) = βx4 where β is a positive constant. The x- cordinate of the particle, when the potential energy is one- third of the kinetic energy, is
A linear harmonic oscillator of force constant 2 x 106 Nm–1 and amplitude 0.01 m has a total mechanical energy 160 J. Among the following statements, which are correct?
The potential energy between two atoms in a diatomic molecule varies with x as where a and b are positive constants. Find the equivalent spring constant for
the oscillation of one atom if the other atom is kept
A particle of mass m moves in the potential energy U shown in the figure. The particle of the motion, if the total energy of the particle is Eo , is
A particle of mass 0.1 kg is executing SHM of amplitude 0.1 m. When the particle passes through the mean position, its KE is 8 ×10-3 J. Find the equation of motion of the particle if the initial phase of oscillation is 45˚
The distance travelled by a particle executing SHM in 10 s, if the time period is 3 s, is
(It is given that the body starts from A√3/3 from equilibrium position, moves is positive direction at t = 0)
Two particles executing SHM with same angular frequency and amplitude A and 2A same
straight line with same position cross other in opposite direction at a distance A/3 from mean position. The phase difference between the two SHM’s is
A spring-block pendulum is shown in the figure. The system is hanging in equilibrium. A bullet of mass m/2 moving with a speed u hits the block from down as shown in the figure. Find the amplitude of oscillation now.
A particle at the end of a spring executes simple harmonic motion with a period T1 while the corresponding period for another spring is T2 If the period of oscillation with the two springs in series is T, then
|
360 tests
|


















