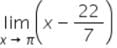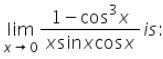Test: Introduction And Algebra Of Limits - Grade 9 MCQ
10 Questions MCQ Test - Test: Introduction And Algebra Of Limits
If the right and left hand limits coincide, we call that common value as the limit of f(x) at x = a and denote it by
For the limit of a function to exist we must have
Let f be any function, such that 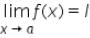 exists, then
exists, then 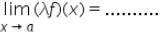
Let f(x) and g(x) be two function, such that 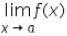 and
and 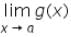 exists, then the limit of the product of the function f(x) and g(x) is given by
exists, then the limit of the product of the function f(x) and g(x) is given by
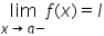 is the expected value of f at x = a given the values of f near x to the left of a. This value is called the……….of f at a.
is the expected value of f at x = a given the values of f near x to the left of a. This value is called the……….of f at a.
As x → a, f(x) → l, then l is called the……..of the function f(X) which is symbolically written as…….
Consider the function f(x) = x + 10. Let us compute the value of the function f(x) for x very near to 5. Some of the points near and to the left of 5 and right to the 5 are given in the table.
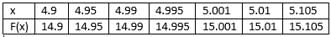
Based on the values, what is most approximante value of the function f(x) at x = 5?