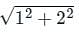Civil Engineering (CE) Exam > Civil Engineering (CE) Tests > Engineering Mathematics > Test: Minima & Maxima - 2 - Civil Engineering (CE) MCQ
Test: Minima & Maxima - 2 - Civil Engineering (CE) MCQ
Test Description
10 Questions MCQ Test Engineering Mathematics - Test: Minima & Maxima - 2
Test: Minima & Maxima - 2 for Civil Engineering (CE) 2024 is part of Engineering Mathematics preparation. The Test: Minima & Maxima - 2 questions and answers have been
prepared according to the Civil Engineering (CE) exam syllabus.The Test: Minima & Maxima - 2 MCQs are made for Civil Engineering (CE) 2024 Exam. Find important
definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Minima & Maxima - 2 below.
Solutions of Test: Minima & Maxima - 2 questions in English are available as part of our Engineering Mathematics for Civil Engineering (CE) & Test: Minima & Maxima - 2 solutions in
Hindi for Engineering Mathematics course. Download more important topics, notes, lectures and mock
test series for Civil Engineering (CE) Exam by signing up for free. Attempt Test: Minima & Maxima - 2 | 10 questions in 30 minutes | Mock test for Civil Engineering (CE) preparation | Free important questions MCQ to study Engineering Mathematics for Civil Engineering (CE) Exam | Download free PDF with solutions
Detailed Solution for Test: Minima & Maxima - 2 - Question 1
Test: Minima & Maxima - 2 - Question 2
What is the maximum value of the expression 1/(2x2 + 5x + 11)?
Detailed Solution for Test: Minima & Maxima - 2 - Question 2
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Detailed Solution for Test: Minima & Maxima - 2 - Question 3
Test: Minima & Maxima - 2 - Question 4
If x2 + y2 = 1, find the maximum value of x2 + 4xy - y2.
Detailed Solution for Test: Minima & Maxima - 2 - Question 4
Test: Minima & Maxima - 2 - Question 5
If x is real, then find the the minimum value of (5x2 - 2x + 7).
Detailed Solution for Test: Minima & Maxima - 2 - Question 5
Test: Minima & Maxima - 2 - Question 6
If x is real, find the minimum value of 4x2 - 5x + 1 = 0
Detailed Solution for Test: Minima & Maxima - 2 - Question 6
Test: Minima & Maxima - 2 - Question 7
What is the maximum value of the expression 1/(3x2 + 4x + 8)?
Detailed Solution for Test: Minima & Maxima - 2 - Question 7
Test: Minima & Maxima - 2 - Question 8
What will be the maximum value of (x – 5)(y – 2)(z – 6) if (x + y + z) = 22?
Detailed Solution for Test: Minima & Maxima - 2 - Question 8
Detailed Solution for Test: Minima & Maxima - 2 - Question 9
Test: Minima & Maxima - 2 - Question 10
If x is real, then find the maximum value of (-3x2 + 5x + 10).
Detailed Solution for Test: Minima & Maxima - 2 - Question 10
|
65 videos|120 docs|94 tests
|
Information about Test: Minima & Maxima - 2 Page
In this test you can find the Exam questions for Test: Minima & Maxima - 2 solved & explained in the simplest way possible.
Besides giving Questions and answers for Test: Minima & Maxima - 2, EduRev gives you an ample number of Online tests for practice
|
65 videos|120 docs|94 tests
|
Download as PDF


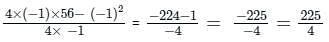
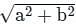 .
.