Absolute Value | Mathematics for ACT PDF Download

Introduction

The absolute value of a variable x is represented by |x| which is pronounced as 'Mod x' or 'Modulus of x'. 'Modulus' is a Latin word, which means 'measure'. Absolute value is commonly referred to as numeric value or magnitude. The absolute value represents only the numeric value and does not include the sign of the numeric value. The modulus of any vector quantity is always taken as positive and is its absolute value. Also, quantities like distance, price, volume, and time, are always represented as absolute values.
As an example the absolute value: |+5| = |-5| = 5. There is no sign assigned to absolute value. In this article, we will explore the concept of the absolute value of a number, its symbol, and how to find the absolute value. We will solve various examples based on the concept for a better understanding.

What is Absolute Value?

The absolute value of a number is its distance from the origin 0. We know that distance is always a non-negative quantity. Since the absolute value is a distance, the absolute value of a number is always a non-negative number. Sometimes a sign is attributed to a numeric value to signify the direction, in addition to the value. The increase or a decrease of a quantity, values above or below the mean value, profit, or loss in a transaction, is sometimes explained by assigning a positive or negative value to the numeric value. But for absolute value, the sign of the numeric value is ignored and only the numeric value is considered.

Absolute Value Definition

The absolute value of a number is defined as its magnitude irrespective of the sign of the number. To find the absolute value of a real number, we consider only the number and remove the sign. It can only be a non-negative value. The absolute value of a positive number is the number itself, that of a negative number is the number without a negative sign, and the absolute value of 0 is 0.

Absolute Value of a Number Line

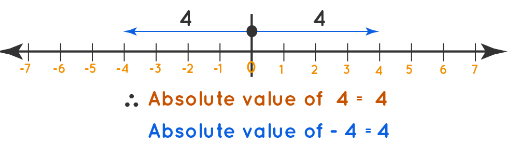
In the above figure, we can observe the absolute values on the number line using the illustration. The absolute value is represented by |x|, and in the above illustration, |4| = |-4| = 4.

Absolute Value Sign

To represent the absolute value of a number (or a variable), we write a vertical bar on either side of the number i.e., |x| where x is an integer. For example, the absolute value of 4 is written as |4|. Also, the absolute value of -4 is written as |-4|. As we discussed earlier, the absolute value results in a non-negative value all the time. Hence, |4|=|-4| =4. That is, it turns negative numbers also into positive numbers. The following figure represents the absolute value symbol.

Representation of Absolute Value Symbol

Absolute Value of a Number

As discussed above, the absolute value of a number is always a non-negative value. To determine the absolute value of a number x, we denote it is |x| and its formula is given by, |x| = x, if x > 0 and |x| = -x, if x < 0, and |x| = 0, if x = 0. Some of the examples of absolute value of a number are:

• |2| = 2
• |-9| = 9
• |3.4| = 3.4
• |-1.23| = 1.23

Absolute Value of 0

So far we have discussed the absolute value of positive and negative numbers. Now, we will discuss the absolute value of zero. Since 0 is neither positive nor positive, therefore the absolute value of 0 is equal to 0. The distance of the number 0 is equal to zero from 0, therefore we can say that the absolute value of 0 is 0.

Absolute Value Function

The absolute value function is defined as f(x) = |x|, { |x| = +x for x ≥ 0, and |x| = -x for x < 0} Using the definition of absolute value, we know that it always results in a non-negative number. Thus, the graph of f(x) = |x| looks as follows.

Graph of Absolute Value

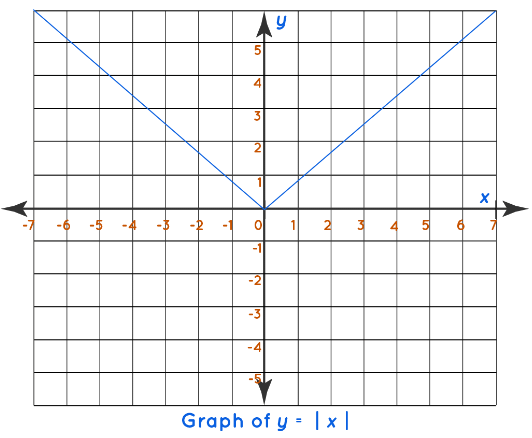
From the definition of absolute value function, the value of |x| depending on the sign of x. |x|= + x. We also know that √ {x²} = + x. Therefore we have √{x²} = |x|.

Important Notes on Absolute Value

• The absolute value of x is represented by either |x| or abs(x).
• The absolute value of any number always results in a non-negative value.
• We pronounce |x| as 'mod x' or 'modulus of x.'