Average | Quantitative Techniques for CLAT PDF Download
| Table of contents |

|
| Introduction |

|
| Properties of Average |

|
| Shortcut techniques in Averages |

|
| Solved Examples |

|
Introduction
Averages can be defined as the central value in a set of data. Average can be calculated simply by dividing the sum of all values in a set by the total number of values. In other words, an average value represent the middle value of a data set.
Average i.e. mean =
Example:
What is the average of first five consecutive odd numbers?
Solution:
The first five consecutive odd numbers are: 1, 3, 5, 7, 9.
Here, the number of data or observations is 5 and the sum of these 5 numbers is 25.
So, average = 25 / 5 = 5.
Properties of Average
- The average of the given numbers lies between the smallest and the largest number.
- If the numbers (whose average is to be found) are at equal distance, the number in the middle of the series (after arranging them in ascending or descending order) is the average.
- The sum of the differences of the numbers, which are less than the average, from the average is always equal to the sum of the differences of the numbers, greater than the average, from the average.
Formula for calculating Average
Example: 10, 12, 15, 16, 20, 35 numbers have the average as
Numbers 10, 12, 15 and 16 are less than the average 18.
Let us find out the sum of the differences of these numbers from the average?
Solution. The differences are 18 – 10 = 8, 18 – 12 = 6, 18 – 15 = 3 18 – 16 = 2
- The sum of the differences is 8 + 6 + 3 + 2 = 19
- Now find out the difference of the numbers, greater than the average, from the average.
- The differences are 20 – 18 = 2, 35 – 18 = 17
∴ The Sum of the differences = 2 + 17 = 19
So, we find that sum of the differences of smaller numbers from average is equal to the sum of differences of larger numbers.
- While computing average, if 0 is one of the quantities, then this should also be included in the calculation of average.
- Unit of average is the same as that of the given quantities
Example:
= 6 cm
- The sum of the quantities = Their average x Their number.
Example: If the average of 5 quantities is 20 (say), then the sum of these quantities is 20 x 5 = 100.
- When a Person replaces another person in the group(age, weight, height and similar kind of Problems)
If the average is increased: New member’s age = Age of person who left+(Increase in average*total number of People)
If the average is decreased: New member’s age = Age of person who left - (Decrease in average*total number of People)
When someone joins the group:
- Increase in average: New member’s age = Earlier average + (Increase in average*total number of People)
- Decrease in average: New member’s Age = Earlier average – (Decrease in average*total number of People)
- If each one of the given numbers is multiplied/ divided by a number k, then the average of the given numbers will be multiplied/divided by k.
- Average of first n natural numbers is

- If a person travels a distance at a speed of x km/hour and the same distance at a speed of y km/hr, then the average speed during the whole journey is

- If a person travels three equal distances at the speed of x km/hr, y km/hr, and z km/hr respectively, then the average speed during the whole journey is given by km/hr.
Shortcut techniques in Averages
Questions based on averages can be easily solved using shortcuts. By using shortcuts, any question can be solved quickly and efficiently which can save a lot of time. So, some shortcuts to solve average questions are explained below along with illustrations.
Shortcut to find average or change in average from a set of values
Example 1:
The average of a batsman in 16 innings is 36. In the next innings, he is scoring 70 runs. What will be his new average?
a) 44
b) 38
c) 40
d) 48
Solution:
Given:
Average score of batsman in 16 innings = 36.
Score of batman in 17th innings = 70.
Formula used:
Average score of a batsman = Sum of runs / Number of innings.
Calculation:
Average score of batsman in 16 innings = Sum of runs / Number of innings
36 = Sum of runs / 16
Sum of runs = 16 × 36
Sum of runs = 576
Sum of runs after the 17th innings = 576 + 70 = 646.
Average score of batsman after 17th innings
= Sum of runs after 17th innings / Number of innings
= 646 / 17
= 38.
∴ His new average will be 38.
Shortcut to find new value when average is given
Now here is a technique which will help to compute the new value when the average is given. Take this question for example:
Example 2:
The average age of 29 students is 18. If the age of the teacher is also included the average age of the class becomes 18.2. Find the age of the teacher?
a) 28
b) 32
c) 22
d) 24
Solution:
Given:
Average age of 29 students is 18.
The age of the teacher included in the class and the average becomes 18.2.
Concept used:
Average = (sum of observations) / (number of observations)
Calculations:
Sum of 29 students = 18 × 29 = 522
If a Teacher is included in the class then the total number of heads will be 29 + 1 = 30.
Then the average becomes 18.2 when the teacher is included in the class, so the sum of 30 heads in a class = 30 × 18.2 = 546
Therefore, teachers age = 546 - 522 = 24 years.
∴ The age of the teacher is 24 years.
Solved Examples
Example 3. Nine persons went to a hotel to take meals. Eight of them spent Rs 12 each and the ninth spent Rs 8 more than the average expenditure of all the nine. What was the total money spent by them.
Solution. Let the average expenditure of all the nine be Rs x
Then 12 x 8 + ( x + 8) = 9x
or 8x = 104 or x = 13
Total money spent = 9 x = 9 X 13 = 117.
Example 4. There are 24 kids in a class and the average weight of a class is 38 kg. If the weight of the teacher is also included then average increases by 1.5 kg. What is the weight of the teacher?
Solution.
Conventional Method:
Total weight of students = Average weight of students*No. of Students
= 38*24 = 912 kg
New average = 38+2 = 39.5 kg
Total number of People = 24+1 (including teacher) = 25
New total of weights = 39.5*25 = 987.5
Weight of teacher = New total of weights – Previous Total weights of Students
= 987.5-912 = 75.5 kg
Shortcut
As the question says there is an increase in the average by 1.5 kg when teacher’s weight is also considered so we will apply the formula discussed above.
► Teachers weight = Earlier Average + (Increase in average * Total number of people)
= 38 + (1.5*25)
= 38 + (37.5) = 75.5 kg
Hence, Teacher’s weight would be 75.5 kg
See the difference in conventional and shortcut method, Due to involvement of numerous steps, the conventional method becomes more time taking although it’s not too long if you are in a habit of oral number crunching but if not then it is advised to use shortcuts.
Example 5. Average runs made by Viraj in 15 matches is 42.8. the average of his first 8 matches is 55.4. What is the average of his last 7 matches?
Solution. As we need average of Viraj’s last 7 matches run we will first find out how many runs he made in those last 7 matches by subtracting runs of his first 8 matches from total runs.
► Total Runs made by Viraj in 15 matches = Average runs made*No. of matches = 42.8*15 = 642
► Runs made by Viraj in first 8 marches = 55.4 * 8 = 443.2
► Runs made by Viraj in last 7 matches = 642 - 443.2 = 198.8
► The average number of runs made by Viraj in his last 7 matches = 198.8/7
= 28.4
Example 6. The average age of 8 persons is increased by 2 years when one of them whose age is 24 years is replaced by a new person. The age of the new person is
(a) 42 years
(b) 40 years
(c) 38 years
(d) 45 years
Solution. The average age of 8 person = x years
Now the total age of 8 person = 8x years
It is mentioned that the average age of 8 person has increased after 2 years.
So now the new average age = (x + 2) years.
Therefore the total age of 8 person = 8(x + 2) years.
Difference of ages = 8(x + 2) − 8x years
⇒ 8x − 16 − 8x Years.
⇒ ∴ 16 Years.
Hence the difference of ages = 16 years.
We clearly know that the new person got replaced 24 years ago by an unknown person.
Now age of new person = 24 + 16 = 40 years
|
56 videos|104 docs|95 tests
|
FAQs on Average - Quantitative Techniques for CLAT
| 1. What are some properties of averages? |  |
| 2. What are some shortcut techniques for calculating averages quickly? |  |
| 3. How can averages be helpful in competitive exams like CLAT? |  |
| 4. How can I improve my understanding of averages for the CLAT exam? |  |
| 5. Can averages be used in other sections of the CLAT exam besides mathematics? |  |

|
Explore Courses for CLAT exam
|

|



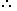 11x - (26 + 29) = 9(x -1)
11x - (26 + 29) = 9(x -1)

















