Binary Logic: Solved Examples | CAT Preparation Course for Working Professionals PDF Download
Directions (1 -4): Read the following information and answer the questions below.
In a coaching institute there are total 170 students and they studied different subject’s viz. Economics, Maths and English.
The ratio of students studying all 3 subjects to students studying atleast 2 subjects is 2:9. The ratio of students studying only one subject to students studying atleast 2 subjects is 8:9.Number of students taking Maths only exceeds number of students of Economics only by 14.
Number of students studying English only exceeds number of students of Economics only by 12. Number of students studying English, Economics, and Maths is 90, 93, 97 respectively.
1. Number of students studying all three subjects is:
a) 18
b) 12
c) 20
d) None of these
Answer: c
Explanation: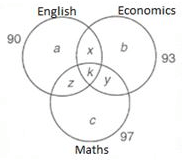
a+b+c=α, x+y+z=β, k=γ
α+β+γ=170
α+2β+3γ = 90 + 93 + 97 = 280
γ : (β+γ) = 2:9
⇒ γ : β = 2 : 7
and α : ( β + γ) = 8 : 9
⇒ α : β : γ = 8 : 7 : 2
∴ α = 80, β = 70 and γ = 20
⇒ a+b+c = 80, x+y+ z = 70 ...(1)
and k = 20
Again c-b = 14 and a-b = 12 ...(2)
On solving eq. (1) and (2) we get a= 30, b= 18, c= 32
Again (a +x + k + z)-(a+k) = (x + z)
= 90 – (30 + 20) = 40
and (x + y + z) - (x + z) = y =70 - 40 = 30
Similarly x= 25 and z= 15
2. Number of students studying no more than one subject is:
a) 76
b) 80
c) 60
d) can’t be determined
Answer: b
Explanation:
a+b+c=α, x+y+z=β, k=γ
α+β+γ=170
α+2β+3γ = 90 + 93 + 97 = 280
γ : (β+γ) = 2:9
⇒ γ : β = 2 : 7
and α : ( β + γ) = 8 : 9
⇒ α : β : γ = 8 : 7 : 2
∴ α = 80, β = 70 and γ = 20
⇒ a+b+c = 80, x+y+ z = 70 ...(1)
and k = 20
Again c-b = 14 and a-b = 12 ...(2)
On solving eq. (1) and (2) we get a= 30, b= 18, c= 32
Again (a +x + k + z)-(a+k) = (x + z)
= 90 – (30 + 20) = 40
and (x + y + z) - (x + z) = y =70 - 40 = 30
Similarly x= 25 and z= 15
3. Number of students using exactly two subjects is:
a) 38
b) 55
c) 70
d) none of these
Answer: c
Explanation:
a+b+c=α, x+y+z=β, k=γ
α+β+γ=170
α+2β+3γ = 90 + 93 + 97 = 280
γ : (β+γ) = 2:9
⇒ γ : β = 2 : 7
and α : ( β + γ) = 8 : 9
⇒ α : β : γ = 8 : 7 : 2
∴ α = 80, β = 70 and γ = 20
⇒ a+b+c = 80, x+y+ z = 70 ...(1)
and k = 20
Again c-b = 14 and a-b = 12 ...(2)
On solving eq. (1) and (2) we get a= 30, b= 18, c= 32
Again (a +x + k + z)-(a+k) = (x + z)
= 90 – (30 + 20) = 40
and (x + y + z) - (x + z) = y =70 - 40 = 30
Similarly x= 25 and z= 15
4. The number of students who are studying both Economics and Maths but not English is:
a) 23
b) 40
c) 36
d) data insufficient
Answer: b
Explanation:
a+b+c=α, x+y+z=β, k=γ
α+β+γ=170
α+2β+3γ = 90 + 93 + 97 = 280
γ : (β+γ) = 2:9
⇒ γ : β = 2 : 7
and α : ( β + γ) = 8 : 9
⇒ α : β : γ = 8 : 7 : 2
∴ α = 80, β = 70 and γ = 20
⇒ a+b+c = 80, x+y+ z = 70 ...(1)
and k = 20
Again c-b = 14 and a-b = 12 ...(2)
On solving eq. (1) and (2) we get a= 30, b= 18, c= 32
Again (a +x + k + z)-(a+k) = (x + z)
= 90 – (30 + 20) = 40
and (x + y + z) - (x + z) = y =70 - 40 = 30
Similarly x= 25 and z= 15
Directions (5 - 7): Read the following information and answer the questions below.
50 students sing, 60 students do not dance and 25 students do both in a class of 100 students.
5. How many students neither sing nor dance?
a) 65
b) 35
c) 15
d) Cannot be determined
Answer: b
Explanation: Students who neither sing nor dance= Total students – Students who do sing alone, dance alone and do both
From Venn diagram, Students who neither sing nor dance= 100-(25+25+15)= 35
(See the Venn diagram and explanation at the end of the questions)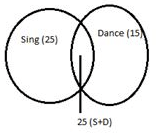
Given,
Total students= 100
Number of students who sing= 50
Number of students who do not dance= 60
So, number of students who dance= 100-60= 40 (Total students- Students who do not dance)
Students who dance and sing= 25 (Overlapping portion in the Venn diagram)
Number of students who sing alone= 50-25=25 (Students who sing- Students who sing and dance)
Number of students who dance alone= 40-25=15 (Students who dance- Students who sing and dance)
6. How many students only dance?
a) 10
b) 15
c) 20
d) Cannot be determined
Answer: b
Explanation: (See the Venn diagram and explanation at the end of the questions)
Given,
Total students= 100
Number of students who sing= 50
Number of students who do not dance= 60
So, number of students who dance= 100-60= 40 (Total students- Students who do not dance)
Students who dance and sing= 25 (Overlapping portion in the Venn diagram)
Number of students who sing alone= 50-25=25 (Students who sing- Students who sing and dance)
Number of students who dance alone= 40-25=15 (Students who dance- Students who sing and dance)
7. How many students at least do either sing or dance?
a) 60
b) 50
c) 75
d) 65
Answer: d
Explanation: (See the Venn diagram and explanation at the end of the questions)
Given,
Total students= 100
Number of students who sing= 50
Number of students who do not dance= 60
So, number of students who dance= 100-60= 40 (Total students- Students who do not dance)
Students who dance and sing= 25 (Overlapping portion in the Venn diagram)
Number of students who sing alone= 50-25=25 (Students who sing- Students who sing and dance)
Number of students who dance alone= 40-25=15 (Students who dance- Students who sing and dance)
Direction (8-10): Read the following information and answer the questions below.
There are 120 employees who work for Airtel Pvt. Ltd. Mumbai, out of which 50 are women. Also:
I. 56 workers are married
II. 52 workers are graduate
III. 40 married workers are graduate of which 18 are men
IV. 30 men are graduate
V. 30 men are married.
8. How many unmarried women are graduate?
a) 22
b) 16
c) 0
d) can’t be determined
Answer: c
Explanation: No one unmarried woman is graduate.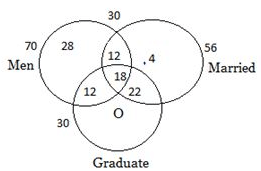
Total number of employees = 120
Women = 50
Men = 70
Married workers = 56
Graduate workers = 52
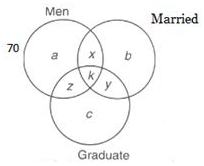
a → unmarried men who are not graduate
b → married women who are not graduate
c → unmarried women who are graduate
x → married men who are not graduate
y → married women who are graduate
z → unmarried men who are graduate
k → married men who are graduate
p → unmarried women who are not graduate
According to the given information the Venn diagram can be completed as given below.
9. How many unmarried women are graduate?
a) 21
b) 24
c) 19
d) none of these
Answer: b
Explanation: Number of unmarried women = 120─ [28 + 4 + 12 + 12 + 22 + 18] = 24
Total number of employees = 120
Women = 50
Men = 70
Married workers = 56
Graduate workers = 52
a → unmarried men who are not graduate
b → married women who are not graduate
c → unmarried women who are graduate
x → married men who are not graduate
y → married women who are graduate
z → unmarried men who are graduate
k → married men who are graduate
p → unmarried women who are not graduate
According to the given information the Venn diagram can be completed as given below.
10. How many graduate men are married?
a) 18
b) 15
c) 13
d) none of these
Answer: a
Explanation: There are 18 graduate men who are married.
Total number of employees = 120
Women = 50
Men = 70
Married workers = 56
Graduate workers = 52
a → unmarried men who are not graduate
b → married women who are not graduate
c → unmarried women who are graduate
x → married men who are not graduate
y → married women who are graduate
z → unmarried men who are graduate
k → married men who are graduate
p → unmarried women who are not graduate
According to the given information the Venn diagram can be completed as given below.
|
303 videos|487 docs|279 tests
|
FAQs on Binary Logic: Solved Examples - CAT Preparation Course for Working Professionals
| 1. What is binary logic? |  |
| 2. How is binary logic used in computers? |  |
| 3. What are the basic logical operators in binary logic? |  |
| 4. Can binary logic be applied to real-life situations? |  |
| 5. Are there any limitations to binary logic? |  |

















