Calculations of pH and pOH Solved Examples | Chemistry for JAMB PDF Download
Q1: Sodium hydroxide is a strong base. What is the pH of a 0.02M sodium hydroxide solution?
(a) 12.3
(b) 1.7
(c) 12.0
(d) 2.0
Ans: (b)
Since sodium hydroxide is a strong base, it will dissociate completely in water. This means that the concentration of the base will be equal to the concentration of hydroxide ions after the reaction runs to completion.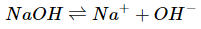
We can find the concentration of hydroxide ions via stoichiometry. One hydroxide ion is created from each molecule of sodium hydroxide that dissociates.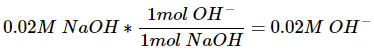
Since we have the concentration of hydroxide ions, we can solve for the pOH of the solution.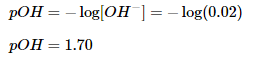
The question asks us to find the pH of the solution, so we will need to convert pOH to pH. To do so, we simply subtract the pOH from 14.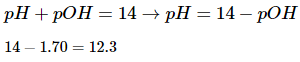
The pH of the solution is 12.3. Because sodium hydroxide is a strong base, it makes sense that the pH is above 7.
Q2: What is the pH for a 0.05M solution of hydrochloric acid?
(a) −0.3
(b) 0.05
(c) 1.3
(d) 6.95
Ans: (c)
Hydrochloric acid is a strong monoprotic acid, meaning that it will dissociate completely in solution and generate one proton from each acid molecule. This means that a 0.05M solution of hydrochloric acid will result in a 0.05M concentration of protons.
The equation for pH is as follows: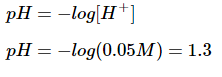
Q3: You are presented with a solution that has a pOH of 2.13. What is the pH of this solution?
(a) 6.57
(b) 11.87
(c) 2.13
(d) None of these
Ans: (b)
pH and pOH are the log concentrations of protons and hydroxide ions, respectively.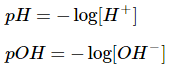
The sum of pH and pOH is always 14. This is because the product of proton concentration and hydroxide concentration must always equal the equilibrium constant for the ionization of water, which is equal to 10−14.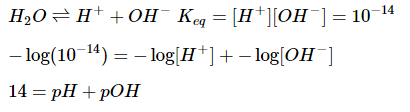
In this question, we know that the pOH is equal to 2.13, allowing us to solve for the pH.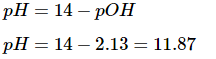
Q4: Hydrofluoric acid has a Ka value of 7.2∗10−4. What is the pH of a 0.04M solution of hydrofluoric acid?
(a) 11.7
(b) 1.4
(c) 2.3
(d) 4.5
Ans: (c)
Hydrofluoric acid is a weak acid, meaning that we will need to use an ICE table in order to find the pH of the solution.
The balanced reaction for hydrofluoric acid in water is: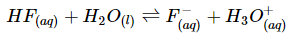
I: Before the reaction proceeds, we have 0.04M of hydrofluoric acid. Since water is a liquid, its concentration is irrelevant for the equilibrium expression. There are also no products yet made.
C: Once the reaction reaches equilibrium, both the hydronium and fluoride concentrations will have increased by an unknown concentration. We will call this increase x. Conversely, the concentration of hydrofluoric acid concentration will have decreased by the same amount, in this case x.
E: Using the equilibrium expression and making it equal to the acid dissociation constant, we can solve for x.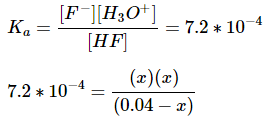
Note: Since the value for x is going to be very small compared to the initial acid concentration, we can disregard the x in the denominator: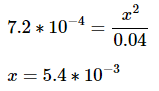
Keep in mind that x is equal to the concentration of hydronium ions now in the solution.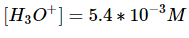
Use this value in the equation for pH: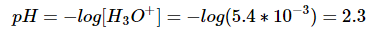
Q5: What is the pH of a 0.02M ammonia solution if its Kb value is 1.8∗10−5?
(a) 7.6
(b) 12.3
(c) 10.8
(d) 3.2
Ans: (c)
Ammonia is a weak base, meaning that we will require an ICE table in order to determine the pH of the solution.
Let's look at the dissociation of ammonia:
I: Before the reaction proceeds, ammonia has a concentration of 0.02M. No product has yet been made.
C: When the reaction is at equilibrium, the products will increase by a concentration of x. Conversely, ammonia's concentration will decrease by the same amount, x.
E: By setting the equilibrium expression equal to the base dissociation constant, we can solve for the value of x.
Note: because the value for x will be so much less than the initial base concentration, we can omit it from the denominator: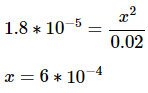
Keep in mind that x is equal to the concentration of hydroxide ions now in the solution.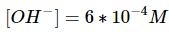
Use this value in the equation for pOH: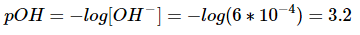
Remember that the sum of pH and pOH is always 14. To find the pH, subtract the pOH from 14.
pH + pOH = 14 → pH = 14 − pOH
pH = 14 − 3.2 = 10.8
Q6: There are __________ hydrogen ions in a solution with a pH of 3 than in a solution with a pH of 6.
(a) 100 times more
(b) 100 times less
(c) 1000 times less
(d) 1000 times more
Ans: (d)
Each whole number on the pH scale represents a factor of ten difference in concentration of hydrogen ions. We con verify this by finding the hydrogen ion concentrations for the two given pH values.
pH = −log[H+]
3 = −log(x) → x = 10−3
6 = −log(x) → x = 10−6
If we take the ratio of these values, we can see that there is a difference of 1000-times more protons in the solution with a pH of 3.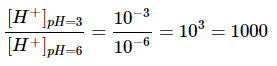
Q7: What is the pOH of a solution containing a hydrogen ion concentration of 1∗10−8M?
(a) 0.8
(b) 6
(c) 8
(d) -8
Ans: (b)
We can find the pOH by first finding the pH.
pH = −log[H+]
Use the given hydrogen ion concentration to find the pH:
pH = −log(1∗10−8) = 8
We know that pH and pOH will always sum to equal 14, allowing us to find the pOH.
pH + pOH = 14
pOH = 14 − pH = 14 − 8 = 6
Q8: A sample of gastric juice has a pH of 2.5. What is the hydrogen ion concentration in this secretion?
(a) 0.005M
(b) 0.03M
(c) 0.0025M
(d) 0.003M
Ans: (d)
pH = −log[H+]
The concentration of hydrogen ions must lie somewhere between 10−3M and 10−2M; alternatively stated, it is between 1∗10−3M and 10∗10−3M. The pH of a solution with hydrogen ion concentration of 10−3M will be 3, and the pH of a solution with hydrogen ion concentration 10−2M will be 2; thus, our concentration must lie between these two values, since our pH is 2.5
2.5 = 3.0−0.5
To find the exact concentration, you must be familiar with the logarithmic scale. A difference of 0.5 is equivalent to a log of 3.
log(3) = 0.48
Our answer must therefore be 3∗10−3M, or 0.003M.
We can calculate the pH in reverse to check our answer.
−log(3∗10−3)
−(log(3)+log(10−3))
−(0.48+(−3))
−(−2.52)
2.52
Q9: What is the pOH of a 6∗10−7M aqueous solution of HCl?
(a) 8.9
(b) 5.2
(c) 7.8
(d) 6.2
Ans: (c)
The first step for this problem is to find the pH. We can then derive the pOH from the pH value.
The pH is given by the equation pH=−log[H+]. Since hydrochloric acid is monoprotic, the concentration of the solution is equal to the concentration of protons.
[HCl] = [H+] = 6∗10−7M
Using this value and the pH equation, we can calculate the pH.
pH = −log(6∗10−7) = 6.2
Now we can find the pOH. The sum of the pH and the pOH is always 14.
pH + pOH = 14
pOH = 14−pH
pOH = 14−6.2 = 7.8
The pOH of the solution is 7.8.
Alternatively, a shortcut can be used to estimate the pH. If [H+] is in the form a∗10−b, then pH is roughly (b − 1).(10 − a).
(7 − 1).(10 − 6) = 6.4
For this question, this shortcut gets us a pH of 6.4, which produces a pOH of 7.6; very close to the real answer!
Q10: Acids and bases can be described in three principal ways. The Arrhenius definition is the most restrictive. It limits acids and bases to species that donate protons and hydroxide ions in solution, respectively. Examples of such acids include HCl and HBr, while KOH and NaOH are examples of bases. When in aqueous solution, these acids proceed to an equilibrium state through a dissociation reaction.
HA ⇋ H+ + A-
All of the bases proceed in a similar fashion.
BOH ⇋ B+ + OH-
The Brønsted-Lowry definition of an acid is a more inclusive approach. All Arrhenius acids and bases are also Brønsted-Lowry acids and bases, but the converse is not true. Brønsted-Lowry acids still reach equilibrium through the same dissociation reaction as Arrhenius acids, but the acid character is defined by different parameters. The Brønsted-Lowry definition considers bases to be hydroxide donors, like the Arrhenius definition, but also includes conjugate bases such as the A- in the above reaction. In the reverse reaction, A- accepts the proton to regenerate HA. The Brønsted-Lowry definition thus defines bases as proton acceptors, and acids as proton donors.
The pH of a solution of HA is lowered from 4 to 3, and then from 3 to 2. Which of the following is the most accurate description of what happens during these transitions?
(a) There are 100 times more protons in solution at pH 2 than at pH 4.
(b) There are 100 times fewer protons in solution at pH 2 than at pH 4.
(c) There are 20 times more hydroxide ions in solution at pH 2 than at pH 4.
(d) There are 20 times more protons in solution at pH 2 than at pH 4.
Ans: (a)
The pH scale is logarithmic. Every pH unit drop corresponds to a tenfold increase in protons.
pH = −log[H+]
2 = −log(0.01)
4 = −log(0.0001)
|
213 videos|209 docs|162 tests
|
















