Chapter 3 - Pair Of Linear Equations In Two Variables, RD Sharma Solutions - (Part-22) | RD Sharma Solutions for Class 10 Mathematics PDF Download
Page No 3.92
Q.8. Father's age is three times the sum of age of his two children. After 5 years his age will be twice the sum of ages of two children. Find the age of father.
Ans. Let the present age of father be x years and the present ages of his two children’s be y and z years.
The present age of father is three times the sum of the ages of the two children’s. Thus, we have
x = 3(y + z)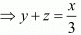
After 5 years, father’s age will be (x + 5) years and the children’s age will be (y + 5) and (z + 5) years. Thus using the given information, we have
x + 5 = 2(y + 5) + (z + 5)
⇒ x + 5 = 2(y + 5 + z + 5)
⇒ x = 2(y + z) + 20 - 5
⇒ x = 2(y + z) + 15
So, we have two equations
x = 2(y + z) + 15
Here x, y and z are unknowns. We have to find the value of x.
Substituting the value of (y + z) from the first equation in the second equation, we have
By using cross-multiplication, we have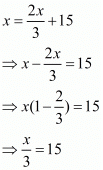
⇒ x = 15 x 3
⇒ x = 45
Hence, the present age of father is 45 years.
Q.9. Two years ago, a father was five times as old as his son. Two year later, his age will be 8 more than three times the age of the son. Find the present ages of father and son.
Ans. Let the present age of father be x years and the present age of his son be y years.
After 2 years, father’s age will be (x + 2) years and the age of son will be (x + 2) years. Thus using the given information, we have
x + 2 = 3(y + 2) + 8
⇒ x + 2 = 3y + 6 + 8
⇒ x - 3y - 12 = 0
Before 2 years, the age of father was (x - 2) years and the age of son was (y - 2) years. Thus using the given information, we have
x - 2 = 5(y - 2)
⇒ x - 2 = 5y - 10
⇒ x - 5y + 8 = 0
So, we have two equations
x - 3y - 12 = 0
x - 5y + 8 = 0
Here x and y are unknowns. We have to solve the above equations for x and y.
By using cross-multiplication, we have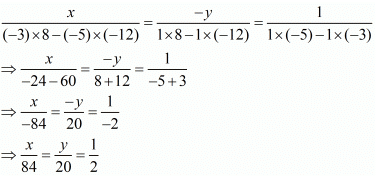
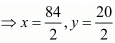
⇒ x = 42, y = 10
Hence, the present age of father is 42 years and the present age of son is 10 years.
Q.10. A is elder to B by 2 years. A's father F is twice as old as A and B is twice as old as his sister S. If the ages of the father and sister differ by 40 years, find the age of A.
Ans. Let the present ages of A, B, F and S be x, y, z and t years respectively.
A is elder to B by 2 years. Thus, we have x = y + 2
F is twice as old as A. Thus, we have z = 2x
B is twice as old as S. Thus, we have y = 2t
The ages of F and S is differing by 40 years. Thus, we have z - t = 40
So, we have four equations
x = y + 2, ......(1)
z = 2x, ......(2)
y = 2t, ......(3)
z - t = 40 ......(4)
Here x, y, z and t are unknowns. We have to find the value of x.
By using the third equation, the first equation becomes x = 2t + 2
From the fourth equation, we have t = z - 40
Hence, we have
x = 2(z - 40