Class 12 Exam > Class 12 Notes > Chapter 4 -Elasticity of Demand Economics
Chapter 4 -Elasticity of Demand Economics - Class 12 PDF Download
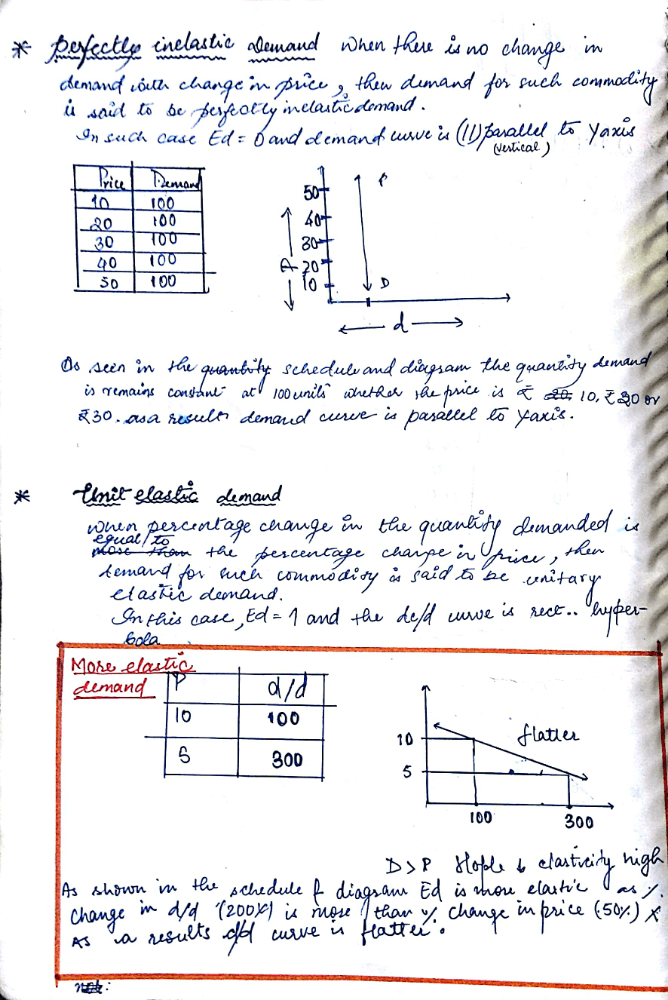
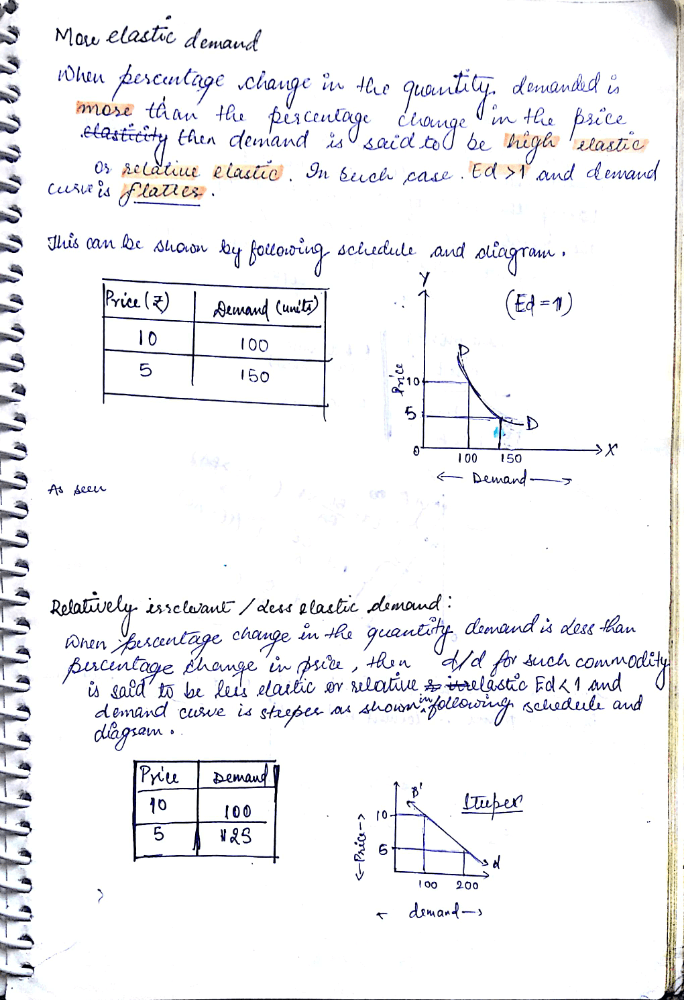
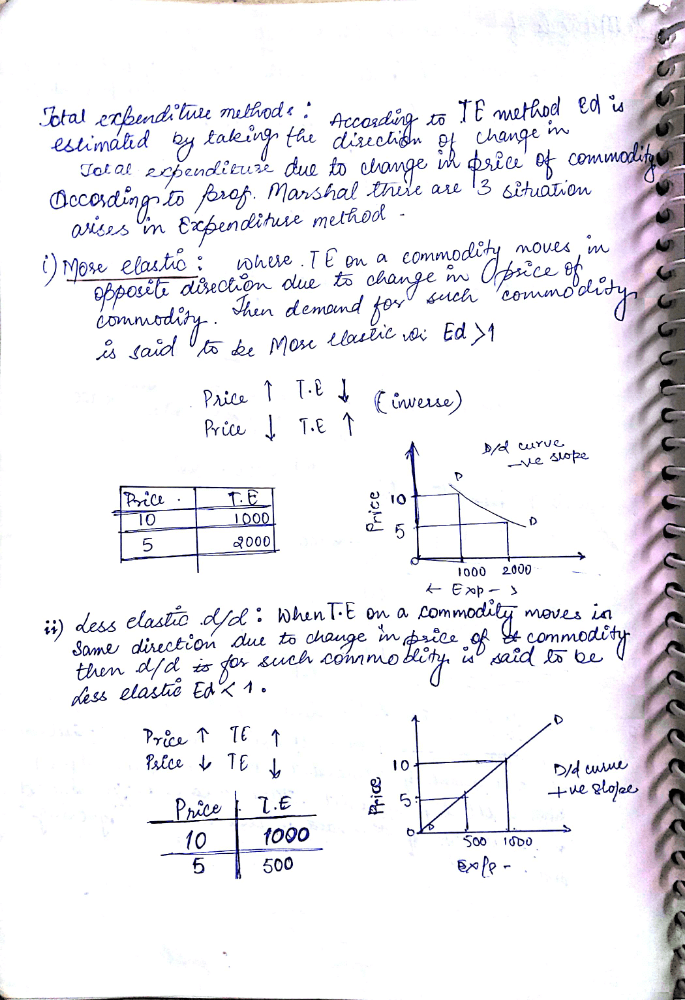
This is Hand written note of class 12 Micro-Economics chapter 4 elasticity of demand . It could be complete note. summary-:
Introduction Important Questions for Class 12 Economics,Concept of Price Elasticity of Demand and Its Determinants
Sample question with explanations---:
Elastic demand: Pepsi, chocolate, and Oriental rugs
Inelastic demand: Home heating oil, water, and heart medication
Inelastic demand: Home heating oil, water, and heart medication
Problem : If supply is unit elastic and demand is inelastic, a shift in which curve would affect quantity more? Price more?
Shifting the demand curve would affect quantity more, and shifting the supply curve would affect price more.Problem : Katherine advertises to sell cookies for $4 a dozen. She sells 50 dozen, and decides that she can charge more. She raises the price to $6 a dozen and sells 40 dozen. What is the elasticity of demand? Assuming that the elasticity of demand is constant, how many would she sell if the price were $10 a box?
To find the elasticity of demand, we need to divide the percent change in quantity by the percent change in price.% Change in Quantity = (40 - 50)/(50) = -0.20 = -20%
% Change in Price = (6.00 - 4.00)/(4.00) = 0.50 = 50%
Elasticity = |(-20%)/(50%)| = |-0.4| = 0.4
The elasticity of demand is 0.4 (elastic).
To find the quantity when the price is $10 a box, we use the same formula:
Elasticity = 0.4 = |(% Change in Quantity)/(% Change in Price)|
% Change in Price = (10.00 - 4.00)/(4.00) = 1.5 = 150%
Remember that before taking the absolute value, elasticity was -0.4, so use -0.4 to calculate the changes in quantity, or you will end up with a big increase in consumption, instead of a decrease!
-0.4 = |(% Change in Quantity)/(150%)|
|(%Change in Quantity)| = -60% = -0.6
-0.6 = (X - 50)/50
X = 20
The new demand at $10 a dozen will be 20 dozen cookies.
Follow for more such notes
FAQs on Chapter 4 -Elasticity of Demand Economics - Class 12
| 1. What is the concept of elasticity of demand in economics? |  |
Ans. Elasticity of demand refers to the responsiveness of the quantity demanded of a product or service to a change in its price. It measures the sensitivity of demand to price changes and helps in understanding consumer behavior and market dynamics.
| 2. How is the price elasticity of demand calculated? | |
Ans. Price elasticity of demand is calculated by dividing the percentage change in quantity demanded by the percentage change in price. The formula for price elasticity of demand is:
Elasticity = (% change in quantity demanded) / (% change in price)
If the elasticity value is greater than 1, demand is considered elastic; if it is less than 1, demand is inelastic; and if it is equal to 1, demand is unitary elastic.
| 3. What factors determine the elasticity of demand? | |
Ans. The elasticity of demand is influenced by several factors, including the availability of substitutes, the necessity of the good or service, the proportion of income spent on the good, and the time period under consideration. Goods with close substitutes, luxury items, and goods that consume a large portion of income are usually more elastic in nature.
| 4. How does elasticity of demand affect pricing decisions for businesses? | |
Ans. The elasticity of demand plays a crucial role in pricing decisions for businesses. If the demand for a product is elastic, meaning that a small change in price leads to a significant change in quantity demanded, businesses may need to lower their prices to attract more customers and increase sales. On the other hand, if the demand is inelastic, businesses can increase prices without affecting the quantity demanded significantly.
| 5. What are some real-life examples of elastic and inelastic demand? | |
Ans. Examples of goods with elastic demand include luxury items like designer clothing, high-end smartphones, and vacation packages. When the prices of these goods increase, consumers tend to reduce their quantity demanded significantly. In contrast, goods with inelastic demand are typically necessities like food, medication, and basic utilities. Even if the prices of these goods increase, consumers still require them and are willing to pay the higher prices, resulting in a relatively smaller decrease in quantity demanded.
Related Exams
About this Document
|
1.1K Views |
|
4.98/5 Rating |
|
Nov 29, 2024 Last updated |
Document Description: Chapter 4 -Elasticity of Demand Economics for Class 12 2024 is part of Class 12 preparation. The notes and questions for Chapter 4 -Elasticity of Demand Economics have been prepared according to the Class 12 exam syllabus. Information about Chapter 4 -Elasticity of Demand Economics covers topics like Introduction Important Questions for Class 12 Economics,Concept of Price Elasticity of Demand and Its Determinants and Chapter 4 -Elasticity of Demand Economics Example, for Class 12 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises and tests below for Chapter 4 -Elasticity of Demand Economics.
Introduction of Chapter 4 -Elasticity of Demand Economics in English is available as part of
our Class 12 preparation & Chapter 4 -Elasticity of Demand Economics in Hindi for Class 12
courses. Download more important topics, notes, lectures and mock test series for Class 12
Exam by signing up for free. Class 12: Chapter 4 -Elasticity of Demand Economics - Class 12
Description
Full syllabus notes, lecture & questions for Chapter 4 -Elasticity of Demand Economics - Class 12 - Class 12 | Plus excerises question with solution to help you revise complete syllabus | Best notes, free PDF download
Information about Chapter 4 -Elasticity of Demand Economics
In this doc you can find the meaning of Chapter 4 -Elasticity of Demand Economics defined & explained in the simplest way possible.
Besides explaining types of Chapter 4 -Elasticity of Demand Economics theory,
EduRev gives you an ample number of questions to practice Chapter 4 -Elasticity of Demand Economics tests, examples and also practice Class 12 tests.
Download as PDF
|
Explore Courses for Class 12 exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
Related Searches
video lectures
,Viva Questions
,Chapter 4 -Elasticity of Demand Economics - Class 12
,Objective type Questions
,study material
,past year papers
,Summary
,shortcuts and tricks
,MCQs
,Chapter 4 -Elasticity of Demand Economics - Class 12
,Extra Questions
,Previous Year Questions with Solutions
,Semester Notes
,practice quizzes
,Free
,Exam
,Chapter 4 -Elasticity of Demand Economics - Class 12
,mock tests for examination
,Important questions
,Sample Paper
,ppt
;
Additional Information about Chapter 4 -Elasticity of Demand Economics for Class 12 Preparation
Chapter 4 -Elasticity of Demand Economics Free PDF Download
The Chapter 4 -Elasticity of Demand Economics is an invaluable resource that delves deep into the core of the Class 12 exam.
These study notes are curated by experts and cover all the essential topics and concepts, making your preparation more efficient and effective.
With the help of these notes, you can grasp complex subjects quickly, revise important points easily,
and reinforce your understanding of key concepts. The study notes are presented in a concise and easy-to-understand manner,
allowing you to optimize your learning process. Whether you're looking for best-recommended books, sample papers, study material,
or toppers' notes, this PDF has got you covered. Download the Chapter 4 -Elasticity of Demand Economics now and kickstart your journey towards success in the Class 12 exam.
Importance of Chapter 4 -Elasticity of Demand Economics
The importance of Chapter 4 -Elasticity of Demand Economics cannot be overstated, especially for Class 12 aspirants.
This document holds the key to success in the Class 12 exam.
It offers a detailed understanding of the concept, providing invaluable insights into the topic.
By knowing the concepts well in advance, students can plan their preparation effectively.
Utilize this indispensable guide for a well-rounded preparation and achieve your desired results.
Chapter 4 -Elasticity of Demand Economics Notes
Chapter 4 -Elasticity of Demand Economics Notes offer in-depth insights into the specific topic to help you master it with ease.
This comprehensive document covers all aspects related to Chapter 4 -Elasticity of Demand Economics.
It includes detailed information about the exam syllabus, recommended books, and study materials for a well-rounded preparation.
Practice papers and question papers enable you to assess your progress effectively.
Additionally, the paper analysis provides valuable tips for tackling the exam strategically.
Access to Toppers' notes gives you an edge in understanding complex concepts.
Whether you're a beginner or aiming for advanced proficiency, Chapter 4 -Elasticity of Demand Economics Notes on EduRev are your ultimate resource for success.
Chapter 4 -Elasticity of Demand Economics Class 12 Questions
The "Chapter 4 -Elasticity of Demand Economics Class 12 Questions" guide is a valuable resource for all aspiring students preparing for the
Class 12 exam. It focuses on providing a wide range of practice questions to help students gauge
their understanding of the exam topics. These questions cover the entire syllabus, ensuring comprehensive preparation.
The guide includes previous years' question papers for students to familiarize themselves with the exam's format and difficulty level.
Additionally, it offers subject-specific question banks, allowing students to focus on weak areas and improve their performance.
Study Chapter 4 -Elasticity of Demand Economics on the App
Students of Class 12 can study Chapter 4 -Elasticity of Demand Economics alongwith tests & analysis from the EduRev app,
which will help them while preparing for their exam. Apart from the Chapter 4 -Elasticity of Demand Economics,
students can also utilize the EduRev App for other study materials such as previous year question papers, syllabus, important questions, etc.
The EduRev App will make your learning easier as you can access it from anywhere you want.
The content of Chapter 4 -Elasticity of Demand Economics is prepared as per the latest Class 12 syllabus.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup