Cubes & Cube Roots Chapter Notes | Mathematics (Maths) Class 8 PDF Download
| Table of contents |

|
| Introduction |

|
| Cubes |

|
| Some Interesting Patterns |

|
| Cube Roots |

|
| Cube Root Through Prime Factorization Method |

|
| What we have discussed |

|
Introduction
This story is about S. Ramanujan, one of India’s greatest mathematical minds. When Prof. G.H. Hardy once visited him in a taxi with the number 1729, Hardy called the number "dull."
Ramanujan quickly corrected him, noting that 1729 is actually the smallest number expressible as the sum of two cubes in two different ways.
- The cube of 10 plus the cube of 9: (103 + 93 = 1729)
- The cube of 12 plus the cube of 1: (123 + 13 = 1729)

This number, now known as the Hardy–Ramanujan Number, had been recognized long before, but Ramanujan's deep love for numbers led him to discover such fascinating properties.
Cubes
Word cube is used in geometry. It is a solid figure which all sides are equal.
Consider a cube of side 3 cm , How many cubes can be made of side 1cm from 3cm side cube!
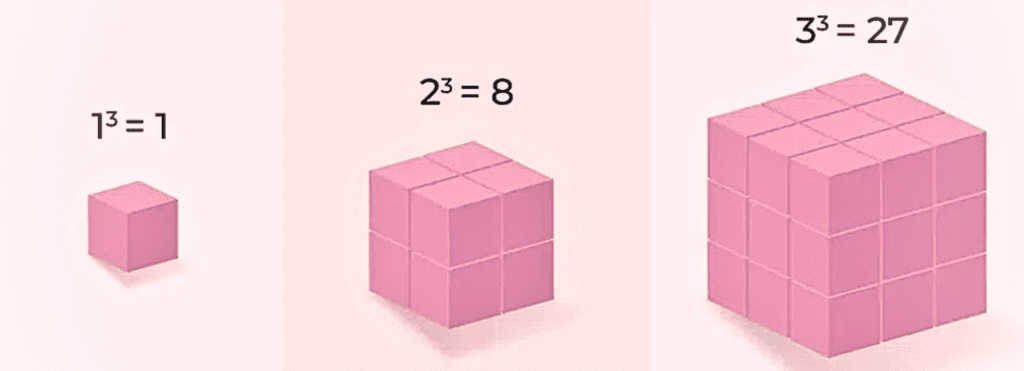
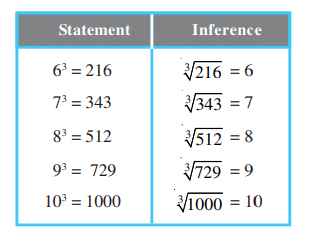
Cube of any number is obtained when the number is multiplied 3 times in a row.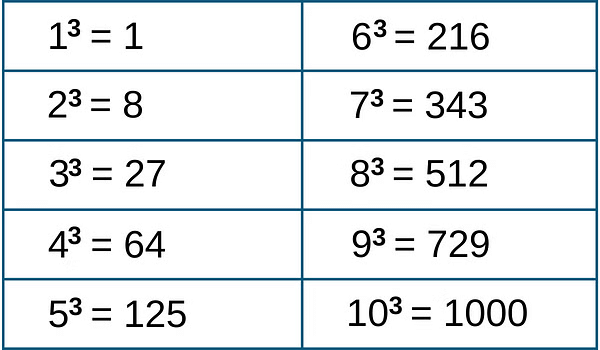 Cubes till 10
Cubes till 10
Perfect Cube: A perfect cube is a number that you get when you multiply a whole number by itself two more times.
A number of the form n3 where n is an integer.
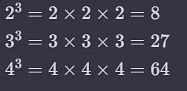
8, 27, 64 are perfect cubes.
There are only ten perfect cubes from 1 to 1000. Cube of any odd integer is odd and cube of any even integer is even.
Some Interesting Patterns
1. Adding Consecutive Odd Numbers
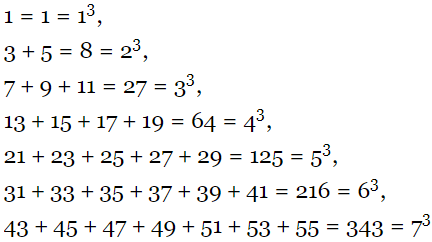
Number of consecutive odd number to add to get "n3" = n
2. Cubes and Their Prime Factors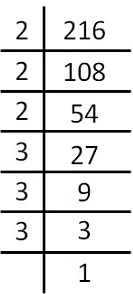
216 = 2 x 2 x 2 x 3 x 3 x3
Since each factor appears 3 times. 216 = (23 × 33) = 63 because an x bn = (a × b)n
Example: Is 354 a perfect cube?
Sol: 354= 2×3×59
In the above factorization 2×3×59 remain.Therefore, 354 is not a perfect cube.
Smallest Multiple that is a perfect cube
Example : Is 392 a perfect cube? If not, find the smallest natural number by which 392 must be multiplied so that the product is a perfect cube.
Sol: 392 = 2 × 2 × 2 × 7 × 7 The prime factor 7 does not appear in a group of three. Therefore, 392 is not a perfect cube. To make its a cube, we need one more 7. In that case 392 × 7 = 2 × 2 × 2 × 7 × 7 × 7 = 2744 which is a perfect cube. Hence the smallest natural number by which 392 should be multiplied to make a perfect cube is 7.
Cube Roots
Lets understand an example -
There is a cube of volume 125 cm3 . Since all the side of cube is equal we want to find the length of our cube.
Volume of cube = (side of cube)3 unit3. ------------------------------------(1)
Side of cube = (Volume of cube)1/3.
Hence the side of any cube is nothing but a cube root of the volume of that cube.
How to denote cube root in mathematics.
Let's understand: (25)1/3 = (53)1/3 = 5.
Hence let's take an integer x then 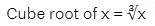
Cube Root Through Prime Factorization Method
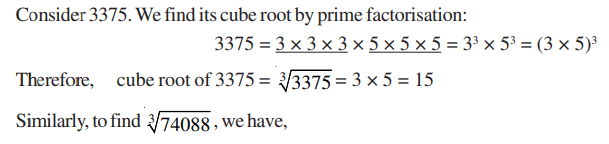
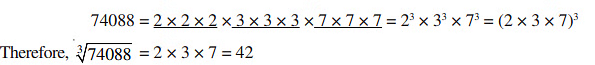
Example : Find the cube root of 8000.
Sol: Prime factorization of 8000 is 2 × 2 × 2 × 2 × 2 × 2 × 5 × 5 × 5
So,
= 2 × 2 × 5 = 20
What we have discussed
- Numbers like 1729, 4104, 13832, are known as Hardy – Ramanujan Numbers. They can be expressed as sum of two cubes in two different ways.
- Numbers obtained when a number is multiplied by itself three times are known as cube numbers. For example 1, 8, 27, ... etc.
- If in the prime factorization of any number each factor appears three times, then the number is a perfect cube.
- The symbol
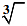 denotes cube root. For example
denotes cube root. For example 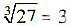 Question for Chapter Notes: Cubes & Cube RootsTry yourself:Which of the following numbers is a perfect cube?View Solution
Question for Chapter Notes: Cubes & Cube RootsTry yourself:Which of the following numbers is a perfect cube?View Solution
|
87 videos|455 docs|31 tests
|
FAQs on Cubes & Cube Roots Chapter Notes - Mathematics (Maths) Class 8
| 1. What is the definition of a cube in mathematics? |  |
| 2. How do you calculate the cube of a number? |  |
| 3. What are cube roots, and how are they related to cubes? |  |
| 4. How can prime factorization be used to find the cube root of a number? |  |
| 5. What patterns can be observed in the cubes of consecutive natural numbers? |  |





















