Circular Motion, Chapter Notes, Class 11, Physics (IIT-JEE & AIPMT) PDF Download
Circular Motion - Work, Energy and Power, Class 11, Physics
Circular Motion
When a particle moves in a plane such that its distance from a fixed (or moving) point remains constant then its motion is called as the circular motion with respect to that fixed (or moving) point. That fixed point is called centre and the distance between fixed point and particle is called radius.
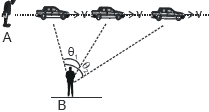
The car is moving in a straight line with respect to the man A. But the man B continuously rotate his face to see the car. So with respect to man 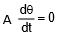
But with respect to man B 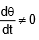
Therefore we conclude that with respect to A the motion of car is straight line but for man B it has some angular velocity
2. Kinematics of Circular Motion :
2.1 Variables of Motion :
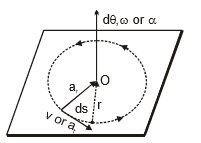
(a) Angular Position :
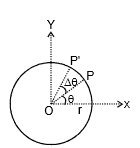
The angle made by the position vector with given line (reference line) is called angular position Circular motion is a two dimensional motion or motion in a plane.
Suppose a particle P is moving in a circle of radius r and centre O. The position of the particle P at a given instant may be described by the angle q between OP and OX. This angle q is called the angular position of the particle. As the particle moves on the circle its angular position q change. Suppose the point rotates an angle Dq in time Dt.
(b) Angular Displacement :
Definition :
Angle rotated by a position vector of the moving particle in a given time interval with some reference line is called its angular displacement.
Important point :
- It is dimensionless and has proper unit SI unit radian while other units are degree or revolution 2p rad = 360° = 1 rev
- Infinitely small angular displacement is a vector quantity but finite angular displacement is not because the addition of the small angular displacement is cummutative while for large is not.
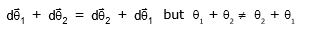
- Direction of small angular displacement is decided by right hand thumb rule. When the fingers are directed along the motion of the point then thumb will represents the direction of angular displacement.
- Angular displacement can be different for different observers
(c) Angular Velocity w

Important points :
- It is an axial vector with dimensions [T-1] and SI unit rad/s.
- For a rigid body as all points will rotate through same angle in same time, angular velocity is a characteristic of the body as a whole, e.g., angular velocity of all points of earth about its own axis is (2p/24) rad/hr.
- If a body makes `n' rotations in `t' seconds then angular velocity in radian per second will be
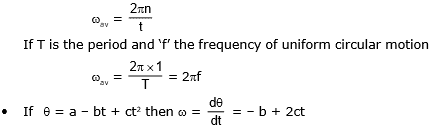
Relation between speed and angular velocity :
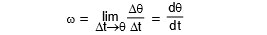
The rate of change of angular velocity is called the angular acceleration (a). Thus,
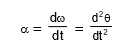
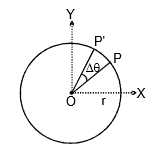
The linear distance PP' travelled by the particle in time Dt is

Ex.1 If q depends on time t in following way θ = 2t2 + 3 then
(a) Find out w average upto 3 sec.
(b) ω at 3 sec
Sol.

(d) Relative Angular Velocity
Angular velocity is defined with respect to the point from which the position vector of the moving particle is drawn Here angular velocity of the particle w.r.t. `O' and `A' will be different
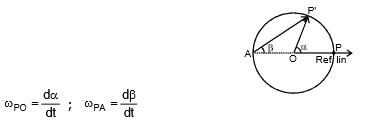
Definition :
Relative angular velocity of a particle `A' with respect to the other moving particle `B' is the angular velocity of the position vector of `A' with respect to `B'. That means it is the rate at which position vector of `A' with respect to `B' rotates at that instant
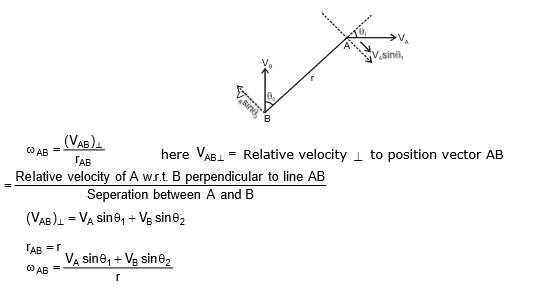
Important points :
If two particles are moving on the same circle or different coplanar concentric circles in same direction with different uniform angular speed wA and wB respectively, the rate of change of angle between and is
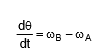
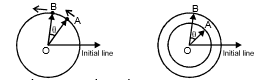
So the time taken by one to complete one revolution around O w.r.t. the other
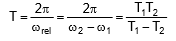
If two particles are moving on two different concentric circles with different velocities then angular velocity of B relative to A as observed by A will depend on their positions and velocities. consider the case when A and B are closest to each other moving in same direction as shown in figure. In this situation

Ex.2 Two particles move on a circular path (one just inside and the other just outside) with angular velocities w and 5 w starting from the same point. Then, which is incorrect.
(a) they cross each other at regular intervals of time  when their angular velocities are oppositely directed
when their angular velocities are oppositely directed
(b) they cross each other at points on the path subtending an angle of 60° at the centre if their angular velocities are oppositely directed
(c) they cross at intervals of time  if their angular velocities are oppositely directed
if their angular velocities are oppositely directed
(d) they cross each other at points on the path subtending 90° at the centre if their angular velocities are in the same sense
Sol. If the angular velocities are oppositely directed, they meet at intervals of time
t =  =
=  =
= 
Angle subtended at the centre by the crossing points
θ = wt =  = 60°
= 60°
When their angular velocities are in the same direction,
t' =  =
=  =
=  and q' =
and q' =  =
= 
Ans. (a)
Ex.3 Two moving particles P and Q are 10 m apart at a certain instant. The velocity of P is 8 m/s making 30° with the line joining P and Q and that of Q is 6 m/s making 30° with PQ in the figure. Then the angular velocity of Q with respect to P in rad/s at that instant is
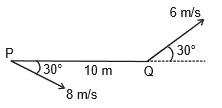
(A) 0
(B) 0.1
(C) 0.4
(D) 0.7
Sol.
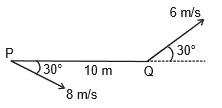
Angular velocity of Q relative to P = 

(D)
(e) Angular Acceleration a :
(i) Average Angular Acceleration :

(ii) Instantaneous Angular Acceleration :
It is the limit of average angular acceleration as Dt approaches zero, i.e.,
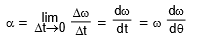
Important points :
- It is also an axial vector with dimension [T-2] and unit rad/s2
- If a = 0, circular motion is said to be uniform.
 i.e., second derivative of angular displacement w.r.t time gives angular acceleration.
i.e., second derivative of angular displacement w.r.t time gives angular acceleration.- a is a axial vector and direction of a is along w if w increases and opposite to w if w decreases
(f) Radial and tangential acceleration
Acceleration of a particle moving in a circle has two components one is along  (along tangent) and the other along
(along tangent) and the other along  (or towards centre). Of these the first one is the called the tangential acceleration. (at) and the other is called radial or centripetal acceleration (ar). Thus.
(or towards centre). Of these the first one is the called the tangential acceleration. (at) and the other is called radial or centripetal acceleration (ar). Thus.
 = rate of change of speed
= rate of change of speed
and 

Here, at is the component which is responsible for changing the magnitude of speed of the particle in circular motion. ar is the component which is responsible for changing the direction of particle in circular motion.
the two component are mutually perpendicular. Therefore, net acceleration of the particle will be :
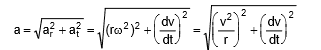
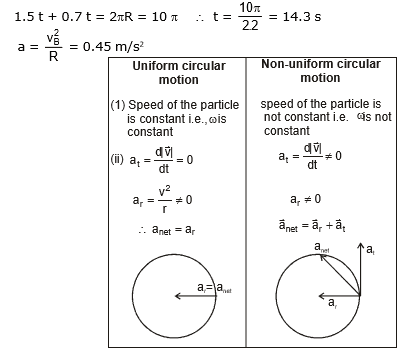
Following three points are important regarding the above discussion :
1. In uniform circular motion, speed (v) of the particle is constant, i.e.,  =0. Thus,
=0. Thus,
at = 0 and a = ar = rw2
2. In accelerated circular motion, 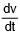 = positive, i.e., at is along
= positive, i.e., at is along  or tangential acceleration of particle is parallel to velocity
or tangential acceleration of particle is parallel to velocity  because
because 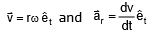
3. In decelerated circular motion, 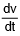 = negative and hence, tangential acceleration is anti-parallel to velocity
= negative and hence, tangential acceleration is anti-parallel to velocity  .
.
(g) Relation between angular acceleration and tangential acceleration
we know that
v = rw
Here, v is the linear speed of the particle
Differentiating again with respect to time, we have

Here, at =  is the rate of change of speed (not the rate of change of velocity).
is the rate of change of speed (not the rate of change of velocity).
Ex.4 A particle travels in a circle of radius 20 cm at a speed that uniformly increases. If the speed changes from 5.0 m/s to 6.0 m/s in 2.0s, find the angular acceleration.
Sol. The tangential acceleration is given by
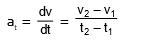
( Here speed increases uniformly at =
Here speed increases uniformly at =  =
=  )
)
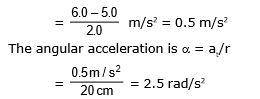
Ex-5 A particle moves in a circle of radius 20 cm. Its linear speed at any time is given by v = 2t where v is in m/s and t is in seconds. Find the radial and tangential acceleration at t = 3 seconds and hence calculate the total acceleration at this time.
Sol. The linear speed at 3 seconds is
v = 2 × 3 = 6 m/s
The radial acceleration at 3 seconds
=  =
=  = 180 m/s2
= 180 m/s2
The tangential acceleration is given by
 , because v = 2t.
, because v = 2t.
tangential acceleration is 2 m/s2.
Net Acceleration =  =
=  = 180.01 m/s2
= 180.01 m/s2
T-1 Is it possible for a car to move in a circular path in such a way that it has a tangential acceleration but no centripetal acceleration ?
Ex.6 A particle moves in a circle of radius 2.0 cm at a speed given by v = 4t, where v is in cm/s and t in seconds.
(a) Find the tangential acceleration at t = 1 s.
(b) Find total accleration at t = 1 s.
Sol. (a) Tangential acceleration
at =  or at =
or at =  = 4 cm/s2
= 4 cm/s2
ac =  =
=  = 8 ⇒ a =
= 8 ⇒ a =  =
=  =
= 
Ex.7 A boy whirls a stone in a horizontal circle of radius 1.5 m and at height 2.0 m above level ground. The string breaks, and the stone files off horizontally and strikes the ground after traveling a horizontal distance of 10 m. What is the magnitude of the cetripetal acceleration of the stone while in circular motion ?
Sol.
t =  =
=  = 0.64 s
= 0.64 s
v =  = 15.63 m/s
= 15.63 m/s
a =  = 0.45 m/s2
= 0.45 m/s2
Ex.8 Find the magnitude of the acceleration of a particle moving in a circle of radius 10 cm with uniform speed completing the circle in 4 s.
Sol. The distance covered in completing the circle is 2 p r = 2p × 10 cm. The linear speed is
v = 2 p r/t =  = 5 p cm/s.
= 5 p cm/s.
The acceleration is ar = =
= =2.5 p2 cm/s2
=2.5 p2 cm/s2
Ex.9 A particle moves in a circle of radius 20 cm. Its linear speed is given by v = 2t where t is in second and v in meter/second. Find the radial and tangential acceleration at t = 3s.
Sol. The linear speed at t = 3s is
v = 2t = 6 m/s
The radial acceleration at t = 3s is
ar = v2/r =  = 180 m/s2
= 180 m/s2
The tangential acceleration is
at =  =
=  = 2 m/s2
= 2 m/s2
Ex.10 Two particles A and B start at the origin O and travel in opposite directions along the circular path at constant
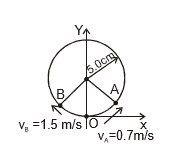
speeds vA = 0.7 m/s and vB = 1.5 m/s, respectively. Determine the time when they collide and the magnitude of the acceleration of B just before this happens.
Sol.
(h) Relations among Angular Variables
These relations are also referred as equations of rotational motion and are -
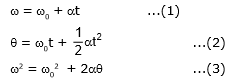
These are valid only if angular acceleration is constant and
are analogous to equations of translatory motion, i.e.,
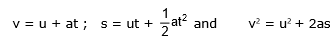
3. Dynamics of circular motion :
In circular motion or motion along any curved path Newton's law is applied in two perpendicular directions one along the tangent and other perpendicular to it. i.e., towards centre. The compnent of net force towards the centre is called centripetal force. The component of net force along the tangent is called tangential force.
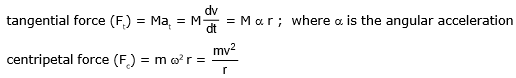
Ex.11 A small block of mass 100 g moves with uniform speed in a horizontal circular groove, with vertical side walls, of radius 25 cm. If the block takes 2.0s to complete one round, find the normal contact force by the slide wall of the groove.
Sol. The speed of the block is
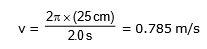
The acceleration of the block is
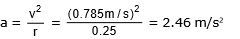
towards the center. The only force in this direction is the normal contact force due to the side walls. Thus from Newton's second law, this force is
N = ma = (0.100 kg) (2.46 m/s2) = 0.246 N
3.1 Centripetal Force :
Concepts : This is necessary resultant force towards the centre called the centripetal force.
F =  = mw2r
= mw2r
(i) A body moving with constant speed in a circle is not in equilibrium.
(ii) It should be remembered that in the absence of the centripetal force the body will move in a straight line with constant speed.
(iii) It is not a new kind of force which acts on bodies. In fact, any force which is directed towards the centre may provide the necessary centripetal force.
Ex.12 A small block of mass 100 g moves with uniform speed in a horizontal circular groove, with vertical side walls, of radius 25 cm. If the block takes 2.0s to complete one round, find the normal contact force by the slide wall of the groove.
Sol. The speed of the block is
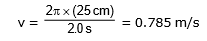
The acceleration of the block is
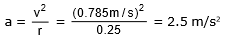
towards the center. The only force in this direction is the normal contact force due to the slide walls. Thus from Newton's second law, this force is
N = ma = (0.100 kg) (2.5 m/s2) = 0.25 N
======================================================
Centrifugal Force - Work, Energy and Power, Class 11, Physics
3.2 Centrifugal Force :
When a body is rotating in a circular path and the centripetal force vanishes, the body would leave the circular path. To an observer A who is not sharing the motion along the circular path, the body appears to fly off tangentially at the point of release. To another observer B, who is sharing the motion along the circular path (i.e., the observer B is also rotating with the body which is released, it appears to B, as if it has been thrown off along the radius away from the centre by some force. This inertial force is called centrifugal force.)
Its magnitude is equal to that of the centripetal force =  . Centrifugal force is a fictitious force which has to be applied as a concept only in a rotating frame of reference to apply Newton's law of motion in that frame)
. Centrifugal force is a fictitious force which has to be applied as a concept only in a rotating frame of reference to apply Newton's law of motion in that frame)
FBD of ball w.r.t non inertial frame rotating with the ball.

Suppose we are working from a frame of reference that is rotating at a constant, angular velocity w with respect to an inertial frame. If we analyse the dynamics of a particle of mass m kept at a distance r from the axis of rotation, we have to assume that a force mrw2 act radially outward on the particle. Only then we can apply Newton's laws of motion in the rotating frame. This radially outward pseudo force is called the centrifugal force.
T-2 A particle of mass m rotates in a circle of radius r with a uniform angular speed w. It is viewed from a frame rotating about same axis with a uniform angular speed w0. The centrifugal force on the particle is
(A) mw2r
(B) mw02r
(C) 
(D) mw0wr
 : A rod move with w angular velocity then we conclude
: A rod move with w angular velocity then we conclude
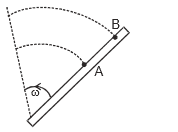
following for point A & B in a rod.
aA = aB sB > sA
qA = qB vB > vA
wA = wB atB > atA
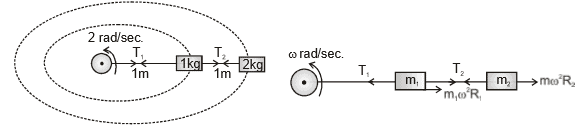
Ex.13 Find out the tension T1, T2 is the string as shown in figure
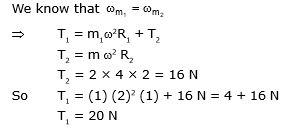
4. simple pendulum
Ex.14 A simple pendulum is constructed by attaching a bob of mass m to a string of length L fixed at its upper end. The bob oscillates in a vertical circle. It is found that the speed of the bob is v when the string makes an angle q with the vertical. Find the tension in the string at this instant.
Sol. The force acting on the bob are (figure)
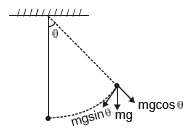
(a) the tension T
(b) the weight mg.
As the bob moves in a vertical circle with centre at O, the radial acceleration is v2/L towards O. Taking the
components along this radius and applying Newton's second law, we get
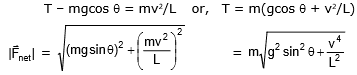
5. Circular Motion in Horizontal Plane
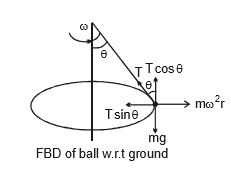
A ball of mass m attached to a light and inextensible string rotates in a horizontal circle of radius r with an angular speed w about the vertical. If we draw the force diagram of the ball. We can easily see that the component of tension force along the centre gives the centripetal force and component of tension along vertical balances the gravitation force. Such a system is called a conical pendulum.
Ex. 15 A particle of mass m is suspended from a ceiling through a string of length L. The particle moves in a horizontal circle of radius r. Find (a) the speed of the particle and (b) the tension in the string.
Sol. The situation is shown in figure.
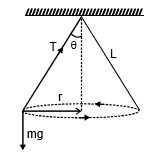
The angle q made by the string with the vertical is given by
sinq = r/L ... (i)
The forces on the particle are
(a) the tension T along the string and
(b) the weight mg vertically downward.
The particle is moving in a circle with a constant speed v. Thus, the radial acceleration towards the centre has magnitude v2/r. Resolving the forces along the radial direction and applying. Newton's second law,

6. Motion of a motorcyclist on a curved path.
A cylist having mass m move with constant speed v on a curved path as shown in figure.
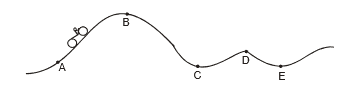
We divide the motion of cyclist in four parts :
(1) from A to B
(2) from B to C
(3) from C to D
(4) from D to E
(1 and 3 are same type of motion)
(A) Motion of cyclist from A to B
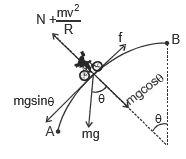
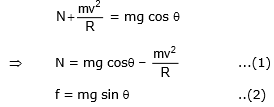
(1) As cyclist move upward
Q q decreases & cos q increases
N increases
and
Q q decreases sin q decreases
friction force required to balance mg sin q (As cyclist is moving with constant speed) also decreases
(B) Motion of cyclist from B to C
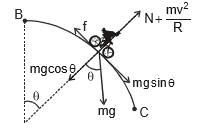
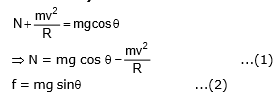
Therefore from B to C Normal force decrease but
friction force increase becuse q increases.
(C) Motion of cyclist from D to E
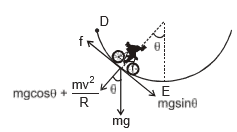
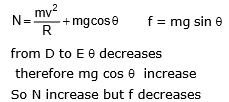
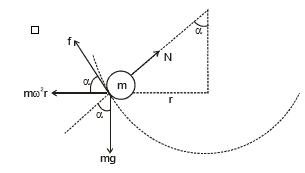
Ex.16 A hemispherical bowl of radius R is rotating about its axis of symmetry which is kept vertical. A small ball kept in the bowl rotates with the bowl without slipping on its surface. If the surface of the bowl is smooth and the angle made by the radius through the ball with the vertical is a. Find the angular speed at which the bowl is rotating.
Sol.
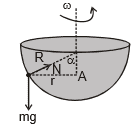
Let w be the angular speed of rotation of the bowl.
Two force are acting on the ball.
1. normal reaction N
2. weight mg
The ball is rotating in a circle of radius r (= R sin a) with centre at A at an angular speed w. Thus,
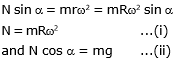
Dividing Eqs. (i) by (ii),
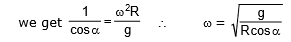
Ex.17 If friction is present between the surface of ball and bowl then find out the range of w for which ball does not slip (m is the friction coefficient)
Friction develop a range of w for which the particle will be at rest.
Sol.
(a) When w > w0
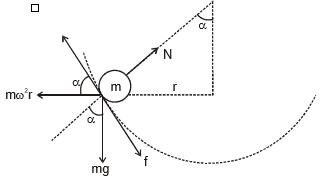
In this situation ball has a tendency to slip upwards so the friction force will act downwards. So F.B.D of ball
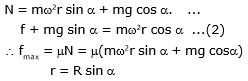
Substituting the values of fmax & r in eq. (2) we get
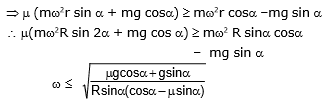
(b) when w < w0
In this situation ball has a tendency to slip downwards so the friction force will act upwards. So F.B.D of ball
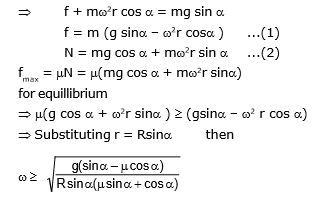
==============================================================
Circular Turning on roads - Work, Energy and Power, Class 11, Physics
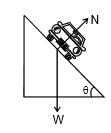
7. Circular Turning on roads :
When vehicles go through turnings, they travel along a nearly circular arc. There must be some force which will produce the required centripetal acceleration. If the vehicles travel in a horizontal circular path, this resultant force is also horizontal. The necessary centripetal force is being provided to the vehicles by following three ways.
1. By Friction only
2. By Banking of Roads only
3. By Friction and Banking of Roads both.
In real life the necessary centripetal force is provided by friction and banking of roads both. Now let us write equations of motion in each of the three cases separately and see what are the constant in each case.
7.1 By Friction Only
Suppose a car of mass m is moving at a speed v in a horizontal circular arc of radius r. In this case, the necessary centripetal force to the car will be provided by force of friction f acting towards center
Thus, 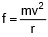
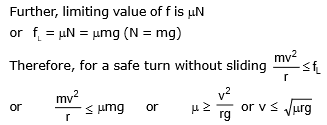
Here, two situations may arise. If m and r are known to us, the speed of the vehicle should not exceed 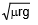 and if v and r are known to us, the coefficient of friction should be greater than
and if v and r are known to us, the coefficient of friction should be greater than 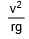 .
.
7.2 By Banking of Roads Only
Friction is not always reliable at circular turns if high speeds and sharp turns are involved to avoid dependence on friction, the roads are banked at the turn so that the outer part of the road is some what lifted compared to the inner part.
Applying Newton's second law along the radius and the first law in the vertical direction.
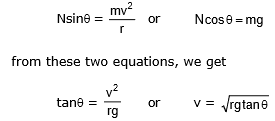
7.3 By Friction and Banking of Road Both
If a vehicle is moving on a circular road which is rough and banked also, then three forces may act on the vehicle, of these force, the first force, i.e., weight (mg) is fixed both in magnitude and direction.
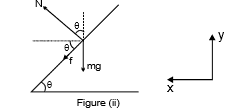
The direction of second force i.e., normal reaction N is also fixed (perpendicular or road) while the direction of the third i.e., friction f can be either inwards or outwards while its magnitude can be varied upto a maximum limit (fL = mN). So the magnitude of normal reaction N and directions plus magnitude of friction f are so adjusted that the resultant of the three forces mentioned above is  towards the center. Of these m and r are also constant. Therefore, magnitude of N and directions plus magnitude of friction mainly depends on the speed of the vehicle v. Thus, situation varies from problem to problem. Even though we can see that :
towards the center. Of these m and r are also constant. Therefore, magnitude of N and directions plus magnitude of friction mainly depends on the speed of the vehicle v. Thus, situation varies from problem to problem. Even though we can see that :
(i) Friction f will be outwards if the vehicle is at rest v = 0. Because in that case the component weight mg sinq is balanced by f.
(ii) Friction f will be inwards if
v > 
(iii) Friction f will be outwards if
v <  and
and
(iv) Friction f will be zero if
v = 
(v) For maximum safe speed (figure (ii)
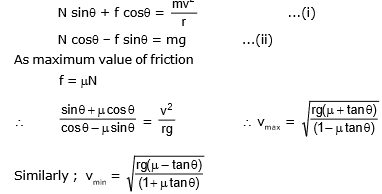
 : The expression tan q =
: The expression tan q =  also gives the angle of banking for an aircraft, i.e., the angle through which it should tilt while negotiating a curve, to avoid deviation from the circular path.
also gives the angle of banking for an aircraft, i.e., the angle through which it should tilt while negotiating a curve, to avoid deviation from the circular path. - The expression tan q =
 also gives the angle at which a cyclist should lean inward, when rounding a corner. In this case, q is the angle which the cyclist must make with the vertical to negotiate a safe turn.
also gives the angle at which a cyclist should lean inward, when rounding a corner. In this case, q is the angle which the cyclist must make with the vertical to negotiate a safe turn.
8. Death well :
A motor cyclist is driving in a horizontal circle on the inner surface of vertical cyclinder of radius R. Friction coefficient between tyres of motorcyclist and surface of cylinder is m. Find out the minimum velocity for which the motorcyclist can do this. v is the speed of motor cyclist and m is his mass.
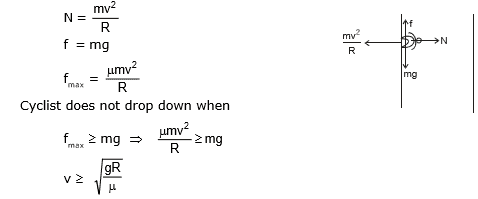
9. Motion of a cyclist on a circular path :
Suppose a cyclist is going at a speed v on a circular horizontal road of radius r which is not banked. Consider the cycle and the rider together as the system. The centre of mass C (figure shown) of the system is going in a circle with the centre at O and radius r.
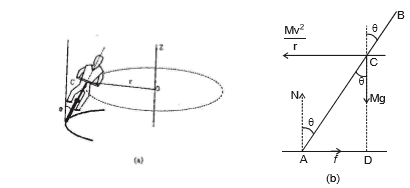
Let us choose O as the origin, OC as the X-axis and vertically upward as the Z-axis. This frame is rotating at an angular speed w =  about the Z-axis. In this frame the system is at rest. Since we are working from a rotating frame of reference, we will have to apply a centrifugal force on each particle. The net centrifugal force on the system will be Mw2r = Mv2/r, where M is the total mass of the system. This force will act through the centre of mass. Since the system is at rest in this frame, no other pseudo force is needed.
about the Z-axis. In this frame the system is at rest. Since we are working from a rotating frame of reference, we will have to apply a centrifugal force on each particle. The net centrifugal force on the system will be Mw2r = Mv2/r, where M is the total mass of the system. This force will act through the centre of mass. Since the system is at rest in this frame, no other pseudo force is needed.
Figure in shows the forces. The cycle is bent at an angle q with the vertical. The forces are
(i) weight Mg,
(ii) normal force N
(iii) friction f and
(iv) centrifugal force 
In the frame considered, the system is at rest. Thus, the total external force and the total external torque must be zero. Let us consider the torques of all the forces about the point A. The torques of N and f about A are zero because these forces pass through A. The torque of Mg about A is Mg(AD) in the clockwise direction and that of  is
is  in the anticlockwise direction. For rotational equilibrium,
in the anticlockwise direction. For rotational equilibrium,
Mg(AD) = 
or, 
or, tanq = ...(10.9)
Thus, the cyclist bends at an angle  with the vertical.
with the vertical.
T.3 A car driver going at a speed of v suddenly finds a wide wall at a distance r. Should he apply breaks or turn the car in a circle of radius r to avoid hitting the wall ?
10. Effect of Earths Rotation on Apparent weight :
The earth rotates about its axis at an angular speed of one revolution per 24 hours. The line joining the north and the south poles is the axis of rotation.
Every point on the earth moves in a circle. A point at equator moves in a circle of radius equal to the radius of the earth and the centre of the circle is same as the centre of the earth. For any other point on the earth, the circle of rotation is smaller than this. Consider a place point on the earth (figure.)
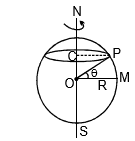
Drop a perpendicular PC from P to the axis SN. The place P rotates in a circle with the centre at C. The radius of this circle is CP. The angle between the line OM and the radius OP through P is called the latitude of the place point. We have CP = OP cosq or, r = R cosq where R is the radius of the earth.
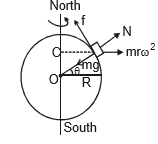
If we calculate work from the frame of reference of the earth, we shall have to assume the existence of pseudo force. In Particular, a centrifugal force mw2r has to be assumed on any particle of mass m placed at P. If we consider a block of mass m at point P then this block is at rest with respect to earth. If resolve the forces along and perpendicular to the line joining the centre of earth then
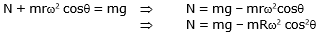
Ex.18 A body weighs 98N on a spring balance at the north pole. What will be its weight recorded on the same scale if it is shifted to the equator ? Use g = GM/R2 = 9.8 m/s2 and the radius of the earth R = 6400 km.
Sol. At poles, the apparent weight is same as the true weight.
Thus, 98N = mg = m(9.8 m/s2)
At the equator, the apparent weight is
mg' = mg -mw2R
The radius of the earth is 6400 km and the angular speed is
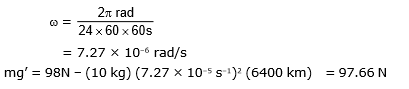
================================================
Solved Examples - Work, Energy and Power, Class 11, Physics
Some solved Examples
Ex.1 Two blocks each of mass M are connected to the ends of a light frame as shown in figure. The frame is rotated about the vertical line of symetry. The rod breaks if the tension in it exceeds T0. Find the maximum frequency with which the frame may be rotated without breaking the rod.
Sol. Consider one of the blocks. If the frequency of revolution is f, the angular velocity is w = 2pf. The acceleration towards the centre is w2l = 4 p2 f2 l . The only horizontal force on the block is the tension of the rod. At the point of breaking, this force is T0. So from Newton's law,
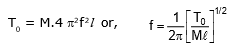
Ex.2 Prove that a motor car moving over a convex bridge is lighter than the same car resting on the same bridge.
Sol. The motion of the motor car over a convex bridge AB is the motion along the segment of a circle AB (figure);

The centripetal force is provided by the difference of weight mg of the car and the normal reaction R of the bridge.

Clearly R < mg, i.e., the weight of the moving car is less than the weight of the stationary car.
Ex.3 A body weighing 0.4 kg is whirled in a vertical circle with a string making 2 revolutions per second. If the radius of the circle is 1.2m. Find the tension (a) at the top of the circle, (b) at the bottom of the circle. Give : g = 10 m s-2 and p = 3.14
Sol. Mass, m = 0.4 kg ;
time period =  second, radius, r = 1.2 m
second, radius, r = 1.2 m
Angular velocity, w =  = 4p rad s-1 = 12.56 rad s-1
= 4p rad s-1 = 12.56 rad s-1
(a) At the top of the circle, T =  -mg
-mg
= mrw2 -mg = m (rw2 -g)
= 0.4 (1.2 × 12.56 × 12.56 -9.8) N = 71.2 N
(b) At the lowest point, T = m(rw2 + g) = 80 N
Ex.4 A metal ring of mass m and radius R is placed on a smooth horizontal table and is set rotating about its own axis in such a way that each part of ring moves with velocity v. Find the tension in the ring.
Sol. Consider a small part ACB of the ring that subtends an angle Dq at the centre as shown in figure.
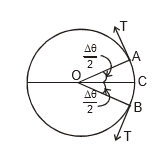
Let the tension in the ring be T.
The forces on this elementary portion ACB are
(i) tension T by the part of the ring left to A
(ii) tension T by the part of the ring to B
(iii) weight (Dm) g
(iv) nomal force N by the table.
As the elementary portion ACB moves in a circle of radius R at constant speed v its acceleration towards centre is
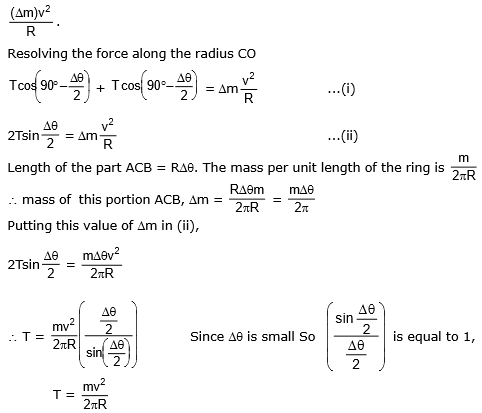
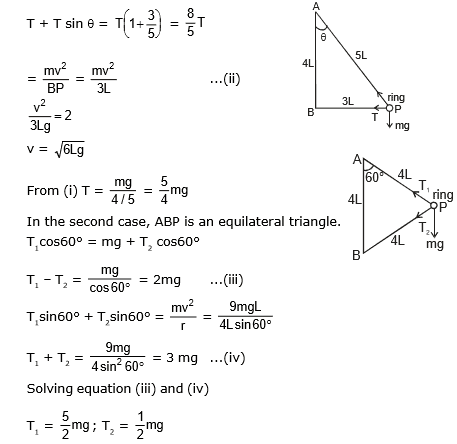
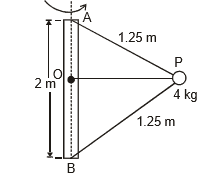
Ex.5 A small smooth ring of mass m is threaded on a light inextensible string of length 8L which has its ends fixed at points in the same vertical line at a distance 4L apart. The ring describes horizontal circles at constant speed with both parts of the string taut and with the lower portion of the string horizontal. Find the speed of the ring and the tension in the string. The ring is then tied at the midpoint of the string and made to perform horizontal circles at constant speed of  . Find the tension in each part of the string.
. Find the tension in each part of the string.
Sol. When the string passes through the ring, the tension in the string is the same in both parts. Also from geometry
BP = 3L and AP = 5L
T cosq =  T = mg ...(i)
T = mg ...(i)
Ex.6 A large mass M and a small mass m hang at the two ends of the string that passes through a smooth tube as shown in Figure. The mass m moves around in a circular path, which lies in the horizontal plane. The length of the string from the mass m to the top of the tube is l and q is the angle this length makes with vertical. What should be the frequency of rotation of mass m so that M remains stationary ?
Sol.
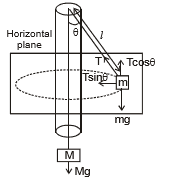
The forces acting on mass m and M are shown in Figure. When mass M is stationary
T = Mg ..(i)
where T is tension in string.
For the smaller mass, the vertical component of tension T cos q balances mg and the horizontal component T sin q supplies the necessary centripetal force.
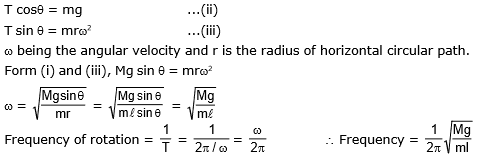
Ex.7 The 4 kg block in the figure is attached to the vertical rod by means of two strings. When the system rotates about the axis of the rod, the two strings are extended as indicated in Figure. How many revolutions per minute must the system make in order that the tension in upper string is 60 N. What is tension in the lower string ?
Sol. The forces acting on block P of mass 4 kg are shown in the Figure.
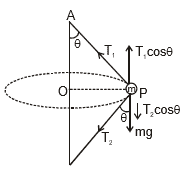
If q is the angle made by strings with vertical, T1 and T2 tensions in strings for equilibrium in the vertical direction
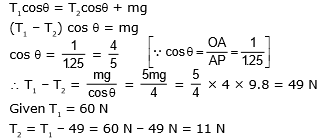
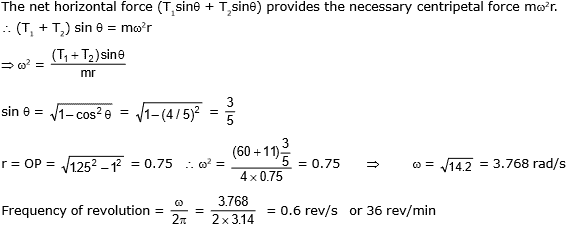
FAQs on Circular Motion, Chapter Notes, Class 11, Physics (IIT-JEE & AIPMT)
| 1. What is circular motion? |  |
| 2. What is the difference between uniform circular motion and non-uniform circular motion? |  |
| 3. What is centripetal force in circular motion? |  |
| 4. How is the frequency of circular motion related to its period? |  |
| 5. What are some real-life examples of circular motion? |  |

|
Explore Courses for Class 11 exam
|

|
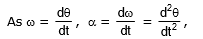 i.e., second derivative of angular displacement w.r.t time gives angular acceleration.
i.e., second derivative of angular displacement w.r.t time gives angular acceleration. also gives the angle of banking for an aircraft, i.e., the angle through which it should tilt while negotiating a curve, to avoid deviation from the circular path.
also gives the angle of banking for an aircraft, i.e., the angle through which it should tilt while negotiating a curve, to avoid deviation from the circular path. 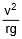 also gives the angle at which a cyclist should lean inward, when rounding a corner. In this case, q is the angle which the cyclist must make with the vertical to negotiate a safe turn.
also gives the angle at which a cyclist should lean inward, when rounding a corner. In this case, q is the angle which the cyclist must make with the vertical to negotiate a safe turn.

















