Class 10 Maths Previous Year Questions - Circles- 2
Previous Year Questions 2017
Q15: The difference between the radii of the smaller circle and the larger circle is 7 cm and the difference between the areas of the two circles is 1078 sq. cm. Find the radius of the smaller circle. [CBSE Delhi 2017 (C)] View Answer
View AnswerAns: Given: r2 - r1 = 7 (r2 > r1) ...(i)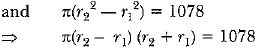
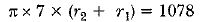 (From equation (i))
(From equation (i))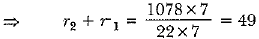 ..... (ii)
..... (ii)
Adding (i) and (ii), we get
2r2 = 56
⇒ r2 = 28 cm
Also, r1 = 21 cm (From equation (i))
∴ Radius of simaller circle = 21 cm.
Q16: Prove that the tangent drawn at any point of a circle is perpendicular to the radius through the point of contact. [AI (C) 2017]
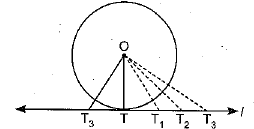
 View Answer
View AnswerAns: Given: A line T tangent to the circle at point T and O is the centre of circle.
To prove: OT ⊥ l
Construction: Take point T1,T2 and T3 on line l and join OT1, OT2, OT3
Proof: We observe that points T1, T2, T3 lie outside the circle, whereas point T lies on the circle.
Hence OT1 > OT
OT2 > OT
OT3 > OT
All distances OT1, OT2, OT3 are greater than OT.
Only OT is the shortest distance.
Also OT = r
Hence r is the shortest distance from tangent l of the circle to the centre as we know that shortest distance between the point on line is perpendicular distance
So, OT ⊥ l
 View Answer
View Answer
Q17: In Fig., PQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents drawn at P and Q intersect at T. Find the length of TP. [AI (C) 2017, Foreign 2015]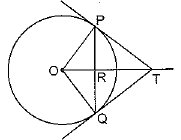 Ans: Given: PQ is a chord of length 8 cm
Ans: Given: PQ is a chord of length 8 cm
Radius OP = 5 cm. PT and QT are tangents to the circle.
To find: TP
OT is perpendicular bisector of PQ
∠ORP = 90°
(Line joining the centre of circle to the common point of two tangents drawn to circle is perpendicular bisector of line joining the point of contact of the tangents.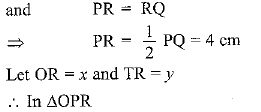
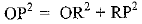
⇒ 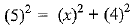
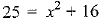
x2 = 16
⇒ x = 3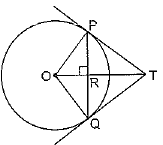
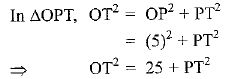
(OR + RT)2 = 25 + PT2
(3 + y)2 = 25 + PT2
9 + y2 + 6y = 25 + PT2 .......(i)
In ΔPRT, TP2 = PR2 + RT2
⇒ PT2 = (4)2 + (y)2 .......(ii)
Put value of PT2 in eq (i)
9 + y2 + 6y = 25 + 16 + y2
6y = 25 + 16 - 9
6y = 32
y = 32/6 = 16/3 cm
Putting y = 16/3 cm in eq (ii), we get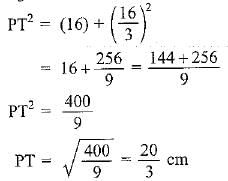
Previous Year Questions 2016
Q18: If from an external point P of a circle with centre 0, two tangents PQ and PR are drawn such that QPR = 120°, prove that 2PQ = PO. [CBSE Delhi (F) 2016]
 View Answer
View AnswerAns: Given, ∠QPR = 120°
Radius is perpendicular to the tangent at the point of contact.
∠OQP = 90° ⇒ ∠QPO = 60°
(Tangents drawn to a circle from an external point are equally inclined to the segment, joining the centre to that point.)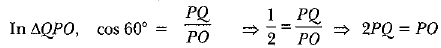
Q19: In Fig. 8.42, AP and BP are tangents to a circle with centre O, such that AP = 5 cm and ∠APS = 60°. Find the length of chord AB. [CBSE Delhi 2016]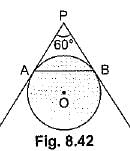
 View Answer
View AnswerAns: PA = PB (Tangents from an external point are equal)
and ∠APB = 60°
⇒ ∠PAB = ∠PBA = 60°
∴ ΔPAB is an equilateral triangle.
Hence AB = PA = 5 cm.
Q20: In Fig. 8.43 from an external point P, two tangents PT and PS are drawn to a circle with centre O and radius r. If OP = 2r, show that ∠OTS = ∠OST = 30°. [CBSE (AI) 2016]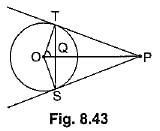
 View Answer
View AnswerAns: Let ∠TOP = θ
∴ 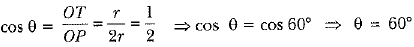
Hence, ∠TOS = 120°
In ∠OTS, OT = OS (Radii of circle)
⇒ 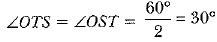
Q21: In Fig. 8.44, are two concentric circles of radii 6 cm and 4 cm with centre O. If AP is a tangent to the larger circle and BP to the smaller circle and length of AP is 8 cm, find the length of BP. [CBSE (F) 2016]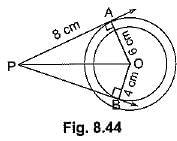
 View Answer
View AnswerAns: OA = 6 cm, OB = 4 cm, AP = 8 cm
OP2 = OA2 + AP2 = 36 + 64 = 100
⇒ OP = 10 cm
BP2 = OP2 - OB2 = 100 - 16 = 84
⇒ 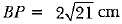
Q22: From an external point P, tangents PA and PR are drawn to a circle with centre O. If ∠PAB = 50°, then find ∠AOB. [CBSE Delhi 2016]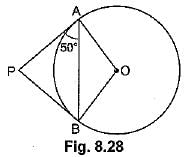
 View Answer
View AnswerAns: ∵ PA = PB ⇒ ∠BAP = ∠ABP = 50°
∴ ∠APB = 180° - 50° - 50° = 80°
and ∠AOB = 180° - 80° = 100°
Q23: In Fig. 8.29, PQ is a tangent at a point C to a circle with centre O. If AB is a diameter and ∠CAB = 30°, find ∠PCA. [CBSE (AI) 2016]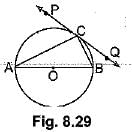
 View Answer
View AnswerAns: ∠ACB = 90° (Angle in the semicircle)
∠CAB = 30° (given)
In ΔABC,
90° + 30° + ∠ABC = 180°
⇒ ΔABC = 60°
Now, ∠PCA = ∠ABC (Angles in the alternate segment)
∴ ∠PCA = 60°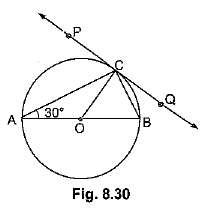
OR
Construction: Jo in 0 to C.
∠PCO = 90° (∵ Line joining centre to point of contact is perpendicular to PQ)
In ΔAOC, OA = OC (Radii of circle)
∴ ∠OAC = ∠OCA = 30° (Equal sides have equal opp. angles)
Now, ∠PCA = ∠PCO - ∠ACO
= 90° - 30° = 60°
Q24: Two tangents PA and PB are drawn to the circle with centre O, such that ∠APB = 120°. Prove that OP = 2AP. (Foreign 2016)
 View Answer
View AnswerAns: Given. A circle C(0, r). PA and PB are tangents to the circle from point P, outside the circle such that ∠APB = 120°. OP is joined.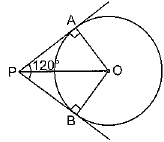
To Prove. OP = 2AP.
Construction. Join OA and OB.
Proof. Consider Δs PAO and PBO
PA = PB [Tangents to a circle, from a point outside it, are equal.]
OP = OP [Common]
∠OAP = ∠OBP = 90°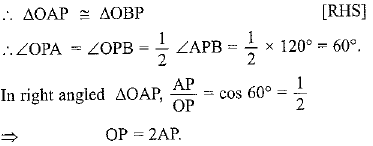
Q25: In Fig. 8.62, two equal circles, with centres O and O', touch each other at X.OO' produced meets the circle with centre O' at A. AC is tangent to the circle with centre O, at the point C. O'D is perpendicular to AC. Find the value of  [CBSE (AI) 2016]
[CBSE (AI) 2016]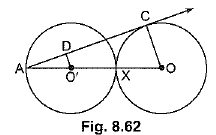
 View Answer
View AnswerAns: AC is tangent to circle with centre O.
Thus ∠ACO = 90°
In ΔAO'D and ΔAOC
∠ADO' = ∠ACO = 90º
∠A = ∠A (Common)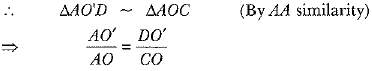
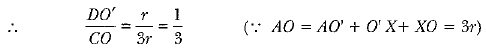
Q26: In Fig. 8.63, O is the centre of a circle of radius 5 cm. T is a point such that OT = 13 cm and OT intersects circle at E. If AB is a tangent to the circle at E, find the length of AB, where TP and TQ are two tangents to the circle. [CBSE Delhi 2016]
 View Answer
View AnswerAns: 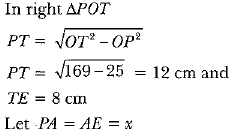
(Tangents from an external point to a circle are equal)
In right ΔAET.
TA2 = TE2 + EA2
⇒ (12 - x)2 = 64 + x2 ⇒ 144 + x2 - 24x = 64 + x2
⇒ x = 80/24 ⇒ x = 3.3 cm
Thus, AB = 6.6 cm
Previous Year Questions 2014
Q27: Prove that the parallelogram circumscribing a circle is a rhombus. [CBSE Delhi 2014; CBSE 2019 (30/5/1)]
 View Answer
View AnswerAns: Let ABCD be a parallelogram such that its sides touch a circle with centre O.
We know that the tangents to a circle from an exterior point are equal in length.
Therefore, we have
AP = AS (Tangents from A) ... (i)
BP = BQ (Tangents from B) ... (ii)
CR = CQ (Tangents from C) ... (iii)
And DR = DS (Tangents from D) ... (iv)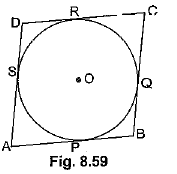
Adding (i), (ii), (iii) and (iv), we have
(AP + BP) + (CR + DR) = (AS + DS) + (BQ + CQ)
AB + CD = AD + BC
AB + AB = BC + BC (∵ ABCD is a parallelogram ∴ AB = CD, BC = DA)
2AB = 2BC ⇒ AB = BC
Thus, AB = BC = CD = AD
Hence, ABCD is a rhombus.
Q28: Prove that the lengths of two tangents drawn from an external point to a circle are equal. [CBSE, Delhi 2014, (F) 2014, Delhi 2016, (AI) 2016, (F) 2016, CBSE Delhi 2017, (AI) 2017, (F) 2017, Delhi 2017 (C)]
 View Answer
View AnswerAns: Given: AP and AQ are two tangents from a pointed to a circle C (O, r).
To Prove: AP = AQ
Construction: Join OP, OQ and OA.
Proof: In order to prove that AP = AQ, we shall first prove that ΔOPA ≅ ΔOQA.
Since a tangent at any point of a circle is perpendicular to the radius through the point of contact.
∴ OP ⊥ AP and OQ ⊥ AQ
⇒ ∠OPA = ∠OQA = 90° ........(i)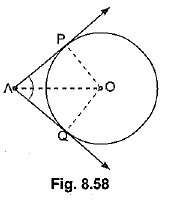
Now, in right triangles OPA and OQA, we have
OP = OQ (Radii of a circle)
∠OPA = ∠OQA (Each 90°)
and OA = OA (Common)
So, by RHS-criterion of congruence, we get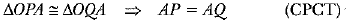
Hence, lengths of two tangents from an external point are equal.
|
63 videos|271 docs|99 tests
|
FAQs on Class 10 Maths Previous Year Questions - Circles- 2
| 1. How do you find the circumference of a circle? |  |
| 2. What is the relationship between the diameter and radius of a circle? |  |
| 3. How do you find the area of a circle? |  |
| 4. What is the relationship between the circumference and diameter of a circle? |  |
| 5. How do you find the radius of a circle if you only know the circumference? |  |

|
Explore Courses for Grade 10 exam
|

|

















