Class 9 Maths Chapter 6 Practice Question Answers - Triangles
Q1: ABC is an isosceles triangle with AB = AC and D is a point on BC such that AD⊥BC (Figure). To prove that ∠BAD =∠CAD, a student proceeded as follows:
ΔABD and ΔACD,
AB = AC (Given)
∠B =∠C (because AB = AC)
and ∠ADB =∠ADC
Therefore, ΔABD ≅ ΔACD(AAS)
So, ∠BAD =∠CAD(CPCT)
What is the defect in the above arguments?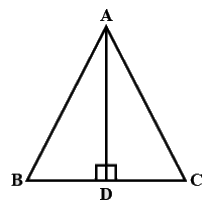 (a) It is defective to use ∠ABD = ∠ACD for proving this result.
(a) It is defective to use ∠ABD = ∠ACD for proving this result.
(b) It is defective to use ∠ADB = ∠ADC for proving this result.
(c) It is defective to use ∠ADB = ∠ADC for proving this result.
(d) Cannot be determined
Ans: △ABD and △ACD
AB=AC (given )
Then ∠ABD =∠ACD ( because AB = AC)
and ∠ADB = ∠ADC=90( because AD⊥BC)
∴△ABD = △ACD
∠BAD = ∠CAD
It is defective to use ∠ABD=∠ACD for proving this result
Q2: Given ΔOAP ≅ ΔOBP in figure, the criteria by which the triangles are congruent is: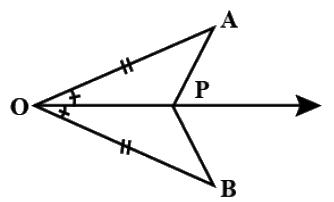 (a) SAS
(a) SAS
(b) SSS
(c) RHS
(d) ASA
Ans: (a)
In △OAP and △OBP
OA=OB (given)
∠AOP=∠BOP (given)
OP=OP (common side)
∴△OAP≅△OBP by SAS congruent rule as two corresponding sides and the included angles of the triangles are equal.
Q3: In ΔABC, if ∠A=50∘ and ∠B=60∘ , then the greatest side is :
(a) AB
(b) BC
(c) AC
(d) Cannot say
Ans: (a)
Using angle sum property of triangle
∠A+∠B+∠C = 180º
⇒ 50º + 60º +∠C = 180º
⇒ ∠C = 180º −110º = 70º
Side opposite to the largest angle is the longest side. Here ∠C is largest and side opposite to it is AB So AB is the longest side.
Q4: The construction of a triangle ABC, given that BC = 3 cm is possible when difference of AB and AC is equal to :
(a) 3.2 cm
(b) 3.1 cm
(c) 3 cm
(d) 2.8 cm
Ans: (d)
Let the length of AB be x and AC be y
A triangle can be formed if the sum of any two sides is greater then the third
⇒BC+AC>AB
⇒3+AC>AB
⇒3>AB−AC
⇒AB−AC<3
So only option Dis correct.
Q5: In ΔPQR, if ∠R >∠Q, then:
(a) QR>PR
(b) PQ>PR
(c) PQ<PR
(d) QR<PR
Ans: (b)
We know that the side opposite to the greater angle is greater.
Given: ∠R>∠Q
The side opposite to ∠R=PQ and the side opposite to ∠Q=PR.
Thus, PQ>PR.
Hence, option B is correct.
Q6: In ΔABC and ΔDEF, AB = DF and ∠A =∠D. The two triangles will be congruent by SAS axiom if :
(a) BC = EF
(b) AC = DE
(c) BC = DE
(d) AC = EF
Ans: (b)
For triangle to be congruent SAS(Side angle Side) axiom we need to have two equal sides and the included angle same.
In ΔABC and ΔDFE
We have AB=DF
and ∠A=∠D
But ∠A is formed by the two sides AB and AC of ΔABC
But ∠D is formed by the two sides DE and DF of ΔDFE
So we need AC=DE for the two triangles to be congruent.
Q7: In triangles ABC and DEF, AB = FD and ∠A=∠D. The two triangles will be congruent by SAS axiom if:
(a) BC = EF
(b) AC = DE
(c) AC = EF
(d) BC = DE
Ans: (b)
For triangle to be congruent by SAS axiom two of the sides and the included angle of the triangles must be equal.
Given AB=DF and ∠A=∠D
No for △ABC≅△DFE by SAS axiom we need AC=DE
So option B is correct.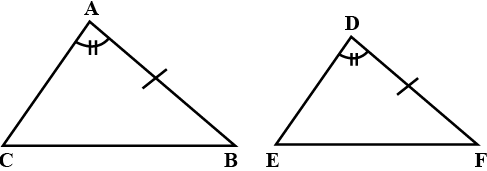
Q8: In ΔABC,∠B = 30º, ∠C = 80º and ∠A = 70º then,
(a) AB > BC < AC
(b) AB < BC > AC
(c) AB > BC > AC
(d) AB < BC < AC
Ans: (c)
In any triangle side opposite to the largest angle is the longest side.
Here ∠C is largest and side opposite to it is AB
∴ AB is the longest side.
Then comes ∠A and side opposite to it is BC.
∴ BC is second longest.
Then comes ∠B and side opposite to it is AC
So it is the smallest side.
So the decreasing order of sides is
AB > BC > AC
Q9: If ΔABC ≅ ΔDEF by SSS congruence rule then :
(a) AB = EF, BC = FD, CA = DE
(b) AB = FD, BC = DE, CA = EF
(c) AB = DE, BC = EF, CA = FD
(d) AB = DE, BC = EF, ∠C = ∠F
Ans: (c)
If triangles are congruent by SSS congruence rule then their corresponding sides are equal.
Here △ABC≅△DEF
∴ AB = DE, BC = EF, AC = DF or (CA=FD)
So option C is correct.
Q10: In the given figure , which of the following statement is true ? (a) ∠B=∠C
(a) ∠B=∠C
(b) ∠B is the greatest angle in triangle
(c) ∠B is the smallest angle in triangle
(d) ∠A is the smallest angle in triangle
Ans: (c)
In any triangle angle opposite to the longest side is largest and smallest side is smallest.
Here BC is the longest side and angle opposite to it is ∠A so ∠A is the largest angle.
Smallest side is AC and angle opposite to it is ∠B so ∠B is the smallest angle.
So option C is correct.
Q11: In ΔPQR, ∠P = 70º and ∠R = 30º . Which side of this triangle is the longest?
(a) PR
(b) QR
(c) PQ
(d) All sides are equal.
Ans: (a)
Given, △PQR, ∠P = 70º, ∠R = 30º
Sum of angles of triangle = 180º
⟹ ∠P + ∠Q + ∠R = 180∘
⟹ 70º + 30º +∠Q = 180º
⟹ ∠Q = 80º
Since ∠Q is the largest ⟹ PR will be the longest side this is because when the two sides of a triangle are unequal, the angle opposite to the longer side is larger.
Q12: If in two triangles ΔABC and ΔPQR, AB = QR,BC = PR and CA = PQ, then :
(a) ΔABC ≅ ΔPQR
(b) ΔCBA ≅ ΔPRQ
(c) ΔBAC ≅ ΔRPQ
(d) ΔPQR ≅ ΔBCA
Ans: (b)
In △ABC and △PQR, we are given
AB=QR,
BC=PR
and CA=PQ
∴ΔCBA≅ΔPRQ
Q13: In ΔABC, ∠A = 100º , ∠B = 30º and ∠C = 50º then
(a) AB>AC
(b) AB<AC
(c) BC<AC
(d) none of these
Ans: (a)
We know that the side opposite to largest angle is longest side of triangle .
∠A is the largest angle and side opposite to it is BC
So BC is the longest side.
Then comes ∠C and side opposite to it is AB.
So AB is second longest side.
Then comes ∠B and side opposite to it is AC.
So it is the smallest side.
So the decreasing order of side is
BC>AB>AC
So option A is correct.
Q14: AB and CD are the smallest and largest sides of a quadrilateral ABCD. Out of ∠B and ∠D, decide which is greater.
(a) ∠B will be greater.
(b) ∠D will be greater.
(c) Both the angles are equal.
(d) Cannot be determined.
Ans: (a)
In the fig. ABCD is a quadrilateral with AB as the smallest side, and CD as the largest side.
We join the diagonal BD.
We label the angles as shown in the figure as θ1 , θ2, θ3, θ4 as shown in figure.
Now in ΔABD,
AB<AD
⟹ θ2 < θ1 → (1)
Similarly in ΔBCD,
BC < CD
⟹ θ4 < θ3 → (2)
Adding (1)and(2),
θ2 + θ4 < θ1 + θ3
which is nothing but,
∠D < ∠B
Thus ∠(B) will be greater.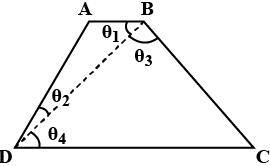
Q15: M is a point on side BC of a triangle ABC such that AM is the bisector of ∠BAC. Is it true to say that perimeter of the triangle is greater than 2 AM? If True enter 1, else if False enter 0.
Ans: Given, △ABC, AM is bisector of ∠BAC
Now, In △ABM,
AB + BM > AM (Sum of two sides of triangle is greater than the third side)
Now, In △ACM,
AC+CM>AM (Sum of two sides of triangle is greater than the third side)
Thus, adding both,
AB + BM + AC + CM > 2AM
AB + AC + BC > 2AM
Q16: In the adjoining figure, AD = BD ∠ABD = 65º & ∠DAC = 22º. Find ∠ACD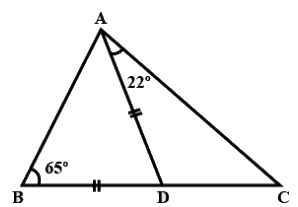 Ans: AD = BD
Ans: AD = BD
∠BAD = ∠ABD = 65
∠BAC = ∠BAD+∠DAC
∠BAC = 65+22
∠BAC = 87
∠A +∠B +∠C = 180°
87 + 65 + ∠C=180°
∠C = 180 − 152
∠C = 28
∴∠ACD = 28
Q17: In given figures sides AB and BC and median AD of a △ABC are respectively proportional to sides PQ,QR and median PM of △PQR. Show that △ABC∼△PQR.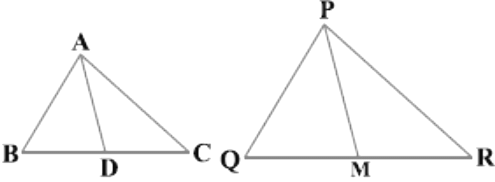 Ans: Given AD and PM are medians of △ABC and △PQR,
Ans: Given AD and PM are medians of △ABC and △PQR,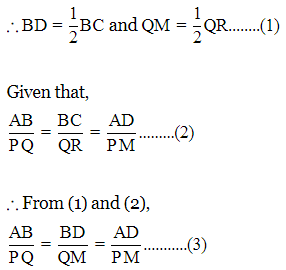
In △ABD and △PQM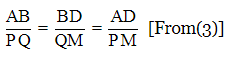
∴ By SSS criterian of proportionality △ABD ∼ △PQM
∴ ∠B = ∠Q (Corresponding Sides of Similar Triangles) .............(4)
In △ABC and △PQR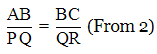
∠B = ∠Q (From 4)
∴ By SAS criterian of proportionality △ABC ∼ △PQR [hence proved]
Q18: In given figure two sides AB,BC and median AM of one triangle ABC are respectively equal to sides PQ and QR and median PN of △PQR. Show that: △ABM ≅ △PQN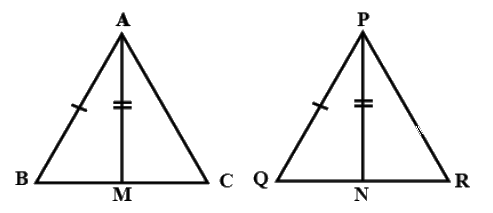 Ans: Given two sides AB,BC and median AM of △ABC are respectively equal to sides PQ and QR and median PN of △PQR.
Ans: Given two sides AB,BC and median AM of △ABC are respectively equal to sides PQ and QR and median PN of △PQR.
BC=QR .. Given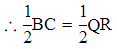
∴ BM = QN ...(1)
In △ABM and △PQN,
AB=PQ ...Given
AM=PN ...Given
BM=QN ...From (1)
∴ΔABM ≅ ΔPQN [hence proved]
Q19: In ΔPQR , If PQ>PR and bisectors of ∠Q and ∠R interested at S. Show that SQ>SR.
Ans: Given that-
ΔPQR, PQ > PR
∠Q & ∠R have bisector intersected at S
To find-
To prove SQ > SR
PQ > PR
∴∠PQR > ∠PRQ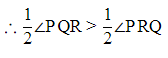
∴∠SRQ >∠SQR [∵ QS and RS bisectors of ∠Q and LR respectively]
⇒ SQ > SR (Since, ∠SRQ is larger and is formed by)
Hence, proved SQ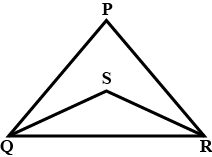
Q20: Find the circumcentre of the triangle with the vertices A (-3,4) , B (3,4) and C (-4,-3). What is the circumradius of the circle?
Ans: We want the equation of circle to pass through points A(−3,4),B(3,4) and (−4,3)
The equation of a circle is x2 + y2 + ax + by + c = 0
From the coordinates of the three given points we get three equations.
⇒ (−3)2 + 42 − 3a + 4b + c = 0
⇒ 32 + 42 − 3a + 4b + c = 0
⇒ (−4)2 + 32 − 4a + 3b + c = 0
we can simplify the above equation -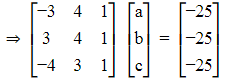
solving the above matrix equation using craner's rule a=0, b=0 and c=−25
Putting there values a,b and c in general form we write required equation of circle x2 + y2 − 25 = 0
On center radius form (x+0)2 + (y + 0)2 = 25
Center of circle is (0,0) and radius =5.
Hence, the answer is 5.
|
40 videos|471 docs|57 tests
|
















