Class 10 Exam > Class 10 Notes > Mathematics for GCSE/IGCSE > Compound Interest
Compound Interest | Mathematics for GCSE/IGCSE - Class 10 PDF Download
Compound Interest
- Compound interest is when interest is paid on both the original amount and the interest accumulated from previous periods.
- This contrasts with simple interest, where interest is only calculated on the original amount.
- Simple interest increases by the same amount each period, while compound interest increases by a progressively larger amount each period.
How do you work with compound interest?
- Keep multiplying by the decimal equivalent of the percentage you want (the multiplier, p)
- A 25% increase (p = 1 + 0.25) each year for 3 years is the same as multiplying by 1.25 × 1.25 × 1.25
- Using powers, this is the same as × 1.253
- In general, the multiplier p applied n times gives an overall multiplier of pn
- If the percentages change varies from year to year, multiply by each one in order
- a 5% increase one year followed by a 45% increase the next year is 1.05 × 1.45
- In general, the multiplier p1 followed by the multiplier p2 followed by the multiplier p3... etc gives an overall multiplier of p1p2p3...
Compound interest formula
- Alternative method: A formula for the final ("after") amount is
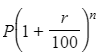 where...
where... - ...P is the original ("before") amount, r is the % increase, and n is the number of years
- Note that
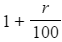 is the same value as the multiplier
is the same value as the multiplier
Depreciation
- Depreciation is the process by which an item loses value over time.
- Examples include cars, game consoles, and other electronics.
- Depreciation is typically calculated as a percentage decrease at the end of each year.
- This calculation works similarly to compound interest, but it involves a percentage decrease instead of an increase.
How do I calculate a depreciation?
- You would calculate the new value after depreciation using the same method as compound interest
- Identify the multiplier, p (1 - "% as a decimal")
- 10% depreciation would have a multiplier of p = 1 - 0.1 = 0.9
- 1% depreciation would have a multiplier of p = 1 - 0.01 = 0.99
- Raise the multiplier to the power of the number of years (or months etc) pn
- Multiply by the starting value
- Identify the multiplier, p (1 - "% as a decimal")
- New value is A x pn
- A is the starting value
- p is the multiplier for the depreciation
- n is the number of years
- Alternative method: A formula for the final ("after") amount is
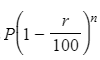 where...
where... - ...P is the original ("before") amount, r is the % decrease, and n is the number of years
- If you are asked to find the amount the value has depreciated by:
- Find the difference between the starting value and the new value
The document Compound Interest | Mathematics for GCSE/IGCSE - Class 10 is a part of the Class 10 Course Mathematics for GCSE/IGCSE.
All you need of Class 10 at this link: Class 10
|
38 videos|395 docs|19 tests
|
Related Searches















