Connectivity | Engineering Mathematics - Engineering Mathematics PDF Download
Introduction
A graph is said to be connected if there is a path between every pair of vertex. From every vertex to any other vertex, there should be some path to traverse. That is called the connectivity of a graph. A graph with multiple disconnected vertices and edges is said to be disconnected.
Example 1: In the following graph, it is possible to travel from one vertex to any other vertex. For example, one can traverse from vertex ‘a’ to vertex ‘e’ using the path ‘a-b-e’.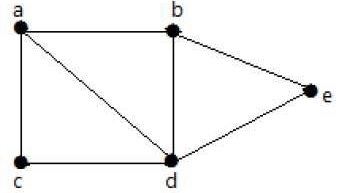
Example 2: In the following example, traversing from vertex ‘a’ to vertex ‘f’ is not possible because there is no path between them directly or indirectly. Hence it is a disconnected graph.
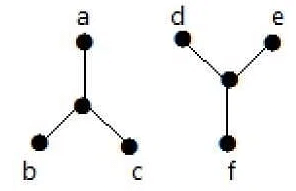
Cut Vertex
Let ‘G’ be a connected graph. A vertex V ∈ G is called a cut vertex of ‘G’, if ‘G-V’ (Delete ‘V’ from ‘G’) results in a disconnected graph. Removing a cut vertex from a graph breaks it in to two or more graphs.
Note: Removing a cut vertex may render a graph disconnected.
A connected graph ‘G’ may have at most (n–2) cut vertices.
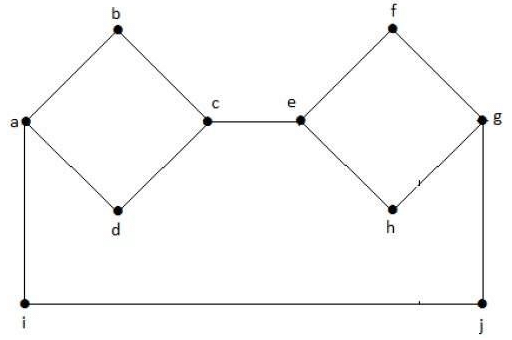
Example: In the following graph, vertices ‘e’ and ‘c’ are the cut vertices.
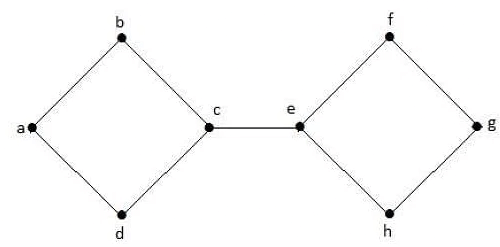
By removing ‘e’ or ‘c’, the graph will become a disconnected graph.
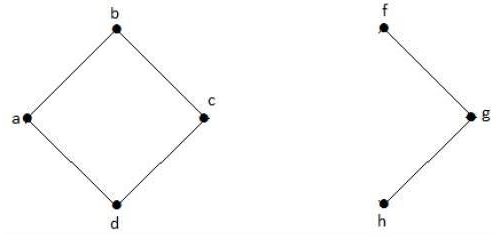
Without ‘g’, there is no path between vertex ‘c’ and vertex ‘h’ and many other. Hence it is a disconnected graph with cut vertex as ‘e’. Similarly, ‘c’ is also a cut vertex for the above graph.
Cut Edge (Bridge)
- Let ‘G’ be a connected graph. An edge ‘e’ ∈ G is called a cut edge if ‘G-e’ results in a disconnected graph.
- If removing an edge in a graph results in to two or more graphs, then that edge is called a Cut Edge.
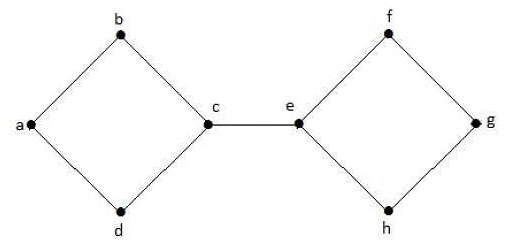
Example: In the following graph, the cut edge is [(c, e)].
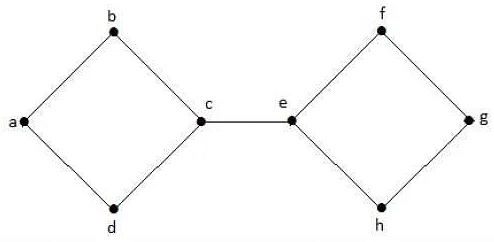
By removing the edge (c, e) from the graph, it becomes a disconnected graph.
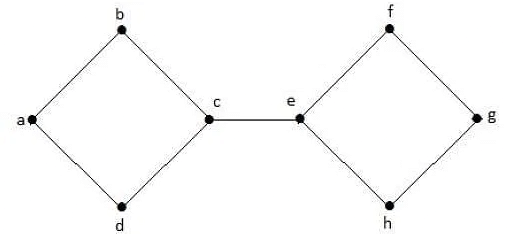
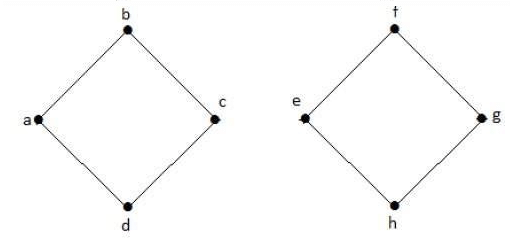
In the above graph, removing the edge (c, e) breaks the graph into two which is nothing but a disconnected graph. Hence, the edge (c, e) is a cut edge of the graph.
Note − Let ‘G’ be a connected graph with ‘n’ vertices, then
- a cut edge e ∈ G if and only if the edge ‘e’ is not a part of any cycle in G.
- the maximum number of cut edges possible is ‘n-1’.
- whenever cut edges exist, cut vertices also exist because at least one vertex of a cut edge is a cut vertex.
- if a cut vertex exists, then a cut edge may or may not exist.
Cut Set of a Graph
- Let ‘G’= (V, E) be a connected graph. A subset E’ of E is called a cut set of G if deletion of all the edges of E’ from G makes G disconnect.
- If deleting a certain number of edges from a graph makes it disconnected, then those deleted edges are called the cut set of the graph.
Example: Take a look at the following graph. Its cut set is E1 = {e1, e3, e5, e8}.
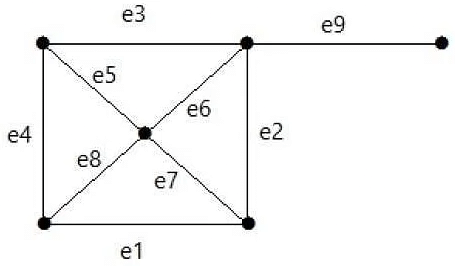
After removing the cut set E1 from the graph, it would appear as follows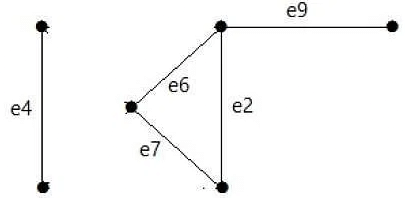
Similarly, there are other cut sets that can disconnect the graph
- E3 = {e9} – Smallest cut set of the graph.
- E4 = {e3, e4, e5}
Edge Connectivity
Let ‘G’ be a connected graph. The minimum number of edges whose removal makes ‘G’ disconnected is called edge connectivity of G.
Notation − λ(G)
In other words, the number of edges in a smallest cut set of G is called the edge connectivity of G.
If ‘G’ has a cut edge, then λ(G) is 1. (edge connectivity of G.)
Example: Take a look at the following graph. By removing two minimum edges, the connected graph becomes disconnected. Hence, its edge connectivity (λ(G)) is 2.
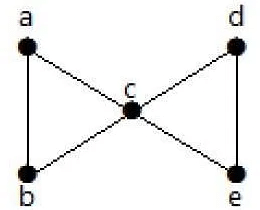
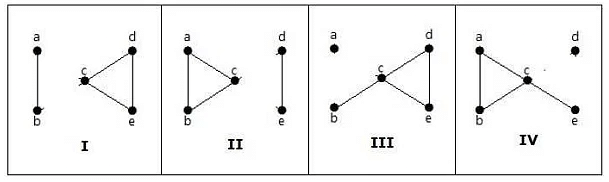
Here are the four ways to disconnect the graph by removing two edges −
Vertex Connectivity
Let ‘G’ be a connected graph. The minimum number of vertices whose removal makes ‘G’ either disconnected or reduces ‘G’ in to a trivial graph is called its vertex connectivity.
Notation − K(G)
Example: In the above graph, removing the vertices ‘e’ and ‘i’ makes the graph disconnected.
If G has a cut vertex, then K(G) = 1.
Notation − For any connected graph G,
K(G) ≤ λ(G) ≤ δ(G)
Vertex connectivity (K(G)), edge connectivity (λ(G)), minimum number of degrees of G(δ(G)).
Example: Calculate λ(G) and K(G) for the following graph
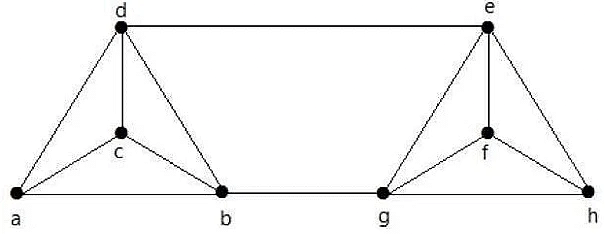
From the graph,
δ(G) = 3
K(G) ≤ λ(G) ≤ δ(G) = 3 (1)
K(G) ≥ 2 (2)
Deleting the edges {d, e} and {b, h}, we can disconnect G.
Therefore,
λ(G) = 2
2 ≤ λ(G) ≤ δ(G) = 2 (3)
From (2) and (3), vertex connectivity K(G) = 2
|
65 videos|129 docs|94 tests
|
















